题目
Chessboard
| Time Limit: 2000MS | Memory Limit: 65536K | |
| Total Submissions: 20511 | Accepted: 6427 |
Description
Alice and Bob often play games on chessboard. One day, Alice draws a board with size M * N. She wants Bob to use a lot of cards with size 1 * 2 to cover the board. However, she thinks it too easy to bob, so she makes some holes on the board (as shown in the figure below).
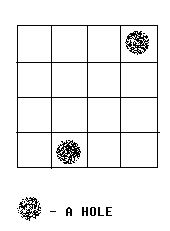
We call a grid, which doesn’t contain a hole, a normal grid. Bob has to follow the rules below:
1. Any normal grid should be covered with exactly one card.
2. One card should cover exactly 2 normal adjacent grids.
Some examples are given in the figures below:

A VALID solution.
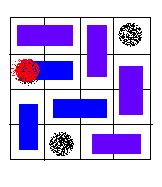
An invalid solution, because the hole of red color is covered with a card.
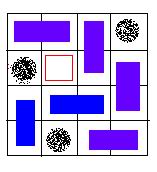
An invalid solution, because there exists a grid, which is not covered.
Your task is to help Bob to decide whether or not the chessboard can be covered according to the rules above.

We call a grid, which doesn’t contain a hole, a normal grid. Bob has to follow the rules below:
1. Any normal grid should be covered with exactly one card.
2. One card should cover exactly 2 normal adjacent grids.
Some examples are given in the figures below:

A VALID solution.

An invalid solution, because the hole of red color is covered with a card.

An invalid solution, because there exists a grid, which is not covered.
Your task is to help Bob to decide whether or not the chessboard can be covered according to the rules above.
Input
There are 3 integers in the first line: m, n, k (0 < m, n <= 32, 0 <= K < m * n), the number of rows, column and holes. In the next k lines, there is a pair of integers (x, y) in each line, which represents a hole in the y-th row, the x-th column.
Output
If the board can be covered, output "YES". Otherwise, output "NO".
Sample Input
4 3 2 2 1 3 3
Sample Output
YES
大意
一个棋盘内,有些地方有洞,有些地方没有,没有洞的地方可以放东西,求是否可以用1*2的长方形填满所有格子(除了洞)。当然长方形不能相互覆盖。
分析
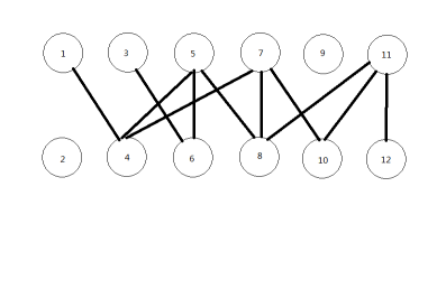
显然,每一块矩形相当于把相邻的两个点匹配起来。 根据前面题目的启发,应该可以相当实用黑白染色,点的编号类似。然后依然是黑点连白点。 如果相邻的点不是洞,那么就连一条边。显然这是一个二分图。 然后跑这个二分图最大匹配。假设有k对匹配对,洞的数目为c,说明有2*k个点匹配成功。 如果满足k*2+c=n*m,那么有解。 连边后如图,其中1-4,3-6,5-8,7-10,11-12即为一组大小为5的最大匹配。
代码
1 #include<cstdio> 2 #include<cstring> 3 #include<iostream> 4 #include<vector> 5 using namespace std; 6 int n,m,x,y,ans,link[4005],s[2050][2050],ss; 7 vector<int> f[4005]; 8 bool cover[4005],a[2050][2050]; 9 int fx[4][2]={{1,0},{0,1},{0,-1},{-1,0}};//4个方向 10 bool find(int i) 11 { 12 for (int k=0;k<f[i].size();k++) 13 if (!cover[f[i][k]]) 14 { 15 int j=f[i][k]; 16 cover[j]=true; 17 int q=link[j]; 18 link[j]=i; 19 if (q==0||find(q)) return true; 20 link[j]=q; 21 } 22 return false; 23 } 24 int main() 25 { 26 cin>>n>>m>>ss; 27 for(int i=1;i<=n;i++) 28 for(int j=1;j<=m;j++) 29 s[i][j]=m*(i-1)+j; 30 for (int i=1;i<=ss;i++) 31 { 32 cin>>x>>y; 33 a[y][x]=1; //attention!!!!! 34 } 35 for (int i=1;i<=n;i++) 36 { 37 for (int j=1;j<=m;j++) 38 { 39 if (!a[i][j]) 40 { 41 int ax,ay; 42 for(int kk=0;kk<4;kk++)//4个方向 43 { 44 ax=i+fx[kk][0],ay=j+fx[kk][1]; 45 if(ax<1||ax>n||ay<1||ay>m) continue;//判断是否越界 46 if(a[ax][ay]) continue;//判断该可攻击的点是否被移除 47 f[s[i][j]].push_back(s[ax][ay]);//连边 48 } 49 } 50 51 } 52 } 53 int ans=0; 54 for (int i=1;i<=n*m;i++) 55 { 56 memset(cover,0,sizeof(cover)); 57 ans+=find(i); 58 } 59 if (2*ans+ss==n*m) cout<<"YES"; 60 else cout<<"NO"; 61 }