【Tarjan】洛谷P3379 Tarjan求LCA

题目描述

如题，给定一棵有根多叉树，请求出指定两个点直接最近的公共祖先。

输入输出格式

输入格式：

第一行包含三个正整数N、M、S，分别表示树的结点个数、询问的个数和树根结点的序号。

接下来N-1行每行包含两个正整数x、y，表示x结点和y结点之间有一条直接连接的边（数据保证可以构成树）。

接下来M行每行包含两个正整数a、b，表示询问a结点和b结点的最近公共祖先。

输出格式：

输出包含M行，每行包含一个正整数，依次为每一个询问的结果。

输入输出样例

输入样例#1：

5 5 4
3 1
2 4
5 1
1 4
2 4
3 2
3 5
1 2
4 5

输出样例#1：

4
4
1
4
4

说明

时空限制：1000ms,128M

数据规模：

对于30%的数据：N<=10，M<=10

对于70%的数据：N<=10000，M<=10000

对于100%的数据：N<=500000，M<=500000

样例说明：

该树结构如下：

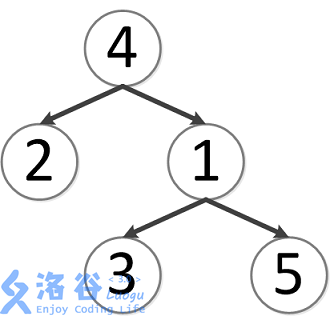
第一次询问：2、4的最近公共祖先，故为4。

第二次询问：3、2的最近公共祖先，故为4。

第三次询问：3、5的最近公共祖先，故为1。

第四次询问：1、2的最近公共祖先，故为4。

第五次询问：4、5的最近公共祖先，故为4。

故输出依次为4、4、1、4、4。

题解

Tarjan求LCA

就是正常的dfs标记一下是否遍历过再加上并查集把子节点往上合并

然后对于每个询问的x判断y是否遍历过

如果是，则标记LCA（x，y）=find（y）；

不是的话继续遍历【显然最后肯定都能求出】

代码

//by 减维
#include<cstdio>
#include<iostream>
#include<cstring>
#include<queue>
#include<cstdlib>
#include<ctime>
#include<cmath>
#include<algorithm>
#define ll long long
using namespace std;

struct edge{
    int to,ne;
}e[1000005];

struct edgge{
    int to,ne,lca;
}ed[1000005];

int n,m,s,ecnt,cnt,head[500005],hea[500005],f[500005];
bool vis[500005];

int find(int x)
{
    if(x==f[x])return x;
    f[x]=find(f[x]);
    return f[x];
}

void add(int x,int y)
{
    e[++ecnt].to=y;
    e[ecnt].ne=head[x];
    head[x]=ecnt;
}

void ad(int x,int y)
{
    ed[++cnt].to=y;
    ed[cnt].ne=hea[x];
    hea[x]=cnt;
}

void dfs(int x)
{
    vis[x]=1;
    for(int i=head[x];i;i=e[i].ne)
    {
        int dd=e[i].to;
        if(vis[dd])continue;
        dfs(dd);
        f[find(dd)]=find(x);
    }
    for(int i=hea[x];i;i=ed[i].ne)
    {
        int dd=ed[i].to;
        if(vis[dd]){
            ed[i].lca=find(dd);
        }
    }
}

int main()
{
    scanf("%d%d%d",&n,&m,&s);
    int x,y;
    for(int i=1;i<n;++i)
    {
        scanf("%d%d",&x,&y);
        add(x,y);
        add(y,x);
    }
    for(int i=1;i<=m;++i)
    {
        scanf("%d%d",&x,&y);
        ad(x,y);
        ad(y,x);
    }
    for(int i=1;i<=n;++i)f[i]=i;
    dfs(s);
    for(int i=1;i<=cnt;i+=2)
        printf("%d
",ed[i].lca==0?ed[i+1].lca:ed[i].lca);
}