二叉查找树(Binary Search Tree),(又:二叉搜索树,二叉排序树)它或者是一棵空树,或者是具有下列性质的二叉树: 若它的左子树不空,则左子树上所有结点的值均小于它的根结点的值; 若它的右子树不空,则右子树上所有结点的值均大于它的根结点的值; 它的左、右子树也分别为二叉排序树。
以前只是知道又这么一种树但是没怎么去了解,这次查看了算法导论上介绍的思路, 用php写了个例子。
节点类

BST树类
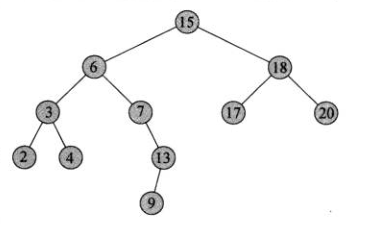
二叉搜索树样图
下面介绍下大致的操作
一 遍历
二叉搜索树可以通过简单的递归来遍历所有节点的关键词, 根据根,以及左右子树的输出顺序分为3种
(左根右) 中序遍历 [2 3 4 6 7 9 13 15 17 18 20]
(根左右) 先序遍历 [15 6 3 2 4 7 13 9 18 17 20]
(左右根) 后序遍历 [2 4 3 9 13 7 6 17 20 18 15]
中序遍历 示例
/** * 遍历节点,获取key数组 * @param Node $node 节点 * @param int $type 遍历类型 0 中序 1 前序 2 后序 * @return array * @author zxqc2018 */ public function walkTree(Node $node, int $type = 0) { $keyArr = []; $walkTreeFunc = function (?Node $node) use (&$keyArr, &$walkTreeFunc, $type){ if (!is_null($node)) { if ($type === 1) { $keyArr[] = $node->getKey(); $walkTreeFunc($node->getLeft()); $walkTreeFunc($node->getRight()); } else if ($type == 2) { $walkTreeFunc($node->getLeft()); $walkTreeFunc($node->getRight()); $keyArr[] = $node->getKey(); } else { $walkTreeFunc($node->getLeft()); $keyArr[] = $node->getKey(); $walkTreeFunc($node->getRight()); } } }; $walkTreeFunc($node); return $keyArr; }
二 查找节点
非递归查找
/** * 根据key, 查找节点 * @param int $key * @param Node|null $node * @return Node|null * @author zxqc2018 */ public function search(int $key, Node $node = null) { if (is_null($node)) { $node = $this->getRoot(); } while (!is_null($node) && $key != $node->getKey()) { if ($key < $node->getKey()) { $node = $node->getLeft(); } else { $node = $node->getRight(); } } return $node; }
递归查找
/** * 根据key, 查找节点 * @param int $key * @param Node|null $node * @return mixed * @author zxqc2018 */ public function searchRecursion(int $key, Node $node = null) { if (is_null($node)) { $node = $this->getRoot(); } $recursionFunc = function ($key, Node $node) use (&$recursionFunc) { if (is_null($node) || $node->getKey() == $key) { return $node; } if ($key < $node->getKey()) { return $recursionFunc($key, $node->getLeft()); } else { return $recursionFunc($key, $node->getRight()); } }; return $recursionFunc($key, $node); }
三 查找最大或小节点
最小节点
/** * 查找最小节点 * @param Node|null $node * @return Node|null * @author zxqc2018 */ public function findMinNode(Node $node) { if (!is_null($node)) { while (!is_null($node->getLeft())) { $node = $node->getLeft(); } } return $node; }
最大节点
/** * 查找最大节点 * @param Node|null $node * @return Node|null * @author zxqc2018 */ public function findMaxNode(Node $node) { if (!is_null($node) && !is_null($node->getRight())) { $node = $this->findMaxNode($node->getRight()); } return $node; }
四 后继和前驱
一颗二叉搜索树,按照中序遍历(从小到大)后的次序, 给定某个节点, 那么 后继 则是 此节点之后的那个节点, 前驱 则反之
查找后继有两种情况
1 节点的右孩子非空, 则后继是 右节点为根的子树种 关键字 最小的节点 。
2 节点的右孩子是空 并且有后继(树中的最大关键字的节点无后继)。那么 后继是 给点节点 最早有左孩子的底层祖先。
拿上面样图中 13 这个节点的 举例 。13的 第一个祖先 是 7 ,由于 13 是7的右孩子,所以肯定比 7 大,而 7的左孩子也肯定比 13 小 , 以此类推, 到 6 的时候,是 祖先的 左孩子 , 说明 6 的祖先 肯定 比 13 , 也是祖先中比 13 大的 最小的节点。
后置
/** * 获取节点的后继 * @param Node $node * @return Node|null * @author zxqc2018 */ public function getSuccessor(Node $node) { //是否有右孩子 if (!is_null($node->getRight())) { return $this->findMinNode($node->getRight()); } $y = $node->getParent(); //向上逐层判断是否为祖先的右孩子 while (!is_null($y) && $node === $y->getRight()) { $node = $y; $y = $y->getParent(); } return $y; }
前驱
/** * 获取节点的前驱 * @param Node $node * @return Node|null * @author zxqc2018 */ public function getPredecessor(Node $node) { //是否有左孩子 if (!is_null($node->getLeft())) { return $this->findMaxNode($node->getLeft()); } $y = $node->getParent(); //向上逐层判断是否为祖先的左孩子 while (!is_null($y) && $node === $y->getLeft()) { $node = $y; $y = $y->getParent(); } return $y; }
五 插入
/** * 插入节点key * @param int $key * @return Node * @author zxqc2018 */ public function insert(int $key) { $x = $this->getRoot(); $y = null; $z = new Node($key); while (!is_null($x)) { $y = $x; if ($key < $x->getKey()) { $x = $x->getLeft(); } else { $x = $x->getRight(); } } //设置插入节点的父节点 $z->setParent($y); //假如树还没根节点 if (is_null($y)) { $this->root = $z; } else if ($key < $y->getKey()) { $y->setLeft($z); } else { $y->setRight($z); } return $z; }
六 删除
删除的情况比较复杂可以分为3种
假如 删除节点 为 z
1) z没有孩子
z的父节点用null 来替换 $z节点
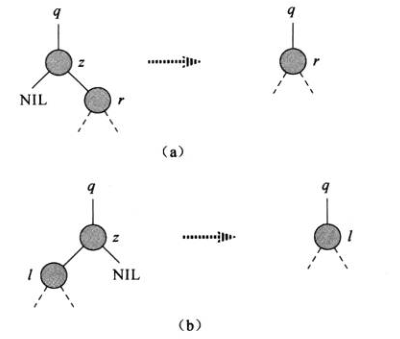
2) z有一个孩子
假如z有一个右孩子, z的右孩子 替换 z, 并且 z右孩子的父节点指向 z的父节点 ,如下图
3) z有两个孩子
可以找到$z节点的后继或者前驱节点来替换$z, 达到删除,并且不破坏树结构的目的。 这里选后继来举例, 可以分成2种情况
假如 后继节点 为 y
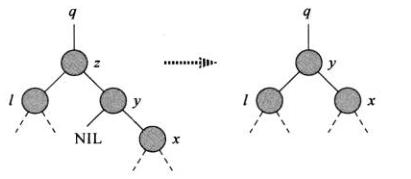
a) z的右孩子就是它的后继节点
y 替换 z 节点, y的左孩子指向 z 的 左孩子, z的 左孩子的 父节点指向 y, y的父节点指向 z 节点的父节点
这里由个情况要说明就是 , z 的 后继节点 的左孩子肯定为null, 假如不是null 的话那么z 的后继就是y的左孩子了, 所以 z的后继 y 肯定是没有左孩子的
b) z的右孩子不是它的后继节点
这情况通过转换下就可以和上面情况一致了,所以只需转换下就OK了
y的右孩子替换 y, y 的右孩子 改成 z 的右孩子, z 的右孩子的 父节点 由 z 改为 y, 这样转换后 就和上面的情况一致了
为什么可以这样转换?
y的右孩子替换 y, 这操作 等同于 删除y 节点 操作
y改为 z 的 右孩子的 父亲, 因为 y 是z 的后继 所以 y 肯定是 z 的右边 子树 中最小的, 所以 y 可以 作为 z 的 右孩子的父亲 , 没有破坏 树的结构

删除代码
/** * 移动节点 * @param Node $src 源节点 * @param Node $dst 目标节点 * @author zxqc2018 */ protected function transplantNode(?Node $src, Node $dst) { if (is_null($dst->getParent())) { $this->root = $src; }else if ($dst === $dst->getParent()->getLeft()) { $dst->getParent()->setLeft($src); } else { $dst->getParent()->setRight($src); } //源节点不空,则把源节点父节点指向目标节点的父节点 if (!is_null($src)) { $src->setParent($dst->getParent()); } } /** * 删除节点 * @param Node $node * @author zxqc2018 */ public function delete(Node $node) { if (is_null($node->getLeft())) { $this->transplantNode($node->getRight(), $node); } else if (is_null($node->getRight())) { $this->transplantNode($node->getLeft(), $node); } else { $successorNode = $this->getSuccessor($node); //删除节点的右孩子不是后继节点,则做相应转换 if ($node->getRight() !== $successorNode) { //后继节点的右孩子替换后继节点 $this->transplantNode($successorNode->getRight(), $successorNode); //设置删除节点的右孩子为后继节点的右孩子 $successorNode->setRight($node->getRight()); //删除节点的右孩子的父节点改为后继节点 $successorNode->getRight()->setParent($successorNode); } //后继节点替换删除节点 $this->transplantNode($successorNode, $node); //设置删除节点的左孩子为后继节点的左孩子 $successorNode->setLeft($node->getLeft()); //删除节点的左孩子的父节点改为后继节点 $successorNode->getLeft()->setParent($successorNode); } }
代码地址