预备知识：线段树，DFS序
DFS序
DFS序，字面意思，就是DFS到的顺序
我们只需要在搜到节点的时候记录一下就可以了
比如这样可能是一个树的DFS序
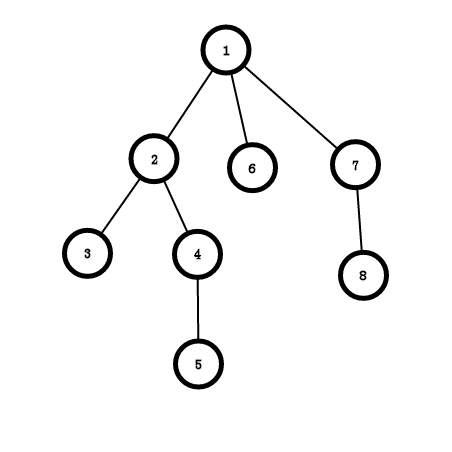
那知道了DFS序又有什么用呢
我们可以发现一颗子树在DFS序上是连续的一段
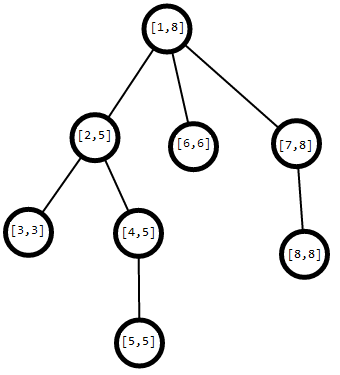
那么它就有了一个区间的性质，每个节点对应一段区间，那么我们在进行一些操作像什么：将以某节点为根的子树节点都加上x时，就可以转化为在线段树内的区间加了
树链剖分
首先我们要清楚一些概念：
重儿子（节点）：子树结点数目最多的结点
轻儿子（节点）：除了重儿子以外的结点
重边：父亲结点和重儿子连成的边
轻边：父亲节点和轻儿子连成的边
重链：由多条重边连接而成的路径
轻链：由多条轻边连接而成的路径
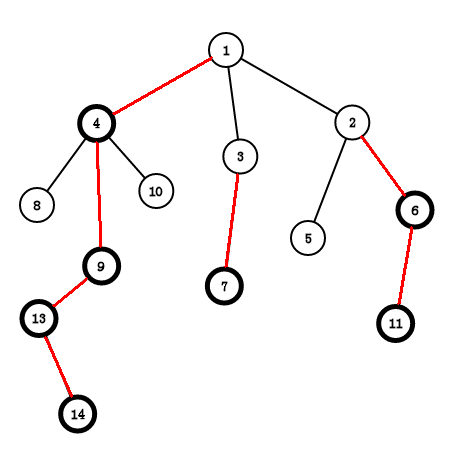
看图感受一下，
可以看到：对于1节点4的子树的节点比2,3的多，所以4是重儿子
对于4节点9的子树的节点比8,9的多，所以9是重儿子
以此类推
所以结果是：图中黑色的节点是重儿子，其余的节点是轻儿子
红边是重边，黑边是轻边
1 - 4 - 9 - 13 - 14；3 - 7；2 - 6 - 11是重链
我们发现：叶节点没有重儿子，非叶节点有且只有1个重儿子
并不是轻儿子之后全是轻儿子，比如3后面就有6和11两个重儿子
我们就可以这样理解：
当一个节点选了他的重儿子之后，我们并不能保证它的轻儿子就是叶节点，所以我们就以这个轻儿子为根，再去选这个轻儿子的轻重儿子
也就是一个DFS的过程，这样我们就会得到很多重链
有如下两个性质：
1.轻边(u,v)中, size(u)≤ size(u/2)
2.从根到某一点的路径上,不超过logn条轻链和不超过logn条重链。
那我们要怎么求出轻重儿子呢
这里我们定义几个数组：
son[u]：表示u的重儿子
size[u]：表示以u为根的树的节点个数，如上图的size[1] = 13
在这里，我们还可以顺带的求出
f[u]：节点的父节点
dep[u]：节点的深度
//u是当前节点
//fa是当前节点的父节点
void dfs1(int u, int fa) {
size[u] = 1;//表示刚搜到u的时候以u为根的子树里只有u一个节点
for (int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;//连向的节点
if (v != fa) {//因为连的是无相边，而且是树，不能往上搜，所以我们要判断u是不是从fa搜过来，也就是判断v是不是u的子节点，也可以写作!dep[v](没有被搜到过)
dep[v] = dep[u] + 1;//v的深度是当前节点的深度+1
f[v] = u;//记录一下父亲
dfs1(v, u);//继续往下搜，一直搜到叶节点为止
size[u] += size[v];//往上回溯，更新以u为根的子树的size
if (size[v] > size[son[u]]) son[u] = v;//重儿子是节点个数更多的子树，如果以u的子树中，以v为根的子树节点多，那就更新一下u的重儿子为v
}
}
}
这样我们就求出了这棵树的所有重儿子，所有点的深度，和所有点的父亲。
既然我们已经求出来重儿子，轻儿子那么我们就要把他们连成链
因为我们要把轻重儿子连城链，所以我们就一定要让链上的点连续，方便我们的操作
那该怎么办呢，这就用到了我们之前提到的DFS序了
我们从根开始一遍DFS，如果有重儿子就先走重儿子（将一条重链放到一个区间内），搜到叶子节点后回溯回去再去搜轻儿子（轻链）
这一遍DFS里，我们又引入了几个数组：
top[u]：节点u所在链的顶端
id[u]：节点u的新编号（DFS序）
a[cnt]：在新编号（DFS序）下的当前点的点值
w[u]：题目中给出的节点u的点值
//u是当前节点
//t是所在链的顶端
void dfs2(int u, int t) {
id[u] = ++cnt;//给这个点一个新的编号
a[cnt] = w[u];//记录这个编号下点的值
top[u] = t;//记录u所在链的顶端为t
if (son[u]) dfs2(son[u], t);//u的重儿子和u在同一条链里
for (int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;//搜轻儿子
if (v != f[u] && v != son[u])//判断是否是轻儿子
dfs2(v, v);//以轻儿子为顶的链
}
}
为什么是dfs2(v,v)呢，因为当v是重儿子的时候，它不可能为一条链的顶，因为根据重边的定义，一定有一条边连向重儿子，若重儿子为顶，还会有一条边连向它，所以重儿子不会为顶端。
树链剖分求LCA
为什么要先讲树剖求LCA呢
因为树剖的很多题是要求要在链上进行操作的，而链上操作其实就是LCA
树剖求LCA其实也是往上跳，但这里的往上跳不是倍增的往上跳
我们这里只要把两个点跳到同一条链里就好了
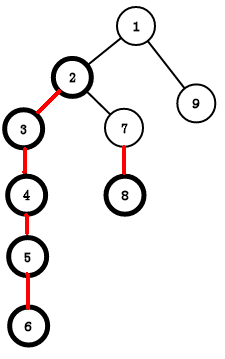
如图：我们要求LCA的话，有两种情况
1、在同一条链内，如3和5。这时直接输出深度较小的那个节点就好了
2、不再同一条链内，如6和8。因为他们不再一条链内，所以我们让其中一个点 x 直接跳到链的顶端也是没有问题的。这时我们就让那个深度深的 x 一直往上跳，这把 x 更新 x 的顶端的父亲节点，也就是到了另一条链上，记录一下它在这条新链上的顶端是谁，用来判断和另一个点 y 是否在同一条链内。当 x 跳到它所在的链的顶端时，深度可能就小于 y 的顶端了，也就是在另一条链的上面(这时 x 不会再往上跳，y 也不会再往上跳，但没有找到LCA)，这时我们就要交换他们（其实只是换了个名字，管原来的 y 叫 x ，管原来的 x 叫 y，原来的树并没有变，想了很久），保证 x , y 会更新到同一条链内
稍微模拟一下
如图：
如果我们要求6和12的LCA
设x = 12,y = 1
这时我们先比较一下两者的top，fx = top[x] = 12，fy = top[y] = 1
发现他们的top不一样
那就往上跳呗，dep[fx] ＞ dep[fy]，所以 x = f[fx] = 9,fx = top[x] = 7
12往上跳跳到了9
变成了这样
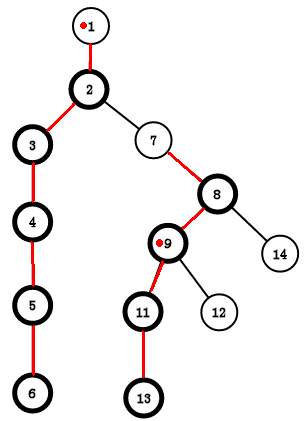
找到9所在链的顶为7
发现7 != 1说明9和1不再同一个链里
继续往上跳
x = top[fx] = 2，fx = top[2] = 1
发现1 == 1
说明我们现在的 x 和 y 在同一条链里了
这时我们就返回深度小的那个就可以了
//fx表示x所在链的顶，fy表示y所在链的顶
int LCA(int x, int y) {
int fx = top[x], fy = top[y];
while (fx != fy) {
if (dep[fx] < dep[fy]) swap(x, y), swap(fx, fy);
x = f[fx], fx = top[x];
}
return dep[x] < dep[y] ? x : y;//最后返回深度小的那个
}
如果还有边权的话，只要在dfs1里加1句话就可以了
void dfs1(int u, int fa) {
size[u] = 1;
for (int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;
if (v != fa) {
dep[v] = dep[u] + 1;
f[v] = u;
dis[v] = dis[u] + e[i].w;//here
dfs1(v, u);
size[u] += size[v];
if (size[v] > size[son[u]]) son[u] = v;
}
}
}
dis[u]表示从根到u的距离，其实倍增，tarjan都是这么着
查询距离的时候还是
dis[x] + dis[y] - 2 * dis[LCA(x, y)]
路径上的修改/查询
讲完了LCA，剩下的路径上修改和查询就和LCA差不多了，只是加了几句话而已
这里要结合我们上面的DFS序和LCA
那对于路径上的操作，我们先想LCA，在求LCA的时候，我们每次都往上跳一条链，直到这两个点在一个路径上为止
就像我们刚开始的找6的top一样
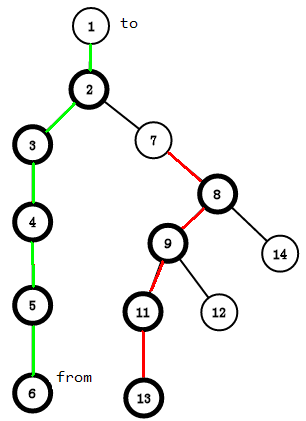
一次就经过了这么一条链（绿边）
我们再回想一下DFS序的性质，会得到在这一条链上，新的编号是连续的，我们要对这一条链进行修改和查询操作，这让我们想到了什么
线段树！！
线段树最支持的不就是区间修改和查询吗
那么我们就可以用线段树维护这一条路径了。
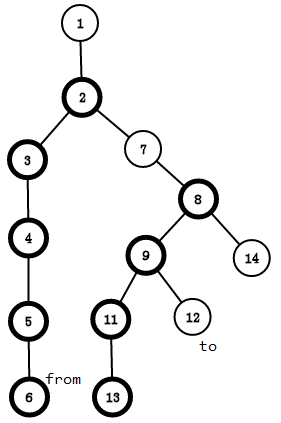
那如果我们要维护6到12这样一条路径呢？
还记得我们求LCA的时候是怎么往上跳的吗
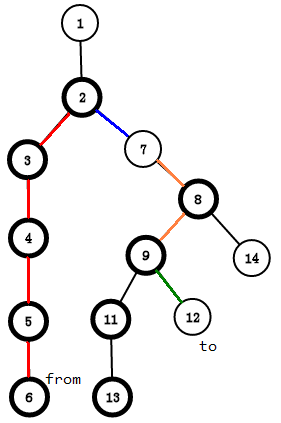
左半部分的12往上跳，每次都跳到所在链的顶端（只记录初末位置），我们可以得到12跳到的当前点 u 所处的链的顶的新编号（跳到9，顶为7），我们又知道当前点 u 的新编号，那我们就可以用线段树对从顶段到当前点 u 这条路径进行操作了，这样我们每次修改一条链（可能是一部分），就完成了路径的操作；
跳到2的时候，fx == fy，两点在同一条链内，x = 2，y = 6，然后我们操作 id[2] 到 id[6] ，这样我们就一条链一条链的完成了对这条路径的操作
全程我们操作的区间是[id[2],id[6]]，[id[7],id[9]]，[id[12],id[12]]
我们不对蓝边上的点和绿边上的点操作，因为他们不是重链，只是注明一下点经过的路径，若对它们操作的话会算重复
我刚写完这句话的时候想到了一个error
如果图长这样
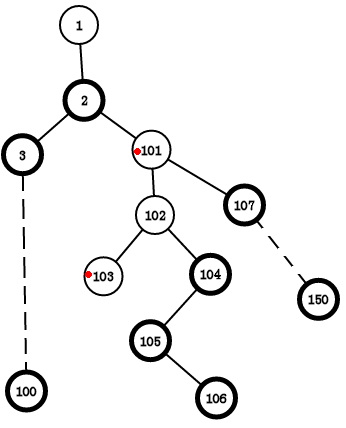（虚线是我省略了那一串的点）
如果我们要查询 103 到 101 呢，它们之间没有重链啊，那只好就一个一个的往上跳了，103->102->101.（退化成单点操作）
//修改
void update_chain(int x, int y, int z) {
int fx = top[x], fy = top[y];
while (fx != fy) {
if (dep[fx] < dep[fy]) swap(x, y), swap(fx, fy);
update(id[fx], id[x], z, 1, cnt, 1);//每往上跳一次，就修改以次经过路径上的值，因为DFS序中id[fx] < id[x]，所以是区间[id[fx],id[x]];
x = f[fx], fx = top[x];
}
if (id[x] > id[y]) swap(x, y);//要保证区间是从小到大的
update(id[x], id[y], z, 1, cnt, 1);
}
//查询
int query_chain(int x, int y) {
int ans = 0, fx = top[x], fy = top[y];
while (fx != fy) {
if (dep[fx] < dep[fy]) swap(x, y), swap(fx, fy);
ans += query(id[fx], id[x], 1, cnt, 1);
x = f[fx], fx = top[x];
}
if (id[x] > id[y]) swap(x, y);
ans += query(id[x], id[y], 1, cnt, 1);
return ans;
}
到此为止，我们就完成了树链剖分的基本操作
完整代码：
#include <bits/stdc++.h>
using namespace std;
const int N = 1e6 + 10;
int n, m, num, cnt, rt, cntt, k, s, mod;
int head[N], size[N], top[N], f[N], son[N], dep[N], a[N], id[N], w[N];
struct node {
int v, nx;
} e[N];
struct tree {
int sum, lazy;
int len;
} t[N];
#define lson rt << 1
#define rson rt << 1 | 1
template<class T>inline void read(T &x) {
x = 0; int f = 0; char ch = getchar();
while (!isdigit(ch)) f |= (ch == '-'), ch = getchar();
while (isdigit(ch)) x = x * 10 + ch - '0', ch = getchar();
x = f ? -x : x;
return ;
}
inline void add(int u, int v) {
e[++num].nx = head[u], e[num].v = v, head[u] = num;
}
void dfs1(int u, int fa) {
size[u] = 1;
for (int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;
if (v != fa) {
dep[v] = dep[u] + 1;
f[v] = u;
dfs1(v, u);
size[u] += size[v];
if (size[v] > size[son[u]]) son[u] = v;
}
}
}
void dfs2(int u, int t) {
id[u] = ++ cnt;
a[cnt] = w[u];
top[u] = t;
if (son[u]) dfs2(son[u], t);
for (int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;
if (v != f[u] && v != son[u]) dfs2(v, v);
}
}
inline void pushup(int rt) {
t[rt].sum = t[lson].sum + t[rson].sum;
}
void build(int l, int r, int rt) {
t[rt].len = r - l + 1;
if (l == r) {
t[rt].sum = a[l];
return;
}
int m = (l + r) >> 1;
build(l, m, lson);
build(m + 1, r, rson);
pushup(rt);
}
inline void pushdown(int rt) {
if (t[rt].lazy) {
t[lson].lazy += t[rt].lazy, t[lson].lazy %= mod;
t[rson].lazy += t[rt].lazy, t[rson].lazy %= mod;
t[lson].sum += t[rt].lazy * t[lson].len, t[lson].sum %= mod;
t[rson].sum += t[rt].lazy * t[rson].len, t[rson].sum %= mod;
t[rt].lazy = 0;
}
}
void update(int L, int R, int c, int l, int r, int rt) {
if (L <= l && r <= R) {
t[rt].sum += (t[rt].len * c) % mod;
t[rt].lazy += c;
return ;
}
pushdown(rt);
int m = (l + r) >> 1;
if (L <= m) update(L, R, c, l, m, lson);
if (R > m) update(L, R, c, m + 1, r, rson);
pushup(rt);
}
int query(int L, int R, int l, int r, int rt) {
if (L <= l && r <= R) return t[rt].sum;
pushdown(rt);
int m = (l + r) >> 1, ans = 0;
if (L <= m) ans += query(L, R, l, m, lson) % mod;
if (R > m) ans += query(L, R, m + 1, r, rson) % mod;
return ans % mod;
}
void update_chain(int x, int y, int z) {
int fx = top[x], fy = top[y];
while (fx != fy) {
if (dep[fx] < dep[fy]) swap(x, y), swap(fx, fy);
update(id[fx], id[x], z, 1, cnt, 1);
x = f[fx], fx = top[x];
}
if (id[x] > id[y]) swap(x, y);
update(id[x], id[y], z, 1, cnt, 1);
}
int query_chain(int x, int y) {
int ans = 0, fx = top[x], fy = top[y];
while (fx != fy) {
if (dep[fx] < dep[fy]) swap(x, y), swap(fx, fy);
ans += query(id[fx], id[x], 1, cnt, 1);
x = f[fx], fx = top[x];
}
if (id[x] > id[y]) swap(x, y);
ans += query(id[x], id[y], 1, cnt, 1);
return ans % mod;
}
int main(int argc, char const *argv[]) {
memset(head, -1, sizeof(head));
read(n), read(m), read(s), read(mod);
for (int i = 1; i <= n; ++ i) read(w[i]);
for (int i = 1, x, y; i < n; ++ i) {
read(x), read(y);
add(x, y), add(y, x);
}
f[s] = 1, dep[s] = 0;
dfs1(s, 0);
dfs2(s, s);
build(1, n, 1);
for (int i = 1, x, y, z; i <= m; ++i) {
read(k), read(x);
if (k == 1) {read(y), read(z); update_chain(x, y, z);}
if (k == 2) {read(y); printf("%d
", query_chain(x, y));}
if (k == 3) {read(z); update(id[x], id[x] + size[x] - 1, z, 1, n, 1);}
if (k == 4) printf("%d
", query(id[x], id[x] + size[x] - 1, 1, n, 1) % mod);
}
return 0;
}
例题：
#include <bits/stdc++.h>
#define ll long long
using namespace std;
const int N = 5e6 + 10;
int n, m, num, cnt, cntt, rt;
int head[N], dep[N], f[N], id[N], size[N], w[N], son[N], a[N], top[N];
struct node {
int v, nx;
} e[N];
struct tree {
ll sum, lazy;
int len;
} t[N];
#define lson rt << 1
#define rson rt << 1 | 1
template<class T>inline void read(T &x) {
x = 0; int f = 0; char ch = getchar();
while (!isdigit(ch)) f |= (ch == '-'), ch = getchar();
while (isdigit(ch)) x = x * 10 + ch - '0', ch = getchar();
x = f ? -x : x;
return;
}
inline void add(int u, int v) {
e[++num].nx = head[u], e[num].v = v, head[u] = num;
}
void dfs1(int u, int fa) {
size[u] = 1;
for (int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;
if (v != fa) {
dep[v] = dep[u] + 1;
f[v] = u;
dfs1(v, u);
size[u] += size[v];
if (size[v] > size[son[u]]) son[u] = v;
}
}
}
void dfs2(int u, int t) {
id[u] = ++ cnt;
a[cnt] = w[u];
top[u] = t;
if (son[u]) dfs2(son[u], t);
for (int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;
if (v != f[u] && v != son[u]) dfs2(v, v);
}
}
inline void pushup(int rt) {
t[rt].sum = t[lson].sum + t[rson].sum;
}
void build(int l, int r, int rt) {
t[rt].len = r - l + 1;
if (l == r) {
t[rt].sum = a[l];
return;
}
int m = l + r >> 1;
build(l, m, lson);
build(m + 1, r, rson);
pushup(rt);
}
inline void pushdown(int rt) {
if (t[rt].lazy) {
t[lson].lazy += t[rt].lazy;
t[rson].lazy += t[rt].lazy;
t[lson].sum += t[rt].lazy * t[lson].len;
t[rson].sum += t[rt].lazy * t[rson].len;
t[rt].lazy = 0;
}
}
void update(int L, int R, ll c, int l, int r, int rt) {
if (L <= l && r <= R) {
t[rt].sum += c * t[rt].len;
t[rt].lazy += c;
return ;
}
int m = l + r >> 1;
pushdown(rt);
if (L <= m) update(L, R, c, l, m, lson);
if (R > m) update(L, R, c, m + 1, r, rson);
pushup(rt);
}
ll query(int L, int R, int l, int r, int rt) {
if (L <= l && r <= R) return t[rt].sum;
pushdown(rt);
int m = l + r >> 1;
ll ans = 0;
if (L <= m) ans += query(L, R, l, m, lson);
if (R > m) ans += query(L, R, m + 1, r, rson);
return ans;
}
ll query_chain(int x, int y) {
ll ans = 0;
int fx = top[x], fy = top[y];
while (fx != fy) {
if (dep[fx] < dep[fy]) swap(x, y), swap(fx, fy);
ans += query(id[fx], id[x], 1, cnt, 1);
x = f[fx], fx = top[x];
}
if (id[x] > id[y]) swap(x, y);
ans += query(id[x], id[y], 1, cnt, 1);
return ans;
}
int main(int argc, char const *argv[]) {
memset(head, -1, sizeof(head));
read(n), read(m);
for (int i = 1; i <= n; ++ i) read(w[i]);
for (int i = 1, x, y; i < n; ++ i) {
read(x), read(y);
add(x, y), add(y, x);
}
dep[1] = 1;
dfs1(1, 0), dfs2(1, 1);
build(1, n, 1);
for (int i = 1, x, y, z; i <= m; ++ i) {
read(x), read(y);
if (x == 1) read(z), update(id[y], id[y], z, 1, n, 1);
if (x == 2) read(z), update(id[y], id[y] + size[y] - 1, z, 1, n, 1);
if (x == 3) printf("%lld
",query_chain(1,y));
}
return 0;
}
#include <bits/stdc++.h>
using namespace std;
const int N = 1e5 + 10;
int n, m, num, cnt, cntt, rt;
int head[N], size[N], dep[N], f[N], id[N], w[N], a[N], top[N], son[N];
string s;
struct node {
int v, nx;
} e[N];
struct tree {
int sum, lazy, mx;
int len;
} t[N];
#define lson rt << 1
#define rson rt << 1 | 1
template<class T> inline void read(T &x) {
x = 0; int f = 0; char ch = getchar();
while (!isdigit(ch)) f |= (ch == '-'), ch = getchar();
while (isdigit(ch)) x = x * 10 + ch - '0', ch = getchar();
x = f ? -x : x;
return;
}
inline void add(int u, int v) {
e[++num].nx = head[u], e[num].v = v, head[u] = num;
}
void dfs1(int u, int fa) {
size[u] = 1;
for (int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;
if (v != fa) {
f[v] = u;
dep[v] = dep[u] + 1;
dfs1(v, u);
size[u] += size[v];
if (size[v] > size[son[u]]) son[u] = v;
}
}
}
void dfs2(int u, int t) {
id[u] = ++ cnt;
a[cnt] = w[u];
top[u] = t;
if (son[u]) dfs2(son[u],t);
for (int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;
if (v != f[u] && v != son[u]) dfs2(v, v);
}
}
inline void pushup(int rt) {
t[rt].sum = t[lson].sum + t[rson].sum;
t[rt].mx = max(t[lson].mx, t[rson].mx);
}
void build(int l, int r, int rt) {
t[rt].len = r - l + 1;
if (l == r) {
t[rt].sum = t[rt].mx = a[l];
return;
}
int m = l + r >> 1;
build(l, m, lson);
build(m + 1, r, rson);
pushup(rt);
}
void update(int L, int c, int l, int r, int rt) {
if (l == r) {
t[rt].sum = c;
t[rt].mx = c;
return;
}
int m = l + r >> 1;
if (L <= m) update(L, c, l, m, lson);
else update(L, c, m + 1, r, rson);
pushup(rt);
}
int query_sum(int L, int R, int l, int r, int rt) {
if (L <= l && r <= R) return t[rt].sum;
int m = l + r >> 1, ans = 0;
if (L <= m) ans += query_sum(L, R, l, m, lson);
if (R > m) ans += query_sum(L, R, m + 1, r, rson);
return ans;
}
int query_max(int L, int R, int l, int r, int rt) {
if (L <= l && r <= R) return t[rt].mx;
int m = l + r >> 1, ans = -0x3f3f3f3f;
if (L <= m) ans = max(ans, query_max(L, R, l, m, lson));
if (R > m) ans = max(ans, query_max(L, R, m + 1, r, rson));
return ans;
}
int query_chain_sum(int x, int y) {
int ans = 0, fx = top[x], fy = top[y];
while (fx != fy) {
if (dep[fx] < dep[fy]) swap(x, y), swap(fx, fy);
ans += query_sum(id[fx], id[x], 1, cnt, 1);
x = f[fx], fx = top[x];
}
if (id[x] > id[y]) swap(x, y);
ans += query_sum(id[x], id[y], 1, cnt, 1);
return ans;
}
int query_chain_max(int x, int y) {
int ans = -0x3f3f3f3f, fx = top[x], fy = top[y];
while (fx != fy) {
if (dep[fx] < dep[fy]) swap(x, y), swap(fx, fy);
ans = max(ans, query_max(id[fx], id[x], 1, cnt, 1));
x = f[fx], fx = top[x];
}
if (id[x] > id[y]) swap(x, y);
ans = max(ans, query_max(id[x], id[y], 1, cnt, 1));
return ans;
}
int main(int argc, char const *argv[]) {
memset(head, -1, sizeof(head));
read(n);
for (int i = 1, x, y; i < n; ++ i) {
read(x), read(y);
add(x, y), add(y, x);
}
for (int i = 1; i <= n; ++ i) read(w[i]);
f[1] = 0, dep[1] = 1;
dfs1(1, 0), dfs2(1, 1);
build(1, n, 1);
read(m);
for (int i = 1, x, y; i <= m; ++ i) {
cin >> s;
if (s == "CHANGE") read(x), read(y), update(id[x], y, 1, n, 1);
if (s == "QMAX") read(x), read(y), printf("%d
", query_chain_max(x, y));
if (s == "QSUM") read(x), read(y), printf("%d
", query_chain_sum(x, y));
}
return 0;
}
//路径最值
#include <bits/stdc++.h>
using namespace std;
const int N = 2e5 + 10;
int n, m, num, cnt;
int head[N], f[N], top[N], son[N], size[N], dep[N], id[N];
struct node {
int v, nx;
} e[N];
struct tree {
int mx;
int lazy;
} t[N];
#define lson rt << 1
#define rson rt << 1 | 1
template<class T>inline void read(T &x) {
x = 0; int f = 0; char ch = getchar();
while (!isdigit(ch)) f |= (ch == '-'), ch = getchar();
while (isdigit(ch)) x = x * 10 + ch - '0', ch = getchar();
x = f ? -x : x;
return;
}
inline void add(int u, int v) {
e[++num].nx = head[u], e[num].v = v, head[u] = num;
}
void dfs1(int u, int fa) {
size[u] = 1;
for (int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;
if (v != fa) {
dep[v] = dep[u] + 1;
f[v] = u;
dfs1(v, u);
size[u] += size[v];
if (size[v] > size[son[u]]) son[u] = v;
}
}
}
void dfs2(int u, int t) {
id[u] = ++cnt;
top[u] = t;
if (son[u]) dfs2(son[u], t);
for (int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;
if (v != f[u] && v != son[u]) dfs2(v, v);
}
}
inline void pushup(int rt) {
t[rt].mx = max(t[lson].mx, t[rson].mx);
}
void build(int l, int r, int rt) {
if (l == r) return ;
int m = (l + r) >> 1;
build(l, m, lson);
build(m + 1, r, rson);
}
void pushdown(int rt) {
if (t[rt].lazy) {
t[lson].lazy += t[rt].lazy;
t[rson].lazy += t[rt].lazy;
t[lson].mx += t[rt].lazy;
t[rson].mx += t[rt].lazy;
t[rt].lazy = 0;
}
}
void update(int L, int R, int c, int l, int r, int rt) {
if (L <= l && r <= R) {
t[rt].lazy += c;
t[rt].mx += c;
return;
}
int m = (l + r) >> 1;
pushdown(rt);
if (L <= m) update(L, R, c, l, m, lson);
if (R > m) update(L, R, c, m + 1, r, rson);
pushup(rt);
}
int query(int L, int R, int l, int r, int rt) {
if (L <= l && r <= R) return t[rt].mx;
int m = (l + r) >> 1, ans = -0x3f3f3f3f;
if (L <= m) ans = max(ans, query(L, R, l, m, lson));
if (R > m) ans = max(ans, query(L, R, m + 1, r, rson));
return ans;
}
void update_chain(int x, int y) {
int fx = top[x], fy = top[y];
while (fx != fy) {
if (dep[fx] < dep[fy]) swap(x, y), swap(fx, fy);
update(id[fx], id[x], 1, 1, cnt, 1);
x = f[fx], fx = top[x];
}
if (id[x] > id[y]) swap(x, y);
update(id[x], id[y], 1, 1, cnt, 1);
}
int main(int argc, char const *argv[]) {
memset(head, -1, sizeof(head));
read(n), read(m);
for (int i = 1, x, y; i < n; ++i) {
read(x), read(y);
add(x, y), add(y, x);
}
f[1] = 0, dep[1] = 1;
dfs1(1, 0), dfs2(1, 1);
build(1, n, 1);
for (int i = 1, x, y; i <= m; ++i) {
read(x), read(y);
if (x > y) swap(x, y);
update_chain(x, y);
}
printf("%d
", query(id[1], id[1] + size[1] - 1, 1, n, 1));
return 0;
}
//树链剖分，但是要转换一下，把边权转化为所连向点的点权，查询的时候要忽略最上面的点
#include <bits/stdc++.h>
using namespace std;
const int N = 1e6 + 10;
int n, num, cnt, x, y, z;
int dep[N], f[N], top[N], size[N], son[N], a[N], w[N], head[N], mx[N], id[N];
struct node {
int v, nx, w;
} e[N];
#define lson rt << 1
#define rson rt << 1 | 1
template<class T>inline void read(T &x) {
x = 0; int f = 0; char ch = getchar();
while (!isdigit(ch)) f |= (ch == '-'), ch = getchar();
while (isdigit(ch)) x = x * 10 + ch - '0', ch = getchar();
x = f ? -x : x;
return ;
}
inline void add(int u, int v, int w) {
e[++num].nx = head[u], e[num].v = v, e[num].w = w, head[u] = num;
}
void dfs1(int u, int fa) {
size[u] = 1;
for (int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;
if (v != fa) {
dep[v] = dep[u] + 1;
f[v] = u;
w[v] = e[i].w;
dfs1(v, u);
size[u] += size[v];
if (size[v] > size[son[u]]) son[u] = v;
}
}
}
void dfs2(int u, int t) {
id[u] = ++cnt;
a[cnt] = w[u];
top[u] = t;
if (son[u]) dfs2(son[u], t);
for (int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;
if (v != f[u] && v != son[u]) dfs2(v, v);
}
}
inline void pushup(int rt) {
mx[rt] = max(mx[lson], mx[rson]);
}
void build(int l, int r, int rt) {
if (l == r) {
mx[rt] = a[l];
return ;
}
int m = (l + r) >> 1;
build(l, m, lson);
build(m + 1, r, rson);
pushup(rt);
}
void update(int L, int c, int l, int r, int rt) {
if (l == r) {
mx[rt] = c;
return ;
}
int m = (l + r) >> 1;
if (L <= m) update(L, c, l, m, lson);
else update(L, c, m + 1, r, rson);
pushup(rt);
}
int query(int L, int R, int l, int r, int rt) {
if (L <= l && r <= R) return mx[rt];
int m = (l + r) >> 1, ans = -0x3f3f3f3f;
if (L <= m) ans = max(ans, query(L, R, l, m, lson));
if (R > m) ans = max(ans, query(L, R, m + 1, r, rson));
return ans;
}
int query_chain(int x, int y) {
int fx = top[x], fy = top[y], ans = -0x3f3f3f3f;
while (fx != fy) {
if (dep[fx] < dep[fy]) swap(x, y), swap(fx, fy);
ans = max(ans, query(id[fx], id[x], 1, cnt, 1));
x = f[fx], fx = top[x];
}
if (id[x] > id[y]) swap(x, y);
ans = max(ans, query(id[x] + 1, id[y], 1, cnt, 1));
return ans;
}
int main(int argc, char const *argv[]) {
memset(head, -1, sizeof(head));
read(n);
for (int i = 1; i < n; ++i) {
read(x), read(y), read(z);
add(x, y, z), add(y, x, z);
}
f[1] = 0, dep[1] = 1;
dfs1(1, 0), dfs2(1, 1);
build(1,n,1);
while (1) {
string s;
cin >> s;
if (s == "QUERY") {
read(x), read(y);
if (x == y) printf("0
");
else printf("%d
", query_chain(x, y));
}
else if (s == "CHANGE") {
read(x), read(y);
x = (x << 1) - 1;
update(id[e[x].v],y,1,n,1);
}
else break;
}
return 0;
}
P3038 [USACO11DEC]牧草种植Grass Planting
#include <bits/stdc++.h>
using namespace std;
const int N = 4e5 + 10;
int n,m,cnt,num;
int top[N],f[N],son[N],size[N],id[N],head[N],dep[N];
struct node {
int v,nx;
}e[N];
struct tree {
int sum;
int lazy;
}t[N];
#define lson rt << 1
#define rson rt << 1 | 1
template<class T>inline void read(T &x) {
x = 0;int f = 0;char ch = getchar();
while(!isdigit(ch)) f |= (ch == '-'),ch = getchar();
while(isdigit(ch)) x = x * 10 + ch - '0',ch = getchar();
x = f ? -x : x;
return;
}
inline void add(int u,int v) {
e[++num].nx = head[u],e[num].v = v,head[u] = num;
}
void dfs1(int u,int fa) {
size[u] = 1;
for(int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;
if(v != fa) {
dep[v] = dep[u] + 1;
f[v] = u;
dfs1(v,u);
size[u] += size[v];
if(size[v] > size[son[u]]) son[u] = v;
}
}
}
void dfs2(int u,int t) {
top[u] = t;
id[u] = ++cnt;
if(son[u]) dfs2(son[u],t);
for(int i = head[u]; ~i; i = e[i].nx) {
int v = e[i].v;
if(v != f[u] && v != son[u]) dfs2(v,v);
}
}
inline void pushup(int rt) {
t[rt].sum = t[lson].sum + t[rson].sum;
}
inline void pushdown(int l,int r,int rt) {
if(t[rt].lazy) {
t[lson].lazy += t[rt].lazy;
t[rson].lazy += t[rt].lazy;
t[lson].sum += t[rt].lazy * l;
t[rson].sum += t[rt].lazy * r;
t[rt].lazy = 0;
}
}
void update(int L,int R,int l,int r,int rt) {
if(L <= l && r <= R) {
t[rt].sum += (r - l + 1);
t[rt].lazy += 1;
return ;
}
int m = (l + r) >> 1;
pushdown(m - l + 1,r - m,rt);
if(L <= m) update(L,R,l,m,lson);
if(R > m) update(L,R,m + 1,r,rson);
pushup(rt);
}
int query(int L,int R,int l,int r,int rt) {
if(L <= l && r <= R) return t[rt].sum;
int m = (l + r) >> 1,ans = 0;
pushdown(m - l + 1,r - m,rt);
if(L <= m) ans += query(L,R,l,m,lson);
if(R > m) ans += query(L,R,m + 1,r,rson);
return ans;
}
void update_chain(int x,int y) {
int fx = top[x],fy = top[y];
while(fx != fy) {
if(dep[fx] < dep[fy]) swap(x,y),swap(fx,fy);
update(id[fx],id[x],1,cnt,1);
x = f[fx],fx = top[x];
}
if(id[x] > id[y]) swap(x,y);
update(id[x] + 1,id[y],1,cnt,1);
}
int query_chain(int x,int y) {
int fx = top[x],fy = top[y],ans = 0;
while(fx != fy) {
if(dep[fx] < dep[fy]) swap(x,y),swap(fx,fy);
ans += query(id[fx],id[x],1,cnt,1);
x = f[fx],fx = top[x];
}
if(id[x] > id[y]) swap(x,y);
ans += query(id[x] + 1,id[y],1,cnt,1);
return ans;
}
int main(int argc, char const *argv[]) {
memset(head,-1,sizeof(head));
read(n),read(m);
for(int i = 1,x,y; i < n; ++i) {
read(x),read(y);
add(x,y),add(y,x);
}
f[1] = 0,dep[1] = 1;
dfs1(1,0),dfs2(1,1);
for(int i = 1,x,y; i <= m; ++ i) {
char c;
cin>>c;
read(x),read(y);
if(c == 'P') update_chain(x,y);
else printf("%d
", query_chain(x,y));
}
return 0;
}