Codeforces Gym102219 2019 ICPC Malaysia National J. Kitchen Plates (暴力,拓扑排序)

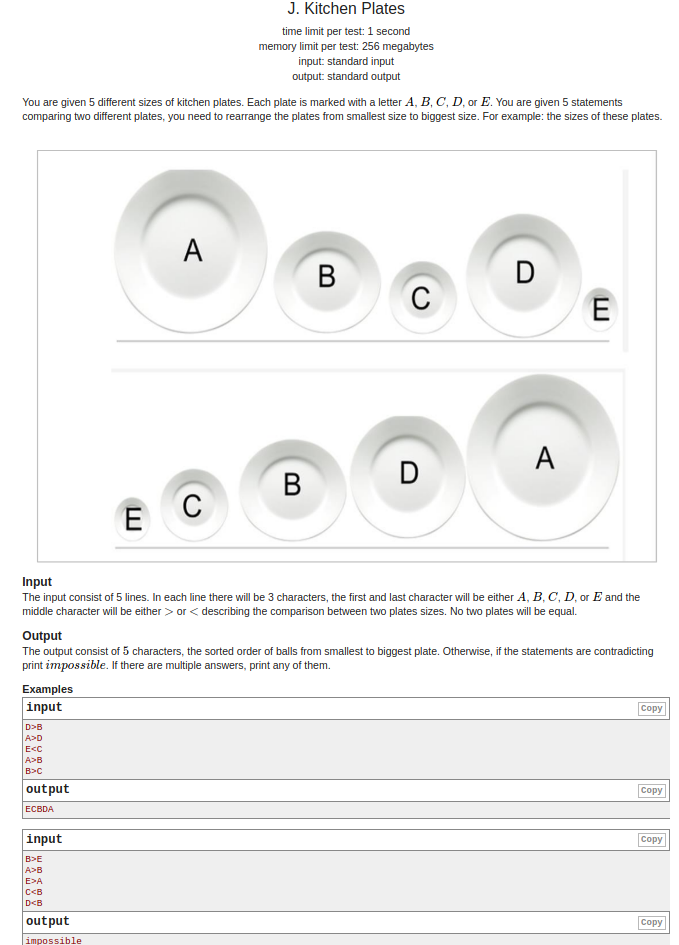
• 题意:给你5个\(A,B,C,D,E\)大小关系式,升序输出它们,如果所给的大小矛盾,输出\(impossible\).

• 题意:当时第一眼想到的就是连边然后排序,很明显是拓扑排序(然而我不会qwq,之后再补),但貌似可以直接暴力来写,用二维数组来记录两个数之间的大小关系,如果一维\(>\)二维就记录true,然后我们要对题目所给的关系进行合并,将所有大小关系弄清楚,三个for就可以搞定了,之后判断一下是否有矛盾存在,最后再统计一下大小然后再按顺序输出即可(这题的输出其实没怎么看懂,之前写的桶排输出不知道为什么不给过qaq).

• 代码:

  #include <iostream>
  #include <cstdio>
  #include <cstring>
  #include <cmath>
  #include <algorithm>
  #include <stack>
  #include <queue>
  #include <vector>
  #include <map>
  #include <set>
  #include <unordered_set>
  #include <unordered_map>
  #define ll long long
  #define fi first
  #define se second
  #define pb push_back
  #define me memset
  const int N = 1e6 + 10;
  const int mod = 1e9 + 7;
  using namespace std;
  typedef pair<int,int> PII;
  typedef pair<long,long> PLL;
   
  string s;
  int v[100][100];
  int cnt[N];
  map<int,int> mp;
  vector<int> rest;
  int main() {
      ios::sync_with_stdio(false);cin.tie(0);
      for(int i=1;i<=5;++i){
          cin>>s;
          //初始化记录
           for(int j=0;j<s.size();++j){
               if(s[1]=='>') v[s[0]-'A'][s[2]-'A']=1;
               else v[s[2]-'A'][s[0]-'A']=1;
           }
      }
      //合并
      for(int k=0;k<5;++k){
          for(int i=0;i<5;++i){
              for(int j=0;j<5;++j){
                  if(v[i][k] && v[k][j]) v[i][j]=1;
              }
          }
      }
   
      for(int i=0;i<5;++i){
          for(int j=0;j<5;++j){
              if(v[i][j] && v[j][i]){
                  puts("impossible");
                  return 0;
              }
          }
      }
      //统计大小
      for(int i=0;i<5;++i){
          for(int j=0;j<5;++j){
              if(v[i][j]) cnt[i]++;
          }
      }
      for(int len=0;len<5;++len){
          for(int num=0;num<5;++num){
              if(cnt[num]==len) printf("%c",num+'A');
          }
      }
   
      return 0;
  }
  

• 补:拓扑排序的裸题,直接写就行了

  #include <iostream>
  #include <cstdio>
  #include <cstring>
  #include <cmath>
  #include <algorithm>
  #include <stack>
  #include <queue>
  #include <vector>
  #include <map>
  #include <set>
  #include <unordered_set>
  #include <unordered_map>
  #define ll long long
  #define fi first
  #define se second
  #define pb push_back
  #define me memset
  const int N = 1e6 + 10;
  const int mod = 1e9 + 7;
  const int INF = 0x3f3f3f3f;
  using namespace std;
  typedef pair<int,int> PII;
  typedef pair<ll,ll> PLL;
  
  string s;
  vector<char> v;
  vector<char> out[N];
  int in[N];
  
  int main() {
      ios::sync_with_stdio(false);cin.tie(0);
      for(int i=1;i<=5;++i){
          cin>>s;
           if(s[1]=='>'){
               in[s[0]]++;
               out[s[2]].pb(s[0]);
           }
           else{
               in[s[2]]++;
               out[s[0]].pb(s[2]);
           }
      }
      queue<char> q;
      for(char i='A';i<='E';++i){
          if(in[i]==0) q.push(i);
      }
      while(!q.empty()){
          char tmp=q.front();
          q.pop();
          v.pb(tmp);
          for(auto w:out[tmp]){
              if(w!=-1){
                  in[w]--;
                  if(in[w]==0) q.push(w);
              }
              w=-1;
          }
      }
      if(v.size()!=5) puts("impossible");
      else{
          for(auto w:v) printf("%c",w);
      }
      return 0;
  }