问题描述
You are given a list of non-negative integers, a1, a2, ..., an, and a target, S. Now you have 2 symbols + and -. For each integer, you should choose one from + and - as its new symbol.
Find out how many ways to assign symbols to make sum of integers equal to target S.
Example 1:
Input: nums is [1, 1, 1, 1, 1], S is 3. Output: 5 Explanation: -1+1+1+1+1 = 3 +1-1+1+1+1 = 3 +1+1-1+1+1 = 3 +1+1+1-1+1 = 3 +1+1+1+1-1 = 3 There are 5 ways to assign symbols to make the sum of nums be target 3.
Note:
- The length of the given array is positive and will not exceed 20.
- The sum of elements in the given array will not exceed 1000.
- Your output answer is guaranteed to be fitted in a 32-bit integer.
参考答案
class Solution { public: int findTargetSumWays(vector<int>& nums, int s) { int sum = accumulate(nums.begin(), nums.end(), 0); return sum < s || (s + sum) & 1 ? 0 : subsetSum(nums, (s + sum) >> 1); } int subsetSum(vector<int>& nums, int s) { int dp[s + 1] = { 0 }; dp[0] = 1; for (int n : nums) for (int i = s; i >= n; i--) dp[i] += dp[i - n]; return dp[s]; } };
答案解释
1. (s+sum) & 1 是什么?
通过 &1 操作,检查(s+sum) 是否可以被2整除,只有被整除的数字,才可以继续运算。
2. (s+sum)>>1 是什么?
除以 2 的操作
3. 为什么要有以上操作?
根据 这个论坛 的解释:
sum(P) - sum(N) = target
sum(P) + sum(N) + sum(P) - sum(N) = target + sum(P) + sum(N)
2 * sum(P) = target + sum(nums)
s+sum 需要被 2 整除。
4. subsetSum 是什么函数?
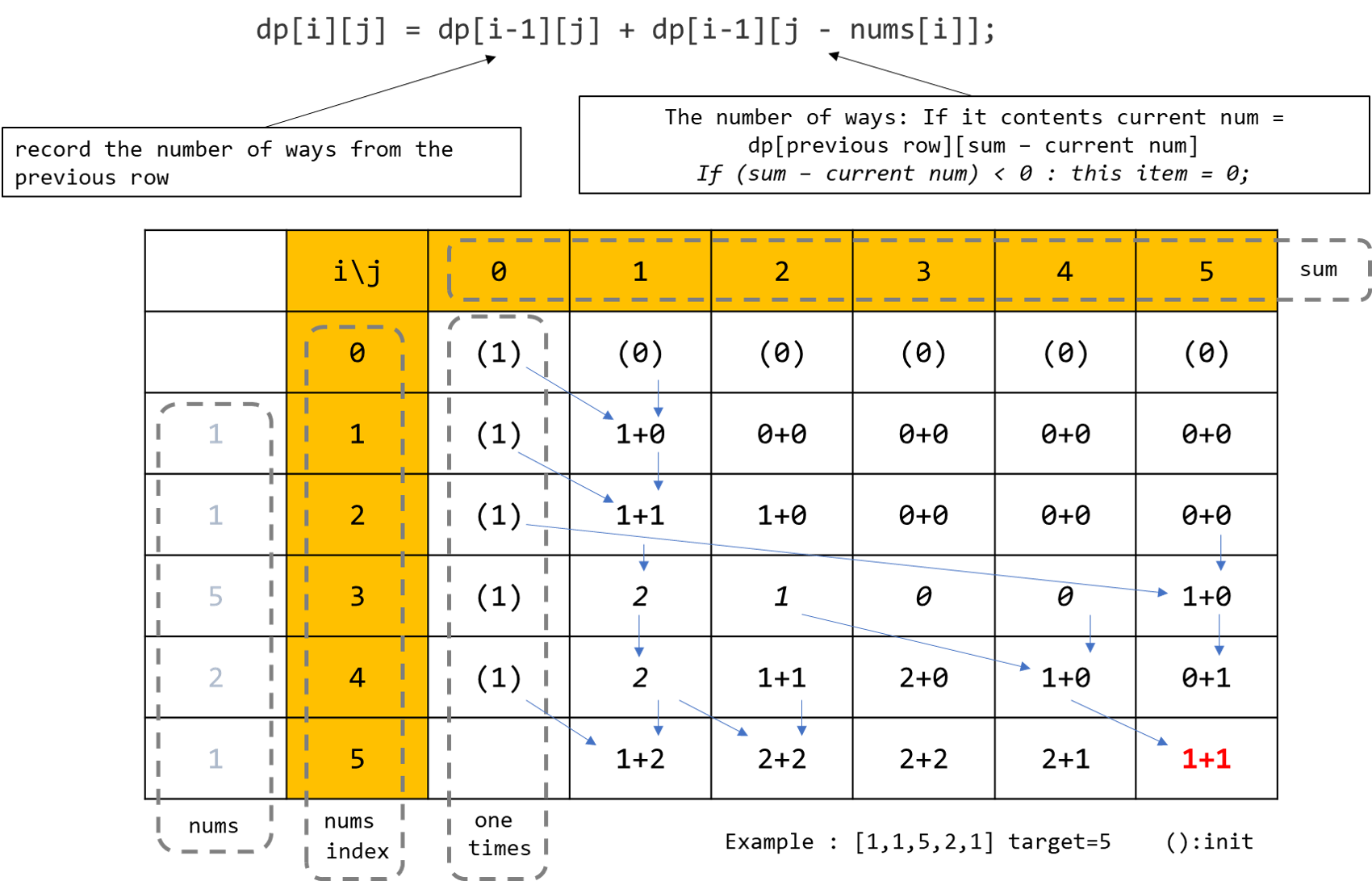
原理,如下图所示(原创):