Introduction of Binary Signal Transmission
In a binary communication system, binary data consisting of a sequence of O's and
l's are transmitted by means of two signal waveforms, say, So(t) and S1 (t). Suppose
that the data rate is specified as R bits per second. Then each bit is mapped into a
corresponding signal waveform according to the rule,

where Tb = 1 / R is defined as the bit time interval. We assume that the data bits 0
and 1 are equally probable-that is, each occurs with probability 1/2 - and are mutually
statistically independent.
The channel through which the signal is transmitted is assumed to corrupt the signal
by the addition of noise, denoted as n ( t), which is a sample function of a white
Gaussian process with power spectrum No/2 watts/hertz. Such a channel is called an
additive white Gaussian noise (AWGN) channel. Consequently, the received signal
waveform is expressed as,
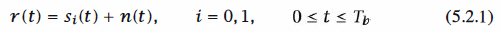
The task of the receiver is to determine whether a 0 or a 1 was transmitted after
observing the received signal r(t) in the interval 0 <= t <= Tb. The receiver is designed
to minimize the probability of error. Such a receiver is called the optimum receiver.
Reference,
1. <<Contemporary Communication System using MATLAB>> - John G. Proakis