[并查集] D. 0-1-Tree

You are given a tree (an undirected connected acyclic graph) consisting of nn vertices and n−1n−1 edges. A number is written on each edge, each number is either 00 (let's call such edges 00-edges) or 11 (those are 11-edges).

Let's call an ordered pair of vertices (x,y)(x,y) (x≠yx≠y) valid if, while traversing the simple path from xx to yy, we never go through a 00-edge after going through a 11-edge. Your task is to calculate the number of valid pairs in the tree.

Input

The first line contains one integer nn (2≤n≤2000002≤n≤200000) — the number of vertices in the tree.

Then n−1n−1 lines follow, each denoting an edge of the tree. Each edge is represented by three integers xixi, yiyi and cici (1≤xi,yi≤n1≤xi,yi≤n, 0≤ci≤10≤ci≤1, xi≠yixi≠yi) — the vertices connected by this edge and the number written on it, respectively.

It is guaranteed that the given edges form a tree.

Output

Print one integer — the number of valid pairs of vertices.

 题意：给定一颗树， 求满足任意两点间先0后1路径条数

---

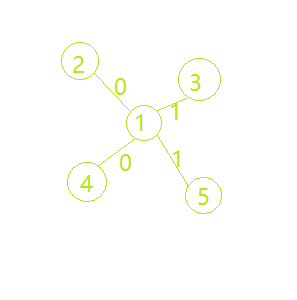
思路：如图所示

以1为当前节点

由树的性质则必有当前节点的1最大联通块为1路径条数 sa （3）

当前节点的0最大联通块为0路径条数 sb （3）

则有通过当前节点且符合题意的路径有 sa * sb - 1(减去当前节点自身的重复统计)

所以用并查集维护节点的最大联通块计算答案即可

---

代码：

/*
    Zeolim - An AC a day keeps the bug away
*/

//#pragma GCC optimize(2)
#include <cstdio>
#include <iostream>
#include <cstdlib>
#include <cmath>
#include <cctype>
#include <string>
#include <cstring>
#include <algorithm>
#include <stack>
#include <queue>
#include <set>
#include <sstream>
#include <map>
#include <ctime>
#include <vector>
#include <fstream>
#include <list>
#include <iomanip>
#include <numeric>
using namespace std;
typedef long long ll;
typedef long double ld;
const int INF = 0x3f3f3f3f;
const ld PI = acos(-1.0);
const ld E = exp(1.0);

const int MAXN = 1e6 + 10;

ll fa[MAXN], fb[MAXN], vla[MAXN], vlb[MAXN];

int findfa(int x)
{
    return x == fa[x] ? x : fa[x] = findfa(fa[x]);
}

int findfb(int x)
{
    return x == fb[x] ? x : fb[x] = findfb(fb[x]);
}

int main()
{
    //ios::sync_with_stdio(false);
    //cin.tie(0);     cout.tie(0);
    //freopen("D://test.in", "r", stdin);
    //freopen("D://test.out", "w", stdout);

    int n;

    cin >> n;

    for(int i = 0; i <= n; ++i)
    {
        fa[i] = fb[i] = i;
        vla[i] = vlb[i] = (ll)1;
    }

    for(int i = 1; i < n; ++i)
    {
        int a, b, val;

        cin >> a >> b >> val;

        if(val == 1)
        {
            int p = findfa(a), q = findfa(b);
            fa[p] = q;
            vla[q] += vla[p];
        }

        else
        {
            int p = findfb(a), q = findfb(b);
            fb[p] = q;
            vlb[q] += vlb[p];
        }
    }

    ll ans = 0;

    for(int i = 1; i <= n; ++i)
    {
    	int p = findfa(i), q = findfb(i);
        ll tsum = vla[p] * vlb[q] - 1ll;
        ans += (tsum);
    }   

    cout << ans << '
';
    
    return 0;
}