blocks(昆明ICPC)

链接：https://ac.nowcoder.com/acm/contest/32708/B

必须得感叹一下这个题真的牛

分析：发现n很小 所以能覆盖完的情况是能枚举出来的 第一个问题就来了

怎么判断状态s能否将整个区域覆盖完全 不难想到求出覆盖面积 合并面积不好算 交面积好算 所以用到容斥原理

面积＝Σ一个矩形面积 - Σ两个矩形相交的面积 + Σ三个矩形相交的面积 - .... 这里的面积都是在指定区域内的面积 如果没相交则面积为0

枚举状态i的所有子集

for (int s = i; s; s = (s - 1) & i)

考虑S的子集，在二进制上从大到小排成一排，那么大的通过减若干个1就一定能到小的，

但是中间会产生大量的状态，这些状态中包含了一些S中不包含的1，故和S&一下，去冗即可，

接下来就是求期望步数

期望倒推 设dp[i]表示当前状态为i 还需多少步才能将所有覆盖

转移有两种

(1)下一步选的已经出现过 cnt/n × dp[i] 其中cnt为状态i中1的个数
(2)下一步选的还未出现过 Σ（1/n × dp[i|1<<j]）

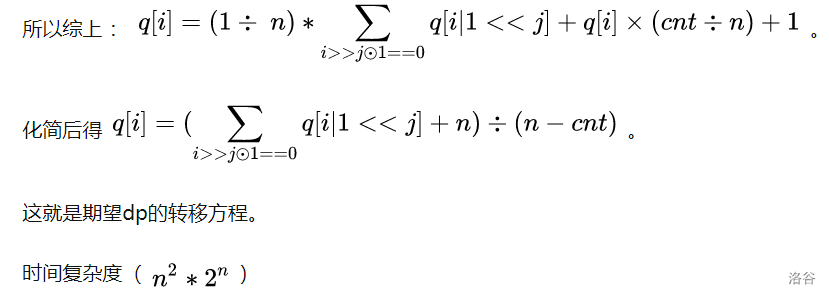
code:

#include <bits/stdc++.h>
using namespace std;
#define ll long long
const int N = 15;
const ll mod = 998244353;
ll n, w, h;
ll x[N], y[N], xx[N], yy[N];
ll dp[1 << 11 + 10], area[1 << 11 + 10], q[1 << 11 + 10];
ll qpow(ll a, ll b)
{
    ll ans = 1;
    while (b)
    {
        if (b & 1)
        {
            ans = ans * a % mod;
        }
        a = a * a % mod;
        b >>= 1;
    }
    return ans;
}
void solve()
{
    cin >> n >> w >> h;
    for (int i = 0; i < n; i++)
    {
        cin >> x[i] >> y[i] >> xx[i] >> yy[i];
    }
    for (int i = 1; i < (1 << n); i++)
    {
        ll x1 = 0, y1 = 0, x2 = w, y2 = h;
        for (int j = 0; j < n; j++)
        {
            if (i >> j & 1)
            {
                x1 = max(x1, x[j]);
                x2 = min(x2, xx[j]);
                y1 = max(y1, y[j]);
                y2 = min(y2, yy[j]);
            }
        }
        area[i] = max(0ll, x2 - x1) * max(0ll, y2 - y1); //枚举上界和下界求矩形面积交
    }
    for (int i = 1; i < (1 << n); i++)
    {
        dp[i] = 0;
        for (int s = i; s; s = (s - 1) & i)
        {
            dp[i] += area[s] * (__builtin_parity(s) ? 1 : -1); //容斥求矩形面积并////__builtin_parity(i)库函数返回二进制中1的个数的奇偶性，奇数返回1，偶数返回0
        }
    }
    ll s = w * h;
    if (dp[(1 << n) - 1] != s) //无法涂满矩阵
    {
        cout << -1 << "\n";
        return;
    }
    for (int i = (1 << n) - 1; i >= 0; i--) //期望dp，式子在上面推过了
    {
        q[i] = 0;
        if (dp[i] == s)
            continue;
        ll s1 = 0;
        for (int j = 0; j < n; j++)
        {
            if (!(i >> j & 1))
            {
                s1 = (s1 + q[i | (1 << j)]) % mod;
            }
        }
        q[i] = (n + s1) * qpow(n - __builtin_popcount(i), mod - 2) % mod; //__builtin_popcount(i)库函数返回二进制中1的个数
    }
    cout << q[0] << "\n";
}
int main()
{
    ll t;
    cin >> t;
    while (t--)
    {
        solve();
    }
}