667. Beautiful Arrangement II

Given two integers n and k, you need to construct a list which contains n different positive integers ranging from 1 to n and obeys the following requirement:
Suppose this list is [a1, a2, a3, ... , an], then the list [|a1 - a2|, |a2 - a3|, |a3 - a4|, ... , |an-1 - an|] has exactly k distinct integers.

If there are multiple answers, print any of them.

Example 1:

Input: n = 3, k = 1
Output: [1, 2, 3]
Explanation: The [1, 2, 3] has three different positive integers ranging from 1 to 3, and the [1, 1] has exactly 1 distinct integer: 1.

Example 2:

Input: n = 3, k = 2
Output: [1, 3, 2]
Explanation: The [1, 3, 2] has three different positive integers ranging from 1 to 3, and the [2, 1] has exactly 2 distinct integers: 1 and 2.

Note:

1. The n and k are in the range 1 <= k < n <= 104.

class Solution {
    public int[] constructArray(int n, int k) {
        int l = 1, r = n;
        int[] res = new int[n];
        for(int i = 0; i < n; i++) {
            res[i] = (k % 2 == 0 ? l++ : r--);
            if(k > 1) k--;
        }
        return res;
    }
}

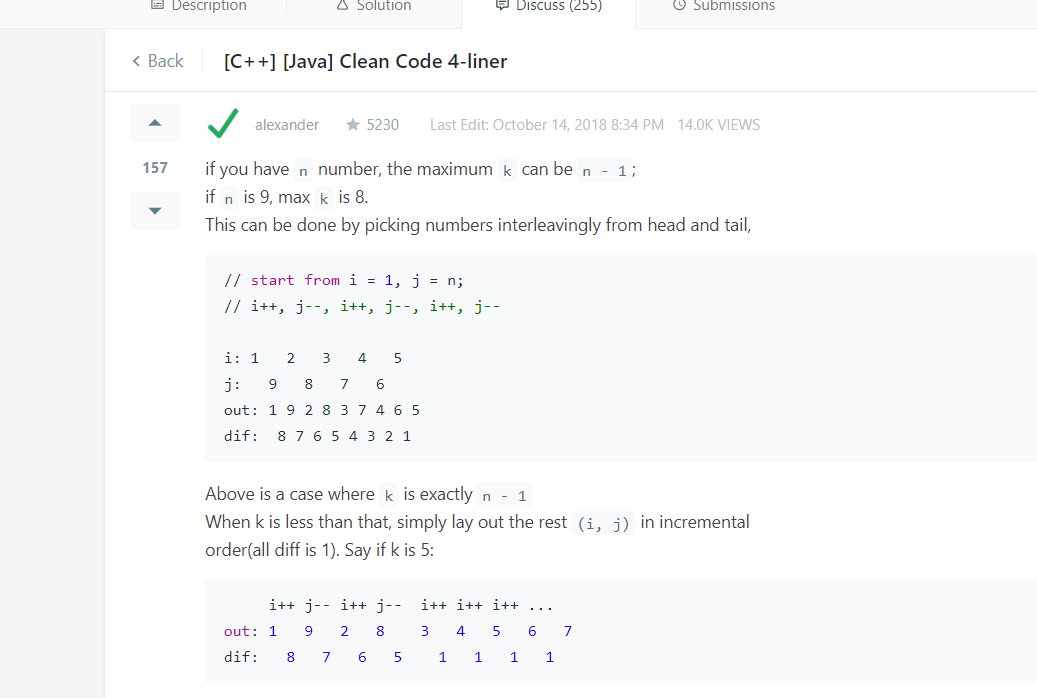
题目要求个permutation从1-n，要求总共要k个不同的相邻两数的diff。

这样想，n个数，总共最多有n-1个diff，how？

1,2,3,4,5的话，n = 4

1,5,2,4,3，diff = 4,3,2,1

所以，如果要k个不同的，到k-1个之后，让所有剩下的数in order排列，这样最后一个diff就是1了。

这样，通过判断k是odd还是even来给每个位置赋值，如果k还不等于1，那就一前一后这样分开选择，等于1就随便选一边把剩下的补齐。

神奇。。