Given the root of a binary tree and an integer distance. A pair of two different leaf nodes of a binary tree is said to be good if the length of the shortest path between them is less than or equal to distance.
Return the number of good leaf node pairs in the tree.
Example 1:
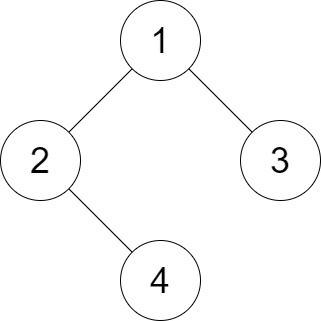
Input: root = [1,2,3,null,4], distance = 3 Output: 1 Explanation: The leaf nodes of the tree are 3 and 4 and the length of the shortest path between them is 3. This is the only good pair.
Example 2:
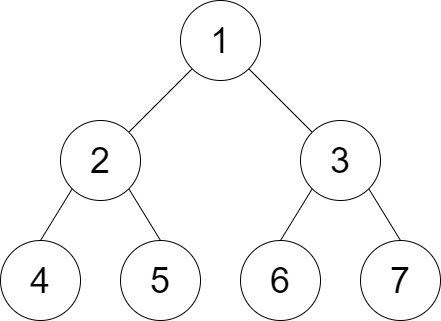
Input: root = [1,2,3,4,5,6,7], distance = 3 Output: 2 Explanation: The good pairs are [4,5] and [6,7] with shortest path = 2. The pair [4,6] is not good because the length of ther shortest path between them is 4.
Example 3:
Input: root = [7,1,4,6,null,5,3,null,null,null,null,null,2], distance = 3 Output: 1 Explanation: The only good pair is [2,5].
Example 4:
Input: root = [100], distance = 1 Output: 0
Example 5:
Input: root = [1,1,1], distance = 2 Output: 1
Constraints:
- The number of nodes in the
treeis in the range[1, 2^10]. - Each node's value is between
[1, 100]. 1 <= distance <= 10
class Solution { private int res; public int countPairs(TreeNode root, int distance) { res = 0; helper(root, distance); return res; } private int[] helper(TreeNode node, int distance) { if (node == null) return new int[11]; int[] left = helper(node.left, distance); int[] right = helper(node.right, distance); int[] A = new int[11]; // node is leaf node, no child, just return if (node.left == null && node.right == null) { A[1] = 1; return A; } // find all nodes satisfying distance for (int i = 0; i <= 10; ++i) { for (int j = 0; j <= 10; ++j) { if (i + j <= distance) res += (left[i] * right[j]); } } // increment all by 1, ignore the node distance larger than 10 for (int i = 0; i <= 9; ++i) { A[i + 1] += left[i]; A[i + 1] += right[i]; } return A; } }
