1,栈的定义
栈:先进后出的数据结构,如下图所示,先进去的数据在底部,最后取出,后进去的数据在顶部,最先被取出。

栈常用操作:
s=Stack() 创建栈
s.push(item) 将数据item放在栈的顶部
s.pop() 返回栈顶部数据,并从栈中移除该数据
s.peek() 返回栈顶部数据,但不移除
s.size() 返回栈的大小
s.isEmpty() 返回栈是否为空
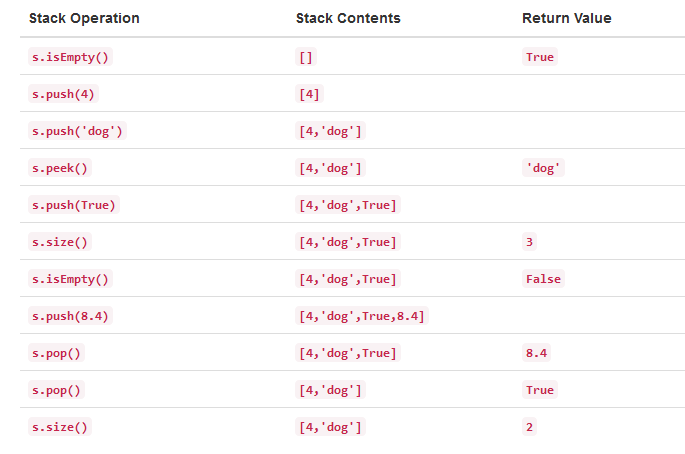
操作示例:
2,用python实现栈
通过python的list来实现栈,其定义如下面代码所示。其中入栈和出栈操作也可以用insert(0,item)和pop(0),但其时间复杂度为O(n); 而append(item)和pop()时间复杂度为O(1)
class Stack(object):
def __init__(self): self.items = [] def push(self,item): self.items.append(item) def pop(self): return self.items.pop() def peek(self): return self.items[-1] def size(self): return len(self.items) def isEmpty(self): return self.items==[]
3, 栈的应用
3.1 判断单个括号是否平衡:如下图中的左括号和右括号是否依次匹配


利用栈作为数据结构,左括号时入栈,右括号时出栈,相应的代码如下:
from stackDemo import Stack parentheses = ['((((((())','()))','(()()(()','()()()','(()()'] def check_balence(pString): s = Stack() for i in range(len(pString)): if pString[i]=='(': s.push(i) else: if not s.isEmpty(): s.pop() else: return False return s.isEmpty() for pt in parentheses: print check_balence(pt)
3.2 判断多种括号是否平衡:{ [ ( 和 ) ] }应依次匹配
平衡示例:

不平衡示例:

实现代码如下:
from stackDemo import Stack def check_balance(sym_string): s= Stack() for i in range(len(sym_string)): symbol = sym_string[i] if symbol in '{[(': s.push(symbol) else: if not s.isEmpty(): top = s.pop() if not match(top,symbol): return False else: return False return s.isEmpty() def match(open,close): opens = '{[(' closes = '}])' return opens.index(open)==closes.index(close)
symbols = ['{ { ( [ ] [ ] ) } ( ) }','[ [ { { ( ( ) ) } } ] ]','[ ] [ ] [ ] ( ) { }',
'( [ ) ]','( ( ( ) ] ) )','[ { ( ) ]']
for sym_string in symbols:
sym_string = sym_string.replace(' ','')
print check_balance(sym_string)
3.3. 将十进制数转化为二进制数
过程:将十进制数不断除2,将余数入栈,最后再一次弹出。
代码实现如下:
from stackDemo import Stack def divideBy2(decNumber): s= Stack() while decNumber>0: remainder = decNumber%2 s.push(remainder) decNumber = decNumber//2 binStr = '' while not s.isEmpty(): binStr = binStr + str(s.pop()) return binStr print divideBy2(8)
3.4. 将十进制数转化为二进制,八进制和十六进制数
(修改代码便可以转换十进制数为任何base的数字)
from stackDemo import Stack
def baseConvetor(decNumber, base): s= Stack() while decNumber>0: remainder = decNumber%base s.push(remainder) decNumber = decNumber//base digits = '0123456789ABCDEF' binStr = '' while not s.isEmpty(): binStr = binStr + digits[s.pop()] if base==8: return "0"+binStr elif base==16: return "0x"+binStr else: return binStr print baseConvetor(30,2) print baseConvetor(30,8) print baseConvetor(30,16)
3.5 算术表达式的转换
一般的算术表达式顺序为Infix Expression,如下表所示,这种形式便于人类理解其执行顺序,但对于电脑,Prefix Expression 和 Postfix Expression 两种形式的表达式更容易理解。需要一个算法程序来完成Infix Expression 到 Prefix Expression 和 Postfix Expression的转换。

Infix Expression 到 Postfix Expression的转换过程:
1,新建一个stack来存放运算符,一个list来存放输出结果
2,对infix expression进行遍历,
当碰到运算数时将其加入到list末尾
当碰到左括号时,将其入栈,碰到右括号时,将栈中的内容依次移出并添加到list末尾,直到碰到相应的左括号停止
当碰到运算符+ - * /时,将其入栈stack,但若栈中有优先度比其高或相同的运算符,先将其移出并添加到list末尾
3,遍历完成后,将栈中剩余内容依次弹出,添加到list末尾
代码实现如下:
def infixtopostfix(infix): prec={'(':1,'+':2, '-':2, '*':3, '/':3} infix_list = infix.split() s=Stack() postfix_list=[] for item in infix_list: if item in "ABCDEFGHIJKLMNOPQRSTUVWXYZ" or item in "0123456789": postfix_list.append(item) elif item=='(': s.push(item) elif item==')': top = s.pop() while top!='(': postfix_list.append(top) top = s.pop() else: while not s.isEmpty() and (prec[item]<=prec[s.peek()]): top = s.pop() postfix_list.append(top) s.push(item) while not s.isEmpty(): postfix_list.append(s.pop()) return ' '.join(postfix_list) print infixtopostfix("A * B + C * D") print infixtopostfix("( A + B ) * C - ( D - E ) * ( F + G )") print infixtopostfix("( A + B ) * C") print infixtopostfix("( A + B ) * ( C + D )")
Postfix Expression的计算过程:
1,创建一个stack来存放运算数
2,遍历Postfix Expression
当碰到运算数时,将其入栈
当碰到运算符时,出栈两次,若第一次出栈为a,第二次出栈为b,计算 (b 运算符 a),并将结果入栈
3,遍历完成后,最终的计算结果在栈顶
代码实现如下:
def postfixEval(postfix): s = Stack() postfix_list = postfix.split() for token in postfix_list: if token in '0123456789': s.push(int(token)) else: operand2 = s.pop() operand1 = s.pop() result = doMath(operand1,operand2,token) s.push(result) return s.pop() def doMath(operand1,operand2,token): if token=='*': return operand1 * operand2 elif token=='/': return operand1 / operand2 elif token=='+': return operand1 + operand2 else: return operand1 - operand2 print postfixEval('5 4 + 8 * 3 2 - 4 2 + * -') print postfixEval('6 5 + 4 *')
Infix Expression 到 Prefix Expression: 将Infix Expression 翻转,左右括号互换,然后按infixtopostfix转换,最后再进行翻转?
过程示例: "( A + B ) * C" — " C * ( B + A )"—"C B A + * "—" * + A B C"
参考:http://interactivepython.org/runestone/static/pythonds/BasicDS/WhatisaStack.html