HDOJ_ACM_折线分割平面

Problem Description

我们看到过很多直线分割平面的题目，今天的这个题目稍微有些变化，我们要求的是n条折线分割平面的最大数目。比如，一条折线可以将平面分成两部分，两条折线最多可以将平面分成7部分，具体如下所示。

 

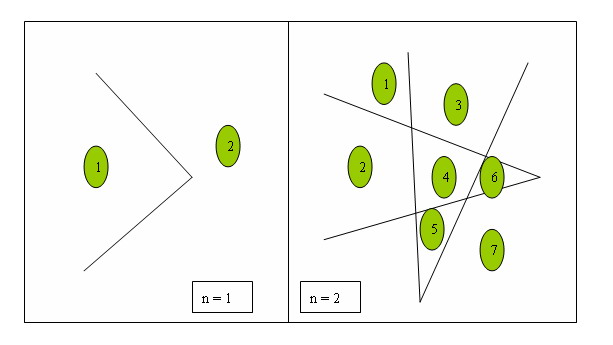Input

输入数据的第一行是一个整数C,表示测试实例的个数，然后是C 行数据，每行包含一个整数n(0<n<=10000),表示折线的数量。

 

Output

            对于每个测试实例，请输出平面的最大分割数，每个实例的输出占一行。

 

Sample Input

2
1
2

 

Sample Output

2
7

 

Code

Key Points

Fristly, it's the question which need inference.

If it's straight line, it's easy for you to find out that f(n)=f(n-1)+(n-1)+1.

Because when you add other straight line, it will increase n - 1 points, it means the number of surface will increase n.

The same to broken line.

When you add other broken line, it will add no more than 4(n-1) points, then the number of surface will increase 4(n-1)+1.

Consequently, you will get the formula: f(n)=f(n-1)+4n-3.

Secondly, according the formula, you can get another formula f(n)=2n^2-n+1 to simplify the code.