loj 6035 「雅礼集训 2017 Day4」洗衣服

分析

神仙贪心题

题解

首先我们用t[i]存下第i件洗完的衣服所用的最短时间，

这个直接用优先队列贪心就好

先把洗衣机的时间按从小到大排序

然后挨个按结束时间贪心

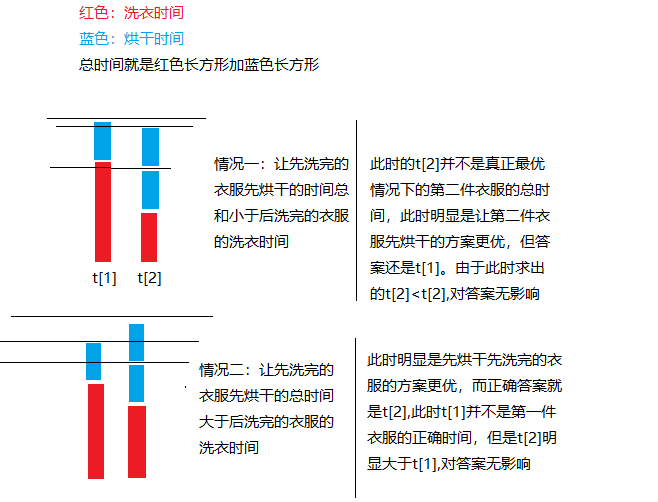
烘干机的贪心就是尽量把后洗完的配给时间更短的烘干机

使等待的时间最小化

等待的时间可以重叠，但是同一台烘干机的烘干时间是不能重叠的

如果一台烘干机要工作两次，那么尽量让这两次中间不等待，所以：

此时的t[i]更新为总时间

下面会解释用同一台烘干机的情况，不同烘干机互不影响，直接贪心就好

代码

/*****************************
User:Mandy.H.Y
Language:c++
Problem:laundry
*****************************/
//君之清极，当宿花丛，睡云端，不知寒 
#include<bits/stdc++.h>
#define Max(x,y) ((x) > (y) ? (x) : (y))
#define Min(x,y) ((x) < (y) ? (x) : (y))

using namespace std;

const int maxn = 1e6 + 5;

int l,n,m;
long long w[maxn],d[maxn],ans;
long long t[maxn],t2[maxn];
long long cntw[maxn],cntd[maxn];

struct Node{
    long long t,id;
    Node(long long _t=0,long long _id=0):t(_t),id(_id){}
    bool operator <(const Node &a)const {
        return t > a.t;
    }
};

priority_queue<Node>q1,q2,q3;

template<class T>inline void read(T &x){
    x = 0;bool flag = 0;char ch = getchar();
    while(!isdigit(ch)) flag |= ch == '-',ch = getchar();
    while(isdigit(ch)) x = (x << 1) + (x << 3) + (ch ^ 48),ch = getchar();
    if(flag) x = -x;
}

template<class T>void putch(const T x){
    if(x > 9) putch(x / 10);
    putchar(x % 10 | 48);
}

template<class T>void put(const T x){
    if(x < 0) putchar('-'),putch(-x);
    else putch(x);
}

void file(){
    freopen("laundry.in","r",stdin);
    freopen("laundry.out","w",stdout);
}

void readdata(){
    read(l);read(n);read(m);
    for(int i = 1;i <= n; ++ i) read(w[i]); 
    for(int i = 1;i <= m; ++ i) read(d[i]); 
    sort(w + 1,w + n + 1);
    sort(d + 1,d + m + 1);

}

void work1(){
    put(w[1] + d[1]);
}

void work(){
    long long cn=1,cm=1;
    q1.push(Node(w[1],cn));
    for(int i = 1;i <= l; ++ i){
        
        Node x = q1.top();
        q1.pop();
        t[i] = x.t;
        q1.push(Node(x.t + w[x.id],x.id));
        if(x.id == cn){
            cn++;
            if(cn <= n)q1.push(Node(w[cn],cn)); 
        } 
    }
    q2.push(Node(d[cm],cm));
    for(int i = l;i >= 1; -- i){
        Node x = q2.top();
        q2.pop();
        if(x.id == cm) {    
            cm++;
            if(cm <= m)q2.push(Node(d[cm],cm));
        }
        t[i] += x.t;
        //这里的t[i]不一定就是真实的洗衣时间，但对答案无影响 
        //最小化等待时间 
        x.t += d[x.id];
        q2.push(x);
        ans = max(ans,t[i]); 
    }
    put(ans);
}

int main(){
//    file();
    readdata();
    if(l == 1) work1();
    else work();
    return 0;
}