平衡二叉树详解
简介
平衡二叉树(Balanced Binary Tree)具有以下性质:它是一棵空树或它的左右两个子树的高度差的绝对值不超过1,并且左右两个子树都是一棵平衡二叉树。平衡二叉树的常用实现方法有红黑树、AVL、替罪羊树、Treap、伸展树等。 其中最为经典当属AVL树,我们
总计而言就是:平衡二叉树是一种二叉排序树,其中每一个结点的左子树和右子树的高度差至多等于1。
性值
AVL树具有下列性质的二叉树(注意,空树也属于一种平衡二叉树):
l 它必须是一颗二叉查找树
l 它的左子树和右子树都是平衡二叉树,且左子树和右子树的深度之差的绝对值不超过1。
l 若将二叉树节点的平衡因子BF定义为该节点的左子树的深度减去它的右子树的深度,则平衡二叉树上所有节点的平衡因子只可能为-1,0,1.
l 只要二叉树上有一个节点的平衡因子的绝对值大于1,那么这颗平衡二叉树就失去了平衡。
实现
平衡二叉树不平衡的情形:
把需要重新平衡的结点叫做α,由于任意两个结点最多只有两个儿子,因此高度不平衡时,α结点的两颗子树的高度相差2.容易看出,这种不平衡可能出现在下面4中情况中:
1.对α的左儿子的左子树进行一次插入
2.对α的左儿子的右子树进行一次插入
3.对α的右儿子的左子树进行一次插入
4.对α的右儿子的右子树进行一次插入

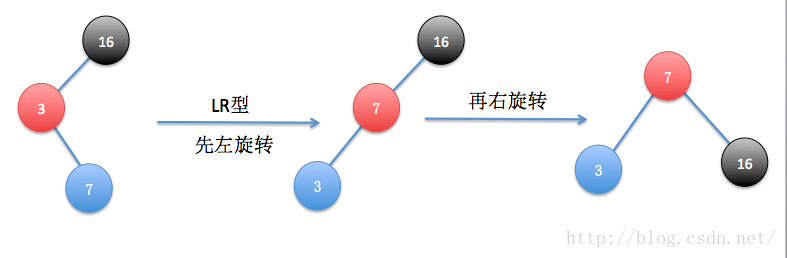
(1)LR型
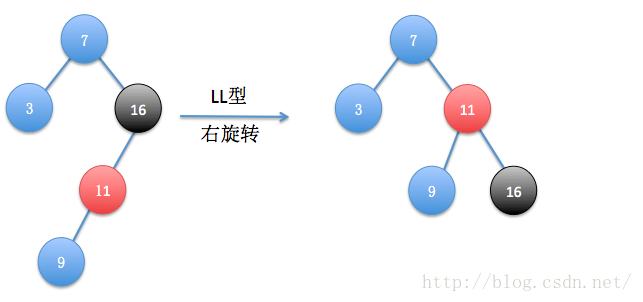
(2)LL型
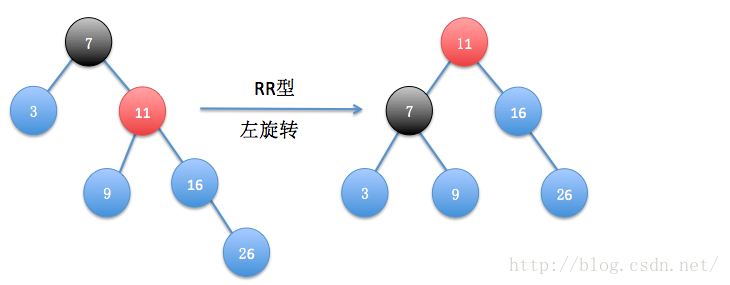
(3)RR型
(4)RL型
完整代码
#include<stdio.h>
#include<stdlib.h>
//结点设计
typedef struct Node {
int key;
struct Node *left;
struct Node *right;
int height;
} BTNode;
int height(struct Node *N) {
if (N == NULL)
return 0;
return N->height;
}
int max(int a, int b) {
return (a > b) ? a : b;
}
BTNode* newNode(int key) {
struct Node* node = (BTNode*)malloc(sizeof(struct Node));
node->key = key;
node->left = NULL;
node->right = NULL;
node->height = 1;
return(node);
}
//ll型调整
BTNode* ll_rotate(BTNode* y) {
BTNode *x = y->left;
y->left = x->right;
x->right = y;
y->height = max(height(y->left), height(y->right)) + 1;
x->height = max(height(x->left), height(x->right)) + 1;
return x;
}
//rr型调整
BTNode* rr_rotate(BTNode* y) {
BTNode *x = y->right;
y->right = x->left;
x->left = y;
y->height = max(height(y->left), height(y->right)) + 1;
x->height = max(height(x->left), height(x->right)) + 1;
return x;
}
//判断平衡
int getBalance(BTNode* N) {
if (N == NULL)
return 0;
return height(N->left) - height(N->right);
}
//插入结点&数据
BTNode* insert(BTNode* node, int key) {
if (node == NULL)
return newNode(key);
if (key < node->key)
node->left = insert(node->left, key);
else if (key > node->key)
node->right = insert(node->right, key);
else
return node;
node->height = 1 + max(height(node->left), height(node->right));
int balance = getBalance(node);
if (balance > 1 && key < node->left->key) //LL型
return ll_rotate(node);
if (balance < -1 && key > node->right->key) //RR型
return rr_rotate(node);
if (balance > 1 && key > node->left->key) { //LR型
node->left = rr_rotate(node->left);
return ll_rotate(node);
}
if (balance < -1 && key < node->right->key) { //RL型
node->right = ll_rotate(node->right);
return rr_rotate(node);
}
return node;
}
//遍历
void preOrder(struct Node *root) {
if (root != NULL) {
printf("%d ", root->key);
preOrder(root->left);
preOrder(root->right);
}
}
int main() {
BTNode *root = NULL;
root = insert(root, 2);
root = insert(root, 1);
root = insert(root, 0);
root = insert(root, 3);
root = insert(root, 4);
root = insert(root, 4);
root = insert(root, 5);
root = insert(root, 6);
root = insert(root, 9);
root = insert(root, 8);
root = insert(root, 7);
printf("前序遍历:");
preOrder(root);
return 0;
}