算法复习——1D/1Ddp优化

搬讲义~~~~

题目1：玩具装箱(bzoj1010)

Description

P教授要去看奥运，但是他舍不下他的玩具，于是他决定把所有的玩具运到北京。他使用自己的压缩器进行压缩，其可以将任意物品变成一堆，再放到一种特殊的一维容器中。P教授有编号为1…N的N件玩具，第i件玩具经过压缩后变成一维长度为Ci.为了方便整理，P教授要求在一个一维容器中的玩具编号是连续的。同时如果一个一维容器中有多个玩具，那么两件玩具之间要加入一个单位长度的填充物，形式地说如果将第i件玩具到第j个玩具放到一个容器中，那么容器的长度将为 x=j-i+Sigma(Ck) i<=K<=j 制作容器的费用与容器的长度有关，根据教授研究，如果容器长度为x,其制作费用为(X-L)^2.其中L是一个常量。P教授不关心容器的数目，他可以制作出任意长度的容器，甚至超过L。但他希望费用最小.

Input

第一行输入两个整数N，L.接下来N行输入Ci.1<=N<=50000,1<=L,Ci<=10^7

Output

输出最小费用

Sample Input

5 4
3
4
2
1
4

Sample Output

经典的决策单调性问题··但也可以用斜率优化····维护一个栈，栈中维护每个决策以及用到它的最优状态的左右区间··每次先更新状态然后将其作为决策二分查找替换栈中决策即可···

另外由于决策单调性是建立在平行四边形不等式的基础上的··而那个东西证起来很麻烦····做题的时候也不容易反应过来··所以尽量还是打表来寻找决策单调性··

代码：

#include<iostream>
#include<cstdio>
#include<cstdlib>
#include<cmath>
#include<ctime>
#include<cctype>
#include<cstring>
#include<string>
#include<algorithm>
using namespace std;
const int N=5e4+5;
long long sum[N],f[N];
struct node
{
  int l,r,pos;
}que[N];
int n,L,head,tail;
inline int R()
{
  char c;int f=0;
  for(c=getchar();c<'0'||c>'9';c=getchar());
  for(;c<='9'&&c>='0';c=getchar())  f=(f<<3)+(f<<1)+c-'0';
  return f;
}
inline long long get(int j,int i)
{
  return f[j]+(long long)(sum[i]-sum[j]+i-j-1-L)*(sum[i]-sum[j]+i-j-1-L);
}
inline int find(node a,int b)
{
  int le=a.l,ri=a.r;
  while(le<=ri)
  {
    int mid=(le+ri)/2;
    if(get(b,mid)<get(a.pos,mid)) ri=mid-1;  
    else le=mid+1;
  }
  return le;
}
inline void work()
{
  head=1,tail=0;
  node temp;temp.l=0,temp.r=n,temp.pos=0;que[++tail]=temp;
  for(int i=1;i<=n;i++)
  {
    while(que[head].r<i)  head++; 
    f[i]=get(que[head].pos,i);
    if(head>tail||get(i,n)<get(que[tail].pos,n))
    {
      while(head<=tail&&(get(i,que[tail].l)<get(que[tail].pos,que[tail].l))) tail--;
      if(head<=tail)
      {
        int t=find(que[tail],i);
        que[tail].r=t-1;
        node temp;temp.l=t;temp.r=n;temp.pos=i;
        que[++tail]=temp;
      }
      else 
      {
        node temp;temp.l=i,temp.r=n,temp.pos=i;
        que[++tail]=temp; 
      }
    }
  }
}
int main()
{
  //freopen("a.in","r",stdin);
  n=R();L=R();  
  for(int i=1;i<=n;i++)  sum[i]=R(),sum[i]+=sum[i-1];
  work();cout<<f[n]<<endl;
  return 0;
}

题目2：土地购买（bzoj1597）

Description

农夫John准备扩大他的农场,他正在考虑N (1 <= N <= 50,000) 块长方形的土地. 每块土地的长宽满足(1 <= 宽 <= 1,000,000; 1 <= 长 <= 1,000,000). 每块土地的价格是它的面积,但FJ可以同时购买多快土地. 这些土地的价格是它们最大的长乘以它们最大的宽, 但是土地的长宽不能交换. 如果FJ买一块3x5的地和一块5x3的地,则他需要付5x5=25. FJ希望买下所有的土地,但是他发现分组来买这些土地可以节省经费. 他需要你帮助他找到最小的经费.

Input

* 第1行: 一个数: N

* 第2..N+1行: 第i+1行包含两个数,分别为第i块土地的长和宽

Output

* 第一行: 最小的可行费用.

Sample Input

4
100 1
15 15
20 5
1 100

输入解释:

共有4块土地.

Sample Output

500

HINT

FJ分3组买这些土地: 第一组:100x1, 第二组1x100, 第三组20x5 和 15x15 plot. 每组的价格分别为100,100,300, 总共500.

这道题首先将土地按x降序排序···可以容易发现那些x和y都会小于某一个土地的土地是肯定是多余无用的···所以将这些点排除后剩余土地肯定是按照x降序··y升序排列的，于是可以推出dp方程

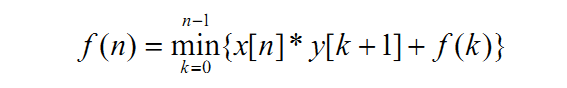
其中f[n]表示的是购买前n块土地的最小花费···方程满足单调性····于是就和上面一道题一样了······其实这道题也可以用斜率优化来做·····后面会提到

#include<iostream>
#include<cstdio>
#include<cstdlib>
#include<cmath>
#include<ctime>
#include<cctype>
#include<cstring>
#include<string>
#include<algorithm>
using namespace std;
const int N=5e4+5;
struct node
{
  int x,y;
}a[N],b[N];
struct node1
{
  int l,r,pos;
}que[N];
inline bool cmp(node a,node b)
{
  if(a.x==b.x)  return a.y>b.y; 
  return a.x>b.x;
}
inline int R()
{
  char c;int f=0;
  for(c=getchar();c<'0'||c>'9';c=getchar());
  for(;c<='9'&&c>='0';c=getchar())  f=(f<<3)+(f<<1)+c-'0';
  return f;
}
int n,tot;
long long f[N];
inline long long calc(int i,int j)
{
  return f[j]+(long long)b[i].y*b[j+1].x;
}
inline int find(node1 a,int b)
{
  int le=a.l,ri=a.r,ans=a.r+1;
  while(le<=ri)
  {
    int mid=(le+ri)/2;
    if(calc(mid,b)<calc(mid,a.pos))  ri=mid-1,ans=mid;
    else le=mid+1;
  }
  return ans;
}
inline bool dp()
{
  int head=1,tail=0;
  node1 temp;temp.l=0;temp.r=tot;temp.pos=0;que[++tail]=temp;
  for(int i=1;i<=tot;i++)
  {
    while(que[head].r<i)  head++;
    f[i]=calc(i,que[head].pos);
    if(calc(tot,i)<calc(tot,que[tail].pos))
    {
      while(head<=tail&&calc(que[tail].l,i)<calc(que[tail].l,que[tail].pos))  tail--;
      if(head<=tail)
      {
        int t=find(que[tail],i);
        que[tail].r=t-1;
        node1 temp;temp.l=t,temp.r=tot,temp.pos=i;que[++tail]=temp;
      }
      else
      {
        node1 temp;temp.l=i;temp.r=tot;temp.pos=i;que[++tail]=temp;
      }
    }
  }
}
int main()
{
 // freopen("a.in","r",stdin);
  n=R();
  for(int i=1;i<=n;i++)
    a[i].x=R(),a[i].y=R();
  sort(a+1,a+n+1,cmp);b[++tot]=a[1];int maxx=a[1].y;
  for(int i=2;i<=n;i++)
    if(a[i].y>maxx)  maxx=a[i].y,b[++tot]=a[i];  
  dp();cout<<f[tot]<<endl;
  return 0;
}

题目1：生产产品（vijo1243）

描述

在经过一段时间的经营后，dd_engi的OI商店不满足于从别的供货商那里购买产品放上货架，而要开始自己生产产品了！产品的生产需要M个步骤，每一个步骤都可以在N台机器中的任何一台完成，但生产的步骤必须严格按顺序执行。由于这N台机器的性能不同，它们完成每一个步骤的所需时间也不同。机器i完成第j个步骤的时间为T[i,j]。把半成品从一台机器上搬到另一台机器上也需要一定的时间K。同时，为了保证安全和产品的质量，每台机器最多只能连续完成产品的L个步骤。也就是说，如果有一台机器连续完成了产品的L个步骤，下一个步骤就必须换一台机器来完成。现在，dd_engi的OI商店有史以来的第一个产品就要开始生产了，那么最短需要多长时间呢？
某日Azuki.7对跃动说:这样的题目太简单,我们把题目的范围改一改
对于菜鸟跃动来说,这是个很困难的问题,他希望你能帮他解决这个问题

格式

输入格式

第一行有四个整数M, N, K, L
下面的N行，每行有M个整数。第I+1行的第J个整数为T[J,I]。

输出格式

输出只有一行，表示需要的最短时间。

样例1

样例输入1

3 2 0 2
2 2 3
1 3 1

样例输出1

限制

提示

对于50%的数据，N<=5，L<=4，M<=10000
对于100%的数据，N<=5， L<=50000，M<=100000

来源

第一届“OI商店杯” dd_engi原创题目

很妙的一道单调队列的题···,之前其实是做过的···

首先容易想到朴素的dp方程：f[i][j]表示第i个机器生产了第j个过程且前j个过程已经完成生产的最小花费···容易得到：

dp[i][j]=min{dp[k][j']+sum[i][j]-sum[i][j']}+K

其中sum为预处理出的前缀和···j-l<j'<j

由此我们将sum[i][j]提到外面后我们不难发现大括号号中的部分实际上是关于j'的一个函数····因此可以按照上述步骤用单调队列解决：

代码：

#include<iostream>
#include<cstdio>
#include<cstdlib>
#include<cmath>
#include<ctime>
#include<cctype>
#include<cstring>
#include<string>
#include<algorithm>
#include<deque>
using namespace std;
const int M=1e5+5;
const int N=10;
const int inf=0x7f7f7f7f;
deque<int> dque[N][N];
int n,m,K,sum[M][N],l,dp[M][N];
inline int R()
{
  char c;int f=0;
  for(c=getchar();c<'0'||c>'9';c=getchar());
  for(;c<='9'&&c>='0';c=getchar())
    f=(f<<3)+(f<<1)+c-'0';
  return f;
}
inline int calc(int i,int k,int j)
{
  return dp[k][j]-sum[k][i];
}
int main()
{
  m=R(),n=R(),K=R(),l=R();
  for(int i=1;i<=n;i++)
    for(int j=1;j<=m;j++)
      sum[j][i]=R(),sum[j][i]+=sum[j-1][i];
  memset(dp,inf,sizeof(dp));
  for(int i=1;i<=n;i++) dp[0][i]=0;
  for(int j=0; j<=m; ++j)
    {
        for(int i=1; i<=n; ++i)
            for(int k=1; k<=n; ++k)
                if(i!=k) while(!dque[i][k].empty()&&(j-dque[i][k].front())>l) dque[i][k].pop_front();
        for(int i=1; i<=n; ++i)
            for(int k=1; k<=n; ++k)
                if(i!=k)
                {
                    int a=0,b=0;
                    if(!dque[i][k].empty()) a=calc(i,dque[i][k].front(),k);
                    b=sum[j][i]+K;
                    dp[j][i]=min(dp[j][i],a+b);
                }
        for(int i=1; i<=n; ++i)
            for(int k=1; k<=n; ++k)
                if(i!=k)
                {
                    while(!dque[i][k].empty()&&(calc(i,dque[i][k].back(),k)>=calc(i,j,k))) dque[i][k].pop_back();
                    dque[i][k].push_back(j);
                }
    }
  int ans=inf;
  for(int i=1;i<=n;i++)  ans=min(ans,dp[m][i]);
  cout<<ans-K<<endl;
  return 0;
}

题目1:Max Sum Plus Plus(hdu1024)

Problem Description

Now I think you have got an AC in Ignatius.L's "Max Sum" problem. To be a brave ACMer, we always challenge ourselves to more difficult problems. Now you are faced with a more difficult problem.

Given a consecutive number sequence S₁, S₂, S₃, S₄ ... S_x, ... S_n (1 ≤ x ≤ n ≤ 1,000,000, -32768 ≤ S_x ≤ 32767). We define a function sum(i, j) = S_i + ... + S_j (1 ≤ i ≤ j ≤ n).

Now given an integer m (m > 0), your task is to find m pairs of i and j which make sum(i₁, j₁) + sum(i₂, j₂) + sum(i₃, j₃) + ... + sum(i_m, j_m) maximal (i_x ≤ i_y ≤ j_x or i_x ≤ j_y ≤ j_x is not allowed).

But I`m lazy, I don't want to write a special-judge module, so you don't have to output m pairs of i and j, just output the maximal summation of sum(i_x, j_x)(1 ≤ x ≤ m) instead. ^_^

Input

Each test case will begin with two integers m and n, followed by n integers S₁, S₂, S₃ ... S_n.
Process to the end of file.

Output

Output the maximal summation described above in one line.

Sample Input

1 3 1 2 3 2 6 -1 4 -2 3 -2 3

Sample Output

6 8

Hint

Huge input, scanf and dynamic programming is recommended.

用f[i][j]表示取了i段且第i段最后一个数取的是num[j]时最大的和···推出朴素dp方程：

f[i][j]=max{f[i][j-1]+num[j],f[i-1][k]+num[j]}

其中k小于j·····

不难发现每当我们先枚举i再枚举j时此时的f[i][j-1]只与上一层i-1有关,所以我们可以降维··用f[j]来代表此时的f[i][j]那么可以推出dp方程

f[j]=max(f[j-1]+num[j],temp[j-1]+num[j]);

其中temp为在枚举i-1时已经处理出的上一层的最小值

代码：

#include<iostream>
#include<cstdio>
#include<cstdlib>
#include<cmath>
#include<ctime>
#include<cctype>
#include<string>
#include<cstring>
#include<algorithm>
using namespace std;
const int N=1e6+5;
const int inf=0x3f3f3f3f;
inline int R()
{
  char c;int f=0,i=1;
  for(c=getchar();(c<'0'||c>'9')&&c!='-';c=getchar());
  if(c=='-')  i=-1,c=getchar();
  for(;c<='9'&&c>='0';c=getchar())  f=(f<<3)+(f<<1)+c-'0';  
  return f*i;
}
int num[N],n,m;
long long f[N],temp[N];
int main()
{
  //freopen("a.in","r",stdin);
  while(scanf("%d%d",&m,&n)!=EOF)
  {  
    memset(temp,0,sizeof(temp));
    memset(f,0,sizeof(f));
    for(int i=1;i<=n;i++)  num[i]=R();
    f[0]=temp[0]=0;
    for(int i=1;i<=m;i++)
    { 
      long long maxx=-inf;
      for(int j=i;j<=n;j++)
      {  
        f[j]=max(f[j-1]+num[j],temp[j-1]+num[j]); 
        temp[j-1]=maxx;maxx=max(maxx,f[j]);
      }
    }
    long long ans=-inf;
    for(int i=m;i<=n;i++)  ans=max(ans,f[i]);
    cout<<ans<<endl;
  }
  return 0;
}

题目2：Max Sum Plus Plus Plus

Problem Description

给定一个由n个正整数组成的整数序列

a1 a2 a3 ... an

求按先后次序在其中取m段长度分别为l1、l2、l3...lm的不交叠的连续整数的和的最大值。

Input

第一行是一个整数n(0 ≤ n ≤ 1000)，n = 0表示输入结束
第二行的第一个数是m(1 ≤ m ≤ 20)，
第二行接下来有m个整数l1，l2...lm。
第三行是n个整数a1, a2, a2 ... an.

Output

输出m段整数和的最大值。

Sample Input

3 2 1 1 1 2 3 4 2 1 2 1 2 3 5 0

Sample Output

5 10

和上面一道题几乎是差不多的···只是注意此时f[i][j]表示的是取第i段,第i段的最后一个数字是num[j]时的最大价值··

#include<iostream>
#include<cstdio>
#include<cmath>
#include<ctime>
#include<cstring>
#include<string>
#include<cctype>
#include<cstdlib>
#include<algorithm>
using namespace std;
const int N=1005;
const int M=25;
int numn[N],numm[M],n,m;
long long sumn[N],summ[M],f[N],maxx[N];
inline int R()
{
  char c;int f=0,i=1;
  for(c=getchar();(c<'0'||c>'9')&&c!='-';c=getchar());
  if(c=='-')  i=-1,c=getchar();
  for(;c<='9'&&c>='0';c=getchar())  f=(f<<3)+(f<<1)+c-'0';
  return f*i;
}
int main()
{
  //freopen("a.in","r",stdin);
  while(true)    
  {
    n=R();if(!n) break;m=R();
    memset(sumn,0,sizeof(sumn));memset(summ,0,sizeof(summ));
    memset(f,0,sizeof(f));memset(maxx,0,sizeof(maxx));
    for(int i=1;i<=m;i++)  numm[i]=R(),summ[i]=numm[i]+summ[i-1];
    for(int i=1;i<=n;i++)  numn[i]=R(),sumn[i]=numn[i]+sumn[i-1];
    for(int i=1;i<=m;i++)
    {  
      for(int j=summ[i];j<=n;j++)
      {
        if(j==summ[i])  f[j]=sumn[j];
        else f[j]=maxx[j-numm[i]]+sumn[j]-sumn[j-numm[i]];
      }
      for(int j=summ[i];j<=n;j++)
      {
        if(j==summ[i])  maxx[j]=f[j];
        else maxx[j]=max(maxx[j-1],f[j]);
      }
    }
    long long anss=-1e+18;
    for(int i=summ[m];i<=n;i++)  anss=max(anss,f[i]);
    cout<<anss<<endl;
  }
  return 0;
}

相关阅读:
JAVA访问权限控制[zhuan]
Netstat简介
 查看cpu性能和磁盘空间
 简单linux查询
 linux 三剑客命令(grep,sed ,awk)
同步、异步的使用场景及好处
 AJAX中同步和异步的区别和使用场景
 在SpringBoot中用SpringAOP实现日志记录功能
 springboot使用@Aspect实现AOP记录日志讲解
 Spring：获取容器中的Bean
原文地址：https://www.cnblogs.com/AseanA/p/7654500.html

最新文章
@省选模拟赛03/16
@codechef
@codechef
@总结
 @codeforces
@bzoj
@bzoj
@bzoj
@bzoj
@bzoj