- Overview of Forward Rate Analysis
- INTRODUCTION
- COMPUTATION OF PAR, SPOT AND FORWARD RATES
- MAIN INFLUENCES ON THE YIELD CURVE SHAPE
- USING FORWARD RATE ANALYSIS IN YIELD CURVE TRADES
- APPENDIX A. NOTATION AND DEFINITIONS USED IN THE SERIES UNDERSTANDING THE YIELD CURVE
- APPENDIX B. CALCULATING SPOT AND FORWARD RATES WHEN PAR RATES ARE KNOWN
- APPENDIX C. RELATIONS BETWEEN SPOT RATES, FORWARD RATES, ROLLING YIELDS, AND BOND RETURNS
著:Antti Ilmanen 译:徐瑞龙
Overview of Forward Rate Analysis
远期收益率分析概述
INTRODUCTION
引言
In recent years, advances have been made in the theoretical and the empirical analysis of the term structure of interest rates. However, such analysis is often very quantitative, and it rarely emphasizes practical investment applications. There appears to be a need to bridge the gap between theory and practice and to set up an accessible framework for sophisticated yield curve analysis. This report serves as an overview of a forthcoming series of reports that will examine the theme Understanding the Yield Curve. After briefly describing the computation of par, spot and forward rates, it presents a framework for interpreting the forward rates by identifying their main influences and finally, it develops practical tools for using forward rate analysis in active bond portfolio management. Subsequent reports will discuss these topics in detail.[1]
近年来,有关收益率期限结构的研究在理论和实证分析方面取得了长足进展。然而,这种分析通常是非常数量化的,并且很少强调在实际投资过程中的应用。有必要弥合理论与实践之间的差距,并为复杂的收益率曲线分析建立一个可行的框架。本报告概述了即将出版的一系列报告,报告的主题是“理解收益率曲线”。在简要描述如何计算到期、即期和远期收益率之后,我们提出了一个分析框架,通过识别影响远期收益率的主要因素来解释远期收益率。最后,我们开发了主动债券投资组合管理中远期收益率分析的实用工具。后续报告将详细讨论这些主题。
The three main influences on the Treasury yield curve shape are: (l) the market's expectations of future rate changes; (2) bond risk premia (expected return differentials across bonds of different maturities); and (3) convexity bias. Conceptually, it is easy to divide the yield curve (or the term structure of forward rates) into these three components. It is much harder to interpret real-world yield curve shapes, but the potential benefits are substantial. For example, investors often wonder whether the curve's steepness reflects the market's expectations of rising rates or positive risk premia. The answer to this question determines whether a duration extension increases expected returns. It also shows whether we can view forward rates as the market's expectations of future spot rates. In addition, our analysis will describe how the market's curve reshaping expectations and volatility expectations influence the shape of today's yield curve. These expectations determine the cost of enhancing a portfolio's convexity via a duration-neutral yield curve trade.
影响国债收益率曲线形态的三个主要因素是:(1)市场对未来收益率变化的预期;(2)债券风险溢价(不同期限的债券预期回报之间的差异);(3)凸度偏差。从概念上讲,很容易将收益率曲线(或远期收益率的期限结构)分解为这三个组成部分。解释现实世界中的收益率曲线形状却要困难得多,但同时潜在着巨大的好处。例如,投资者常常想知道曲线的陡峭程度是否反映了市场对收益率上升或正风险溢价的预期。这个问题的答案决定了增加久期是否会增加预期回报。它还说明了我们是否可以将远期收益率视为市场对未来即期收益率的预期。此外,我们的分析将描述市场曲线形态变化的预期和波动率的预期如何影响当下收益率曲线的形状。这些预期决定了在久期中性策略中增强投资组合凸度的成本。
Forward rate analysis also can be valuable in direct applications. Forward rates may be used as break-even rates with which subjective rate forecasts are compared or as relative value tools to identify attractive yield curve sectors. Subsequent reports will analyze many aspects of yield curve trades, such as barbell-bullet trades, and present empirical evidence about their historical behavior.
远期收益率分析在实际应用中也是有价值的。与主观收益率预测进行比较时,远期收益率可以看作是盈亏平衡收益率,或作为相对价值工具,以确定收益率曲线上有吸引力的部分。随后的报告将从多方面分析收益率曲线交易,例如杠铃-子弹交易策略,并提供这些策略的历史表现数据。
COMPUTATION OF PAR, SPOT AND FORWARD RATES
计算到期、即期和远期收益率
At the outset, it is useful to review the concepts "yield to maturity," "par yield," "spot rate," and "forward rate" to ensure that we are using our terms consistently. Appendix A is a reference that describes the notation and definitions of the main concepts used throughout the series Understanding the Yield Curve. Our analysis focuses on government bonds that have known cash flows (no default risk, no embedded options). Yield to maturity is the single discount rate that equates the present value of a bond's cash flows to its market price. A yield curve is a graph of bond yields against their maturities. (Alternatively, bond yields may be plotted against their durations, as we do in many figures in this report.) The best-known yield curve is the on-the-run Treasury curve. On-the-run bonds are the most recently issued government bonds at each maturity sector.
首先,明确“到期收益率”、“票面收益率”、“即期收益率”和“远期收益率”这些概念是有必要的,以确保我们使用一致的术语。附录A汇总了整个《理解收益率曲线》系列中所使用的主要概念的符号和定义。我们的分析专注于现金流已知(没有违约风险、没有嵌入期权)的政府债券。到期收益率是保证债券现金流的现值与市场价格相等的单一贴现率。收益率曲线是债券收益率关于其到期期限的曲线图。(正如本报告中的许多图片表现的那样,债券收益率也可以根据债券久期作图。)最著名的收益率曲线是当期(on-the-run)国债收益率曲线。当期国债是每个相应期限节点最近发行的政府债券(译注:也是交易最活跃的债券之一)。
Because these bonds are always issued with price near par (100), the on-the-run curve often resembles the par yield curve, which is a curve constructed for theoretical bonds whose prices equal par.
因为这些债券总是以接近面值(100)的价格发行,所以当期收益率曲线通常类似于票面收益率曲线,即假设价格等于债券面值而构建出的收益率曲线。
While the yield to maturity is a convenient summary measure of a bond's expected return —— and therefore a popular tool in relative value analysis —— the use of a single rate to discount multiple cash flows can be problematic unless the yield curve is flat. First, all cash flows of a given bond are discounted at the same rate, even if the yield curve slope suggests that different discount rates are appropriate for different cash flow dates. Second, the assumed reinvestment rate of a cash flow paid at a given date can vary across bonds because it depends on the yield of the bond to which the cash flow is attached. This report will show how to analyze the yield curve using simpler building blocks —— single cash flows and one-period discount rates —— than the yield to maturity, an average discount rate of multiple cash flows with various maturities.
虽然到期收益率是债券预期回报的合适度量,也是相对价值分析中的常用工具,但是除非收益率曲线是平的,否则使用单一收益率来贴现多个现金流可能会有问题。首先,给定债券的所有现金流都以相同的贴现率贴现,但是收益率曲线的倾斜表明不同期限的现金流适用不同的贴现率。其次,在给定日期支付的现金流的假设再投资回报率可能因债券而异,因为它取决于现金流对应的债券的收益率。本报告将展示如何使用更简单的模块(单一现金流和一期贴现率)来分析收益率曲线,而不是用到期收益率——不同期限的多个现金流的平均贴现率。
A coupon bond can be viewed as a bundle of zero-coupon bonds (zeros). Thus, it can be unbundled into a set of zeros, which can be valued separately. These zeros then can be rebundled into a more complex bond, whose price should equal the sum of the component prices.[2] The spot rate is the discount rate of a single future cash flow such as a zero. Equation (1) shows the simple relation between an n-year zero's price (P_n) and the annualized n-year spot rate (s_n).
付息债券可以视为一系列零息债券的组合。因此,付息债券可以先拆分为一组零息债券,然后对这些零息债券分别定价。这些零息债券可以重新组成一个更复杂的债券,其价格应要等于各部分价格的总和。即期收益率是未来单个现金流(如零息债券)的贴现率。等式(1)显示了n年期零息债券价格(P_n)和年化的n年期即期收益率(s_n)之间的简单关系。
A single cash flow is easy to analyze, but its discount rate can be unbundled even further to one-period rates. That is, a multiyear spot rate can be decomposed into a product of one-year forward rates, the simplest building blocks in a term structure of interest rates. A given term structure of spot rates implies a specific term structure of forward rates. For example, if the m-year and n-year spot rates are known, the annualized forward rate between maturities m and n, (f_{m,n}) is easily computed from Equation (2).
单一现金流易于分析,其贴现率可以进一步分拆为若干一期贴现率。也就是说,多年期即期收益率可以分解为一系列一年期远期收益率的乘积,一年期远期收益率是即收益率期限结构中最简单的构建模块。给定的即期收益率期限结构隐含着远期收益率的期限结构。例如,如果m年和n年即期收益率是已知的,则m年和n年之间的年化远期收益率(f_{m,n})容易通过等式(2)计算出。
The forward rate is the interest rate for a loan between any two dates in the future, contracted today. Any forward rate can be "locked in" today by buying one unit of the n-year zero at price (P_n = 100/(1 + s_n)^n) and by shortselling (P_n/P_m) units of the m-year zero at price (P_m = 100/(1 + s_m)^m). (Such a weighting requires no net investment today because both the cash inflow and the cash outflow amount to (P_n).) The one-year forward rate ((f_{n-1,n}) such as (f_{2,3}), (f_{3,4}), ...) represents a special case of Equation (2) in which m = n-1. The spot rate represents another special case in which (s_n = f_{0,n}).
远期收益率是指当下确定的未来任何两个日期之间的贷款收益率。任何远期收益率可以通过在价格(P_n = 100/(1 + s_n)^n)下购买一个单位的n年期零息债券,在价格(P_m = 100/(1 + s_m)^m)做空(P_n / P_m)单位的m年期零息债券来“锁定”。这样的组合不需要净投资,因为现金流入和现金流出量等于(P_n)。一年期远期收益率((f_{n-1,n})如(f_{2,3}),(f_{3,4}),...)对应于等式(2)的特殊情况,其中 m = n-1。即期收益率对应另一种特殊情况,(s_n = f_{0,n})
To summarize, a par rate is used to discount at set of cash flows (those of a par bond) to today, a spot rate is used to discount a single future cash flow to today and a forward rate is used to discount a single future cash flow to another (nearer) future date. The par yield curve, the spot rate curve and the forward rate curve contain the same information about today's term structure of interest rates [3] —— if one set of rates is known, it is easy to compute the other sets.[4] Figure 1 shows a hypothetical example of the three curves. In Appendix B, we show how the spot and forward rates were computed based on the par yields.
总而言之,到期收益率用于将一组现金流(债券面值决定的)贴现到现在,即期收益率用于将单个未来现金流贴现到现在,而远期收益率用于将单个未来现金流贴现到另一个(更近的)未来日期。票面收益率曲线、即期收益率曲线和远期收益率曲线包含了关于收益率期限结构的相同信息——如果一组收益率已知,则很容易计算其他收益率。图1显示了一个假设中三条曲线的例子。在附录B中,我们展示了如何基于票面收益率计算即期和远期收益率。
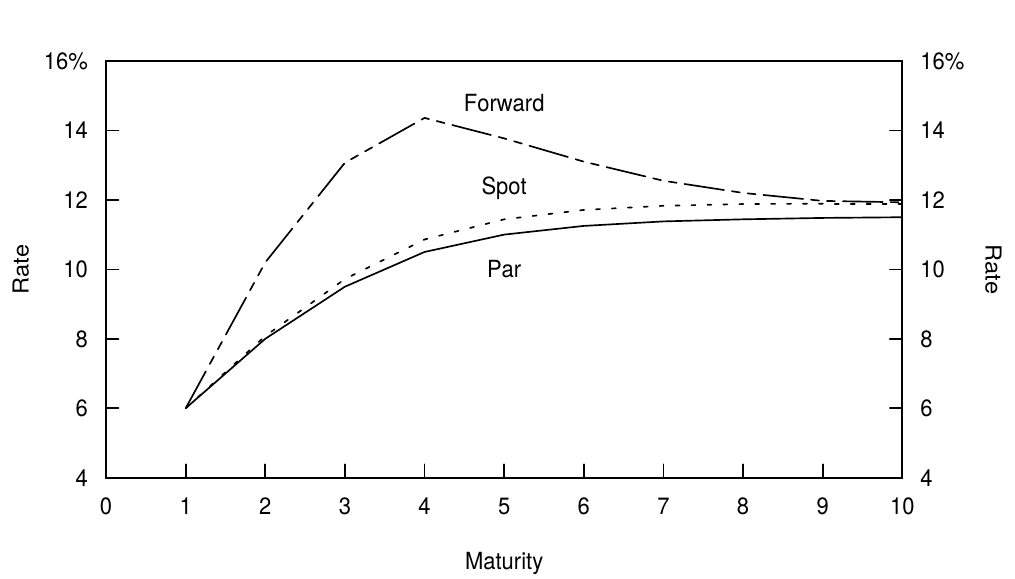
Figure 1. Par, Spot and One-Year Forward Rate Curves
In this example, the par and spot curves are monotonically upward sloping, while the forward rate curve [5] first slopes upward and then inverts (because of the flattening of the spot curve). The spot curve lies above the par curve, and the forward rate curve lies above the spot curve, This is always the case if the spot curve is upward sloping. If it is inverted, the ordering is reversed: The par curve is highest and the forward curve lowest. Thus, loose characterizations of one curve (for example, steeply upward-sloping, flat, inverted, humped) are generally applicable to the other curves. However, the three curves are identical only if they are horizontal. In other cases, the forward rate curve magnifies any variation in the slope of the spot curve. One-year forward rates measure the marginal reward for lengthening the maturity of the investment by one year, while the spot rates measure an investment's average reward from today to maturity n. Therefore, spot rates are (geometric) averages of one or more forward rates. Similarly, par rates are averages of one or more spot rates; thus, par curves have the flattest shape of the three curves. In Appendix C, we discuss further the relationship between spot and forward rate curves.
在这个例子中,票面收益率曲线和即期收益率曲线是单调向上倾斜的,而远期收益率曲线首先向上倾斜,然后向下倾斜(因为即期收益率曲线变平)。即期收益率曲线位于到期收益率曲线上方,并且远期收益率曲线位于即期收益率曲线上方。如果即期收益率向上倾斜,则情况总是如此。如果情况是倒挂的,则顺序是相反的:到期收益率曲线是最高的,远期收益率曲线是最低的。因此,一个曲线不常见的特征(例如,陡峭地向上倾斜、平坦、倒挂或中部隆起)通常也会出现于其他曲线。然而,三条曲线只有在水平时才是相同的。在其他情况下,远期收益率曲线会放大即期收益率曲线斜率出现的任何变化。一年期远期收益率衡量了将投资期限延长一年的边际回报,而即期收益率衡量了投资从当前至到期的平均回报。因此,即期收益率是一个或多个远期收益率的(几何)平均值。类似地,到期收益率是一个或多个即期收益率的平均值。因此,到期收益率曲线是三个曲线中形态最平坦的。在附录C中,我们进一步讨论即期和远期收益率曲线之间的关系。
It is useful to view forward rates as break-even rates. The implied spot rates one year forward ((f_{1,2}), (f_{1,3}), (f_{1,4}), ...) are, by construction, equal to such future spot rates that would make all government bonds earn the same return over the next year as the (riskless) one-year zero. For example, the holding-period return of today's two-year zero (whose rate today is (s_2)) will depend on its selling rate (as a one-year zero) in one year's time. The implied one-year spot rate one year forward ((f_{1,2})) is computed as the selling rate that would make the two-year zero's return (the left-hand side of Equation (3)) equal to the one-year spot rate (the right-hand side of Equation (3)).[6] Formally, Equation (3) is derived from Equation (2) by setting m = 1 and n = 2 and rearranging.
将远期收益率视为盈亏平衡收益率是有用的。如果一年后的即期收益率等于隐含的即期收益率((f_{1,2}), (f_{1,3}), (f_{1,4}), ...),则所有政府债券在接下来的一年的(无风险)回报将和一年期零息债券相同。例如,当前的两年期零息债券(收益率为(s_2))的持有期回报将取决于其一年的卖出收益率(一年期零息债券的收益率)。一年后隐含的一年期即期收益率((f_{1,2}))做为卖出收益率将使两年期零息债券的收益率(公式(3)的左边)等于一年期即期收益率(公式(3)的右边)。形式上,等式(3)通过设置 m = 1 和 n = 2 并重新排列可以从等式(2)导出。
Consider an example using numbers from Figure 1, in which the one-year spot rate ((s_1)) equals 6% and the two-year spot rate ((s_2)) equals 8.08%. Plugging these spot rates into Equation (3), we find that the implied one-year spot rate one year forward ((f_{1,2})) equals 10.20%. If this implied forward rate is exactly realized one year hence, today's two-year zero will be worth (100/1.1020 = 90.74) next year. Today, this zero is worth (100/1.08083^2 = 85.61); thus, its return over the next year would be (90.74/85.61 - 1 = 6\%), exactly the same as today's one-year spot rate. Thus, 10.20% is the break-even level of future one-year spot rate. In other words, the one-year rate has to increase by more than 420 basis points (10.20%-6.00%) before the two-year zero underperforms the one-year zero over the next year. If the one-year rate increases, but by less than 420 basis points, the capital loss of the two-year zero will not fully offset its initial yield advantage over the one-year zero.
考虑使用图1中数字的例子,其中一年期即期收益率((s_1))等于6%,两年期即期收益率((s_2))等于8.08%。将这些即期收益率代入等式(3),我们发现一年后隐含的一年期即期收益率((f_{1,2}))等于10.20%。如果这个隐含的远期收益率在一年后完全实现,那么当前的两年期零息债券将在明年价值(100 / 1.1020 = 90.74)。当前,这个零息债券价值是(100 / 1.08083^2 = 85.61)。因此,它在下一年的收益率将是(90.74 / 85.61 - 1 = 6\%),与当前的一年期即期收益率完全相同。因此,10.20%是未来一年期即期收益率的盈亏平衡水平。换句话说,在下一年两年期零息债券的表现不如一年期零息债券之前,一年期即期收益率必须增加420个基点(10.20%-6.00%)。如果一年期即期收益率上升,但低于420个基点,相对于一年期零息债券,两年期零息债券的资本损失将不会完全抵消其初始收益率的优势(译注:初始收益率的优势是指两年期零息债券的年化收益率高于一年期零息债券的年化收益率)。
More generally, if the yield changes implied by the forward rates are subsequently realized, all government bonds, regardless of maturity, earn the same holding-period return. In addition, all self-financed positions of government bonds (such as long a barbell versus short a bullet) earn a return of 0%; that is, they break even. In contrast, if the yield curve remains unchanged over a year, each n-year zero earns the corresponding one-year forward rate (f_{n-1,n}). This can be seen from Equation (2) when m = n-1; (1+f_{n-1,n}) equals ((1+s_{n})^n / (1+s_{n-1})^{n-1}), which is the holding-period return from buying an n-year zero at rate (s_n), and selling it one year later at rate (s_{n-1}). Thus, the one-year forward rate equals a zero's horizon return for an unchanged yield curve (see Appendix C for details).
更一般地说,如果远期收益率隐含的收益率变化随后实现,所有不同期限的政府债券都会获得相同的持有期回报。此外,所有自融资的政府债券组合(如做多杠铃组合并做空子弹组合)都获得0%的回报。也就是说,实现了收支平衡。相反,如果收益率曲线在一年中保持不变,则每个n年期零息债券将赚取相应的一年期远期收益率(f_{n-1,n})。这可以从等式(2)中看出,当 m = n-1; (1+f_{n-1,n})等于((1+s_n)^n / (1+s_{n-1})^{n-1}),相当于今年以年化收益率为(s_n)买入,并在一年后以(s_{n-1})的收益率卖出。因此,一年期远期收益率等于不变收益率曲线情况下的零息债券的持有期回报(详见附录C)。
MAIN INFLUENCES ON THE YIELD CURVE SHAPE
影响收益率曲线形状的主要因素
In this section, we describe some economic forces that influence the term structure of forward rates or, more generally, the yield curve shape. The three main influences are the market's rate expectations, the bond risk premia (expected return differentials across bonds) and the so-called convexity bias. In fact, these three components fully determine the yield curve; we will show in later reports that the difference between each one-year forward rate and the one-year spot rate is approximately equal to the sum of an expected spot rate change, a bond risk premium and the convexity bias. We first discuss how each component influences the curve shape, and then we analyze their combined impact.
在本节中,我们描述一些影响远期收益率期限结构,或更一般地说,影响收益率曲线形状的经济因素。三个主要的影响因素是市场的收益率预期、债券风险溢价(不同债券预期回报的差)和所谓的凸度偏差。事实上,这三个分量完全决定了收益率曲线。我们将在后续的报告中揭示,一年期远期收益率和一年期即期收益率之间的差异大约等于即期收益率的预期变化、债券风险溢价和凸度偏差的总和。我们首先讨论每个因素如何影响曲线形状,然后分析它们的组合产生的影响。
Rate Expectations
收益率预期
It is clear that the market's expectation of future rate changes is an important determinant of the yield curve shape. For example, a steeply upward-sloping curve may indicate that the market expects near-term Fed tightening or rising inflation. However, it may be too restrictive to assume that the yield differences across bonds with different maturities only reflect the market's rate expectations. The well-known pure expectations hypothesis has such an extreme implication. The pure expectations hypothesis asserts that all government bonds have the same near-term expected return (as the nominally riskless short-term bond) because the return-seeking activity of risk-neutral traders removes all expected return differentials across bonds. Near-term expected returns are equalized if all bonds that have higher yields than the short-term rate are expected to suffer capital losses that offset their yield advantage. When the market expects an increase in bond yields, the current term structure becomes upward-sloping so that any long-term bond's yield advantage and expected capital loss (due to the expected yield increase) exactly offset each other. In other words, if investors expect that their long-term bond investments will lose value because of an increase in interest rates, they will require a higher initial yield as a compensation for duration extension. Conversely, expectations of yield declines and capital gains will lower current long-term bond yields below the short-term rate, making the term structure inverted.
很明显,市场对未来收益率变化的预期是收益率曲线形状的重要决定因素。例如,陡峭向上倾斜的曲线可能表明市场预期美联储近期将采取紧缩政策或通货膨胀上升。然而,假设不同期限债券收益率的差异仅反映市场对收益率预期的观点可能太过局限。众所周知的完全预期假说就有这样的极端含义。完全预期假说认为所有政府债券具有相同的近期预期回报(作为名义上无风险的短期债券),因为风险中性交易者的逐利行为消除了债券间的预期回报差异。如果所有收益率比短期收益率高的债券产生的资本损失抵消其收益率优势,则近期预期回报将相等。当市场预期债券收益率增加时,当前期限结构变得向上倾斜,因此任何长期债券的收益率优势和预期资本损失(由于预期收益率增长)完全相互抵消。换句话说,如果投资者预期他们的长期债券投资将因收益率上升而失去价值,那么他们需要更高的初始收益率作为久期增加的补偿。相反,预期收益率下降和资本回报将使当前长期债券收益率低于短期收益率,期限结构出现倒挂。
The same logic —— that positive (negative) initial yield spreads offset expected capital losses (gains) to equate near-term expected returns —— also holds for combinations of bonds, including duration-neutral yield curve positions. One example is a trade that benefits from the flattening of the yield curve between two- and ten-year maturities: selling a unit of the two-year bond, buying a duration-weighted amount of the ten-year bond and putting the remaining proceeds from the sale to "cash" (very short-term bonds). Given the typical concave yield curve shape (as a function of duration), such a curve flattening position earns a negative carry.[7] The trade will be profitable only if the curve flattens enough to offset the impact of the negative carry. Implied forward rates indicate how much flattening (narrowing of the two-to ten-year spread) is needed for the trade to break even.
同样的逻辑,正(负)的初始利差抵消预期资本损失(回报)以使近期预期回报相等,这也适用于包括久期中性策略在内的债券组合。举一个例子,从两年期和十年期之间收益率曲线的平坦化盈利:卖出一个单位的两年期债券,购买一个依久期加权的十年期债券并把剩余的回报作为“现金”持有(相当于非常短期的债券)。给定典型的上凸曲线(作为久期的函数),曲线在变平的过程中产生负的Carry。只有当曲线变平的程度足以抵消负Carry的影响时,交易才会有利可图。隐含的远期收益率表示了曲线需要变平多少(两年与十年的利差收窄)才能使交易保持收支平衡。
In the same way as the market's expectations regarding the future level of rates influence the steepness of today's yield curve, the market's expectations regarding the future steepness of the yield curve influence the curvature of today's yield curve. If the market expects more curve flattening, the negative carry of the flattening trades needs to increase (to offset the expected capital gains), making today's yield curve more concave (curved). Figure 2 illustrates these points. This figure plots coupon bonds' yields against their durations or, equivalently, zeros yields against their maturities, given various rate expectations. Ignoring the bond risk premia and convexity bias, if the market expects no change in the level or slope of the curve, today's yield curve will be horizontal. If the market expects a parallel rise in rates over the next year but no reshaping, today's yield curve will be linearly increasing (as a function of duration). If the market expects rising rates and a flattening curve, today's yield curve will be increasing and concave (as a function of duration).[8]
与市场对未来收益率水平的预期影响当前收益率曲线陡峭程度的方式相同,市场对收益率曲线未来陡峭程度的预期会影响当前收益率曲线的曲率。如果市场预期曲线变平,做平交易的负Carry将增加(以抵消预期的资本回报),使当前的收益率曲线更加上凸(弯曲)。图2说明了这些点。这副图根据不同的收益率预期,绘制了付息债券的收益率与其久期的关系,或等效地,零息债券收益率与其期限的关系。忽略债券风险溢价和凸度偏差,如果市场预期曲线的水平或斜率没有变化,当前的收益率曲线将是水平的。如果市场预期明年的收益率同时上涨但形状不变,当前的收益率曲线将呈线性增长(作为久期的函数)。如果市场预期收益率上升并且曲线变平,当前的收益率曲线将是向上倾斜和上凸的(作为久期的函数)。
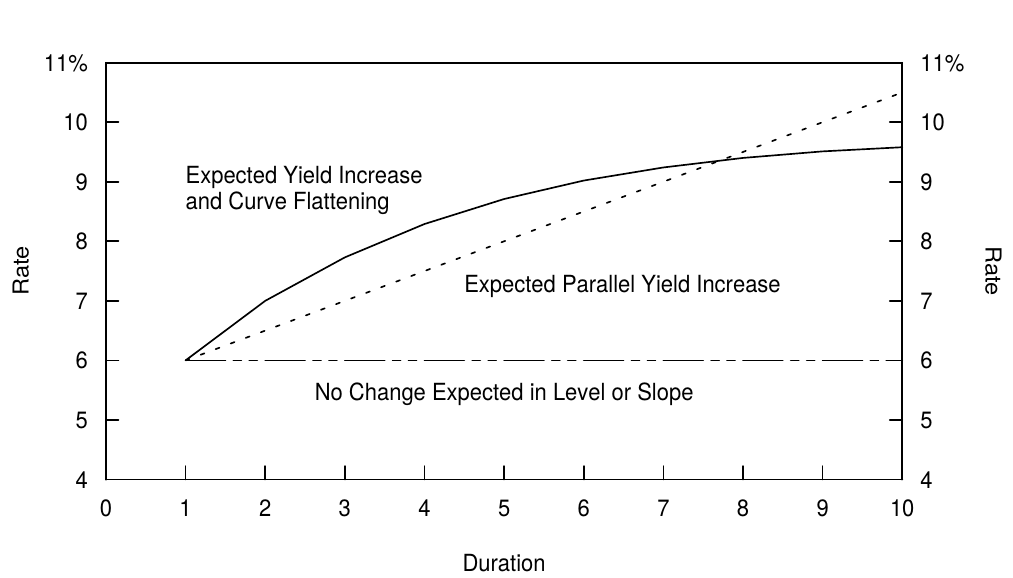
Figure 2. Yield Curves Given the Market's Various Rate Expectations
Bond Risk Premium
债券风险溢价
A key assumption in the pure expectations hypothesis is that all government bonds, regardless of maturity, have the same expected return. In contrast, many theories and empirical evidence suggest that expected returns vary across bonds. We define the bond risk premium as a longer-term bond's expected one-period return in excess of the one-period bond's riskless return. A positive bond risk premium would tend to make the yield curve slope upward. However, various theories disagree about the sign (+/-), the determinants and the constancy (over time) of the bond risk premium. The classic liquidity premium hypothesis argues that most investors dislike short-term fluctuations in asset prices; these investors will hold long-term bonds only if they offer a positive risk premium as a compensation for their greater return volatility. Also some modern asset pricing theories suggest that the bond risk premium should increase with a bond's duration, its return volatility or its covariance with market wealth. In contrast, the preferred habitat hypothesis argues that the risk premium may decrease with duration; long-duration liability holders may perceive the long-term bond as the riskless asset and require higher expected returns for holding short-term assets. While academic analysis focuses on risk-related premia, market practitioners often emphasize other factors that cause expected return differentials across the yield curve. These include liquidity differences between market sectors, institutional restrictions and supply and demand effects. We use the term "bond risk premium" broadly to encompass all expected return differentials across bonds, including those caused by factors unrelated to risk.
完全预期假说中的一个关键假设是,所有不同期限的政府债券都具有相同的预期回报。不过,许多理论和实证证据表明债券的预期回报不同。我们将债券风险溢价定义为长期债券的预期回报超过债券无风险回报的部分。正的债券风险溢价将倾向于使收益率曲线向上倾斜。然而,各种理论对于债券风险溢价的符号(+/-)、决定因素和恒定性(随时间)的看法不同。经典流动性溢价假说认为大多数投资者不喜欢资产价格的短期波动,这些投资者持有长期债券除非长期债提供正的风险溢价作为其更大的波动率的补偿。还有一些现代资产定价理论表明,债券风险溢价应该随债券的久期、回报的波动率或与市场财富的协方差而增加。相比之下,优先偏好假说(preferred habitat hypothesis)认为风险溢价可能随久期而减少,长久期负债持有人可能认为长期债券是无风险资产,并且对持有短期资产要求更高的预期回报。虽然学术分析侧重于研究风险相关的溢价,但市场从业者通常强调其他导致收益率曲线上预期回报不同的因素。这些因素包括不同市场之间的流动性差异、制度限制和供求关系影响。我们使用术语“债券风险溢价”广泛地涵盖债券所有的预期回报差异,包括那些与风险无关的因素引起的回报差异。
Historical data on US Treasury bonds provide evidence about the empirical behavior of the bond risk premium. For example, the fact that the Treasury yield curve has been upward sloping nearly 90% of the time in recent decades may reflect the impact of positive bond risk premia. Historical average returns provide more direct evidence about expected returns across maturities than do historical yields. Even though weekly and monthly fluctuations in bond returns are mostly unexpected, the impact of unexpected yield rises and declines should wash out over a long sample period. Therefore, the historical average returns of various maturity sectors should reflect the long-run expected returns.
美国国债的历史数据提供了债券风险溢价经验行为的证据。例如,近几十年国债收益率曲线90%的时间内呈现上升形态,可能反映了债券的正风险溢价的影响。较历史收益率而言,历史平均收益率提供了更多预期回报因期限不同的直接证据。尽管债券回报的周度和月度的大幅波动大多是不可预见的,但收益率意外上升和下跌的影响应在长样本期内清除掉。因此,各不同期限的历史平均回报应该反映了长期预期回报。
Figure 3 shows the empirical average return curve as a function of average duration and contrasts it to one theoretical expected return curve, one that increases linearly with duration. The theoretical bond risk premia are measured in Figure 3 by the difference between the annualized expected returns at various duration points and the annualized return of the riskless one-month bill (the leftmost point on the curve). Similarly, the empirical bond risk premia are measured by the historical average bond returns at various durations in excess of the one-month bill.[9] Historical experience suggests that the bond risk premia are not linear in duration, but that they increase steeply with duration in the front end of the curve and much more slowly after two years. The concave shape may reflect the demand for long-term bonds from pension funds and other long-duration liability holders.
图3示出了做为平均久期函数的经验平均回报曲线,并且将其与一个理论预期回报曲线对比,其中理论预期回报曲线随久期线性增加。图3中不同久期的理论债券风险溢价通过年化预期回报与无风险一月期国库券(曲线上的最左点)年化回报之间的差来度量。同样,不同久期的经验债券风险溢价是通过历史平均债券回报超过一月期国库券年化回报的部分来衡量的。历史经验表明,债券风险溢价关于久期不是线性的,曲线的前端迅速增加,而两年后增加速度要慢得多。上凸的形状可以反映养老基金和其他长久期负债持有人对长期债券的需求。

Figure 3. Theoretical and Empirical Bond Risk Premia
Figure 3 may give us the best empirical estimates of the long-run average bond risk premia at various durations. However, empirical studies also suggest that the bond risk premia are not constant but vary over time. That is, it is possible to identify in advance periods when the near-term bond risk premia are abnormally high or low. These premia tend to be high after poor economic conditions and low after strong economic conditions. A possible explanation for such countercyclic variation in the bond risk premium is that investors become more risk averse when their wealth is relatively low, and they demand larger compensation for holding risky assets such as long-term bonds.[10]
图3可以给出在不同久期平均债券风险溢价的最佳实证估计。然而,实证研究还表明债券风险溢价不是恒定的,而是随时间变化。也就是说,可以预先识别近期债券风险溢价异常的时点。这些风险溢价往往在经济衰退的情况下变高,而在经济强劲之后变低。债券风险溢价的这种反周期变化的一个可能的解释是,当他们的财富相对较低时,投资者变得更加风险厌恶,当持有风险资产如长期债券时,他们要求更大的风险补偿。
Convexity Bias
凸度偏差
The third influence on the yield curve —— the convexity bias —— is probably the least well known. Different bonds have different convexity characteristics, and the convexity differences across maturities can give rise to (offsetting) yield differences. In particular, long-term zeros exhibit very high convexity (see top panel of Figure 4), which tends to depress their yields. Convexity bias refers to the impact these convexity differences have on the yield curve shape.
第三个影响收益率曲线的因素——凸度偏差,可能是最不为人所知的。不同的债券具有不同的凸度特征,并且不同期限的凸度差异可以产生(抵消)收益率差异。特别地,长期零息债券具有非常高的凸度(参见图4的上部),这倾向于降低其收益率。凸度偏差是指凸度差异对收益率曲线形状的影响。
Convexity is closely related to the nonlinearity in the bond price-yield relationship. All noncallable bonds exhibit positive convexity; their prices rise more for a given yield decline than they fall for a similar yield increase. All else being equal, positive convexity is a desirable characteristic because it increases a bond's return (relative to return in the absence of convexity) whether yields go up or down —— as long as they move somewhere. Because positive convexity can only improve a bond's performance (for a given yield), more convex bonds tend to have lower yields than less convex bonds with the same duration.[11] In other words, investors tend to demand less yield if they have the prospect of improving their returns as a result of convexity. Investors are primarily interested in expected returns, and these high-convexity bonds can offer a given expected return at a lower yield level.
凸度与债券价格-收益率关系中的非线性部分密切相关。所有非可赎回债都有正的凸度,收益率下降对应的价格上升程度要比收益率上升产生的多一点。所有其他条件相同的情况下,正凸度是一个理想的特征,因为它增加了债券的回报(相对于没有凸度时的回报),无论收益率是上升还是下降。因为对于给定的收益率,正凸度能提高债券的表现,所以高凸度债券的收益率往往低于具有相同久期但凸度低的债券。换句话说,如果投资者预期通过增加凸度来提高回报,他们会接受较低的收益率。投资者主要对预期回报感兴趣,这些高凸度债券可以在较低的收益率水平下提供给定的预期回报。
The lower panel of Figure 4 illustrates the pure impact of convexity on the curve shape by plotting the spot rate curve and the curve of one-year forward rates when all bonds have the same expected return (8%) and the short-term rates are expected to remain at the current level. With no bond risk premia and no expected rate changes, one might expect these curves to be horizontal at 8%. Instead, they slope down at an increasing pace because lower yields are needed to offset the convexity advantage of longer-duration bonds and thereby to equate the near-term expected returns across bonds.[12] Short-term bonds have little convexity; therefore, there is little convexity bias at the front end of the yield curve, but convexity can have a dramatic impact on the curve shape at very long durations. Convexity bias can be one of the main reasons for the typical concave yield curve shape (that is, for the tendency of the curve to flatten or invert at long durations).
通过绘制即期收益率曲线和一年期远期收益率曲线(所有债券具有8%的预期回报并且短期收益率也为8%),图4的下半部分显示了凸度对曲线形状的影响。没有债券风险溢价和预期收益率变化的情况下,可以预期这些曲线将维持在8%的水平。然而,曲线加速下降,因为需要较低的收益率以抵消较长期债券的凸度优势,从而使债券的近期预期回报相等。短期债券几乎没有凸度,因此在曲线的前端几乎没有凸度偏差,但是凸度在长久期端对曲线形状具有显着的影响。凸度偏差是收益率曲线呈现典型的上凸形态的主要原因之一(因为曲线变平的趋势或在长久期端的倒挂)。
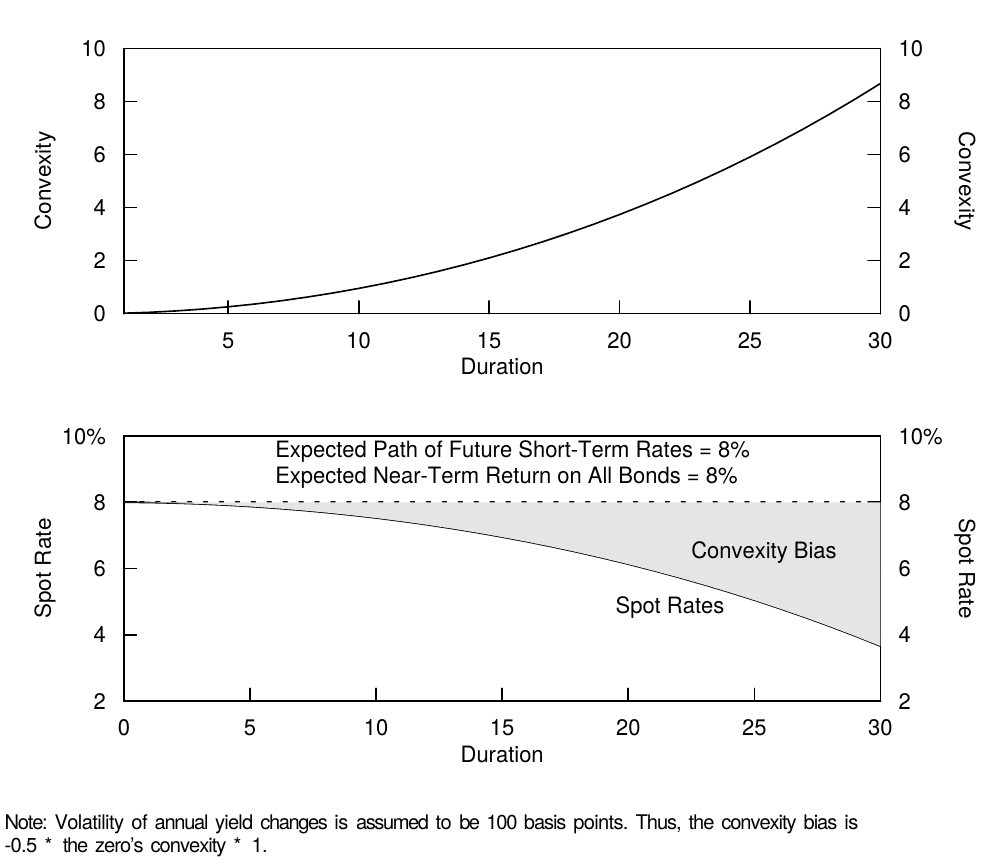
Figure 4. Convexity and the Yield Curve
The value of convexity increases with the magnitude of yield changes. Therefore, increasing volatility should make the overall yield curve shape more concave (curved) and widen the spreads between more and less convex bonds (duration-matched coupon bonds versus zeros and barbells versus bullets).[13]
凸度的价值随着收益率变化程度的大小而增加。因此,波动率增加会使收益率曲线形状更上凸(弯曲),并加大在不同凸度债券(久期匹配的付息债券-零息债券和杠铃-子弹组合)之间的利差。
Putting the Pieces Together
总结
Of course, all three forces influence bond yields simultaneously, making the task of interpreting the overall yield curve shape quite difficult. A steeply upward-sloping curve can reflect either the market's expectations of rising rates or high required risk premia. A strongly humped curve (that is, high curvature) can reflect the market's expectations of either curve flattening or high volatility (which makes convexity more valuable), or even the concave shape of the risk premium curve.
当然,所有三种因素在同时影响债券收益率,这使得从总体上解释收益率曲线形状的任务变得相当困难。陡峭向上倾斜的曲线可以反映市场对收益率上升的预期抑或是增高的风险溢价。高度弯曲的曲线(即高曲率)可以反映市场对曲线变平或高波动性(使凸度更有价值)的预期,甚至反映风险溢价曲线的上凸。
In theory, the yield curve can be neatly decomposed into expectations, risk premia and convexity bias. In reality, exact decomposition is not possible because the three components vary over time and are not directly observable but must be estimated.[14] Even though an exact decomposition is not possible, the analysis in this and subsequent reports should give investors a framework for interpreting various yield curve shapes. These reports will characterize the behavior of rate expectations, risk premia and convexity bias; show how they influence the curve; and evaluate the magnitude of their impact using historical data. Furthermore, our survey of earlier literature and our new empirical work will evaluate which theories and market myths are correct (consistent with data) and which are false. The main conclusions are as follows:
理论上,收益率曲线可以完全分解为预期、风险溢价和凸度偏差三部分。实际上,精确分解是不可能的,因为三个分量随时间变化,并且不能直接观察到,必须估计。即使不可能进行精确分解,本报告和后续报告中的分析也能为投资者提供解释各种收益率曲线形状的框架。这些报告将描述预期收益率、风险溢价和凸度偏差的行为,显示它们如何影响曲线,并使用历史数据测算其影响的程度。此外,我们对早期文献的研究和我们新的实证工作将评估哪些理论和市场传言是正确的(与数据一致),哪些是错误的。主要结论如下:
- We often hear that "forward rates show the market's expectations of future rates." However, this statement is only true if no bond risk premia exist and the convexity bias is very small.[15] If the goal is to infer expected short-term rates one or two years ahead, the convexity bias is so small that it can be ignored. In contrast, our empirical analysis shows that the bond risk premia are important at short maturities. Therefore, if the forward rates are used to infer the market's near-term rate expectations, some measures of bond risk premia should be subtracted from the forwards, or the estimate of the market's rate expectations will be strongly upward biased.
- 我们经常听到“远期收益率显示市场对未来收益率的预期”。然而,这种说法只有在没有债券风险溢价存在且凸度偏差非常小的时候才是真的。如果目标是推断一到两年后的预期短期收益率,这时的凸度偏差非常小,以至于可以忽略。相比之下,我们的实证分析表明,债券风险溢价对短期收益率的影响是重要的。因此,如果远期收益率用于推断市场对近期收益率的预期,则应该从远期收益率中减去债券风险溢价,否则市场对收益率的预期将严重被高估。
- The traditional term structure theories assume a zero risk premium (pure expectations hypothesis) or a nonzero but constant risk premium (liquidity premium hypothesis, preferred habitat hypothesis) which is inconsistent with historical data. According to the pure expectations hypothesis, an upward-sloping curve should predict increases in long-term rates, so that a capital loss offsets the long-term bonds' yield advantage. However, empirical evidence shows that, on average, small declines in long-term rates, which augment the long-term bonds' yield advantage, follow upward-sloping curves. The steeper the yield curve is, the higher the expected bond risk premia. This finding clearly violates the pure expectations hypothesis and supports hypotheses about time-varying risk premia.
- 传统的期限结构理论假定零风险溢价(完全预期假说)或非零但恒定的风险溢价(流动性溢价假说、优先偏好假说),这与历史数据不一致。根据完全预期假说,向上倾斜的曲线预测长期收益率的增长,从而资本损失抵消长期债券的收益率优势。然而,实证证据表明,曲线向上倾斜时,长期收益率的小幅下降将增加长期债券的收益率优势。收益率曲线越陡,预期债券风险溢价就越高。这一发现明显违反完全预期假说,反而支持关于时变风险溢价的假设。
- Modern term structure models make less restrictive assumptions than the traditional theories above. Yet, many popular one-factor models assume that bonds with the same duration earn the same expected return. Such an assumption implies that duration-neutral positions with more or less convexity earn the same expected return (because a yield disadvantage exactly offsets any convexity advantage). However, if the market values very highly the insurance characteristics of positively convex positions, more convex positions may earn lower expected returns. Our analysis of the empirical performance of duration-neutral barbell-bullet trades will show that, in the long run, barbells tend to marginally underperform bullets.
- 比于上述传统理论,现代期限结构模型制定的约束假设更少。然而,许多流行的单因子模型假设,具有相同久期的债券获得相同的预期回报。这种假设意味着无论凸度多少,久期中性的头寸获得相同的预期回报(因为收益率的劣势恰好抵消了任何凸度的优势)。然而,如果市场为正凸度的保险特性给出高定价,则凸度大的头寸将获得较低的预期回报。我们对久期中性的杠铃-子弹组合的实证表现分析显示,从长远来看,杠铃组合的表现倾向于略微落后于子弹组合。
USING FORWARD RATE ANALYSIS IN YIELD CURVE TRADES
在曲线交易中使用远期收益率分析
Recall that if the pure expectations hypothesis holds, all bond positions have the same near-term expected return. In particular, an upward-sloping yield curve reflects expectations of rising rates and capital losses, and convexity is priced so that a yield disadvantage exactly offsets the convexity advantage. In such a world, yields do not reflect value, no trades have favorable odds and active management can add value only if an investor has truly superior forecasting ability. Fortunately, the real world is not quite like this theoretical world. Empirical evidence (presented in parts 2-4 of this series of reports) shows that expected returns do vary across bonds. The main reason is probably that most investors exhibit risk aversion and preferences for other asset characteristics; moreover, investor behavior may not always be fully rational. Therefore, yields reflect value and certain relative value trades have favorable odds.
回想一下,如果完全预期假说成立,所有债券都有相同的近期预期回报。特别地,向上倾斜的收益率曲线反映了加息和资本损失的预期,并且凸度被定价,使得收益率劣势恰好抵消凸度优势。在这样的世界中,收益率不反映价值,没有有利的交易,除非投资者具有真正优秀的预测能力,否则积极的管理无法增加价值。幸运的是,现实世界不太像这个理论世界。经验证据(在本系列报告的第2-4部分)表明,债券的预期回报是不同的。主要原因可能是大多数投资者表现出风险厌恶,并偏好其他资产特征。此外,投资者的行为可能不总是完全理性的。因此,收益率反映价值,并且某些相对价值交易有利可图。
The previous section provided a framework for thinking about the term structure shapes. In this section, we describe practical applications —— different ways to use forward rates in yield curve trades. The first approach requires strong subjective rate views and faith in one's forecasting ability.
上一节提供了一个用于研究收益率期限结构形状的框架。在本节中,我们描述实际应用——在收益率曲线交易中使用远期收益率的不同方式。第一种方法需要强烈的主观观点和对预测能力的信心。
Forwards as Break-Even Rates for Active Yield Curve Views
主观收益率曲线观点下远期收益率做为盈亏平衡收益率
The forward rates show a path of break-even future rates and spreads. This path provides a clear yardstick for an active portfolio manager's subjective yield curve scenarios and yield path forecasts. It incorporates directly the impact of carry on the profitability of the trade. For example, a manager should take a bearish portfolio position only if he expects rates to rise by more than what the forwards imply. However, if he expects rates to rise by less than what the forwards imply (that is, by less than what is needed to offset the positive carry), he should take a bullish portfolio position. If the manager's forecast is correct, the position will be profitable. In contrast, managers who take bearish portfolio positions whenever they expect bond yields to rise —— ignoring the forwards —— may find that their positions lose money, because of the negative carry, even though their rate forecasts are correct.
远期收益率显示了盈亏平衡的未来收益率和利差的路径。这个路径为主动投资组合管理者看待收益率曲线和预测收益率路径提供了一个明确的标准。它直接显示了对交易盈利的影响。例如,如果预计收益率上升幅度将超过远期收益率,管理者应该看跌。然而,如果他预期收益率上升幅度小于远期收益率的幅度(即小于抵消正Carry对应的利差),他应该看多。如果管理者的预测是正确的,那么当前头寸将是有利可图的。相比之下,即使管理者对收益率的预测是正确的,如果他们预期债券收益率上升,但忽视远期收益率的分析,依然会发现他们的头寸亏损,因为头寸产生负的Carry。
One positive aspect about the role of forward rates as break-even rates is that it does not depend on assumptions regarding expectations, risk premia or convexity bias. The rules are simple. If forward rates are realized, all positions earn the same return. If yields rise by more than the forwards imply, bearish positions are profitable and bullish positions lose money. If yields rise by less than the forwards imply, the opposite is true. Similar statements hold for any yield spreads and related positions, such as curve-flattening positions.
远期收益率作为盈亏平衡收益率的一个积极方面是它不依赖于关于预期、风险溢价或凸度偏差的假设。规则很简单,如果实现远期收益率,所有头寸将获得相同的回报。如果收益率上升超过远期收益率对应的部分,空头是有利可图,多头亏损。如果收益率上升小于远期收益率对应的部分,情况则相反。类似的陈述适用于任何利差和相关头寸,例如做平曲线的头寸。
Figure 5 shows the US par yield curve and the implied par curves three months forward and 12 months forward based on the Salomon Brothers Treasury Model as of March 31, 1995. If we believe that forward rates only reflect the market's rate expectations, a comparison of these curves tells us that the market expects rates to rise and the curve to flatten over the next year. Alternatively, the implied yield rise may reflect a bond risk premium and the implied curve flattening may reflect the value of convexity. Either way, the forward yield curves reflect the break-even levels between profits and losses.
图5显示了1995年3月31日“Salomon Brothers 国债模型”测算的美国国债票面收益率曲线和提前三个月与12个月的票面收益率曲线。如果我们认为远期收益率只反映市场对收益率的预期,这些曲线告诉我们,市场预期收益率将上升,曲线在明年趋于平缓。或者,隐含收益率曲线上升可能反映债券风险溢价,隐含收益率曲线变平可能反映凸度价值。无论如何,远期收益率曲线反映了盈亏平衡水平。
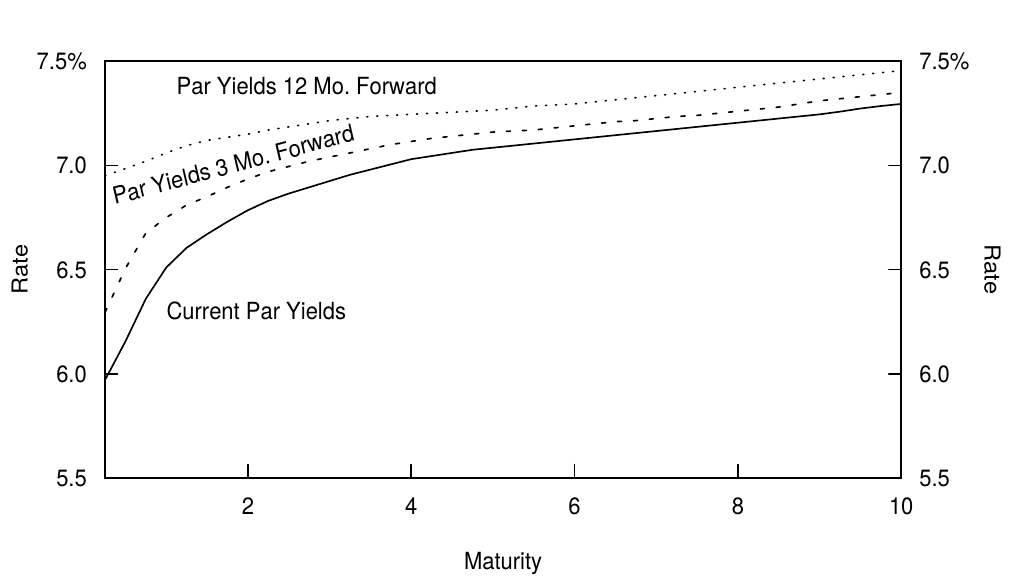
Figure 5. Current and Forward Par Yield Curves, as of 31 Mar 95
The information in the forward rate structure can be expressed in several ways. Figure 5 is useful for an investor who wants to contrast his subjective view of the future yield curve with an objective break-even curve at some future horizon. Another graph may be more useful for an investor who wants to see the break-even future path of any constant-maturity rate (instead of the whole curve) and contrast it with his own forecast, which may be based on a macroeconomic forecast or on the subjective view about the speed of Fed tightening. As an example, Figure 6 shows such a break-even path of future three-month rates at the end of March 1995.[16] To add perspective, the graph also contains the historical path of the three-month rate over the past eight years and the break-even path of the future three-month rates at the end of 1994 when the Treasury market sentiment was much more bearish.
远期收益率结构中的信息可以以几种方式表示。图5客观地提供了未来某个期间的盈亏平衡曲线,投资者可以将其同自己对未来收益率曲线的主观预测进行对比。投资者会基于宏观经济预测或关于美联储财政紧缩速度的主观观点判断收益率走势,这时将固定期限的盈亏平衡收益率的未来路径(而不是整个曲线)与自己的预测进行对比显得非常有用。作为一个例子,图6显示了1995年3月底以来三月期收益率的盈亏平衡路径。为了加深理解,图表还包含过去八年三月期收益率的历史路径,以及1994年年底开始的三月期收益率盈亏平衡路径,那时国债市场的看空情绪更加强烈。
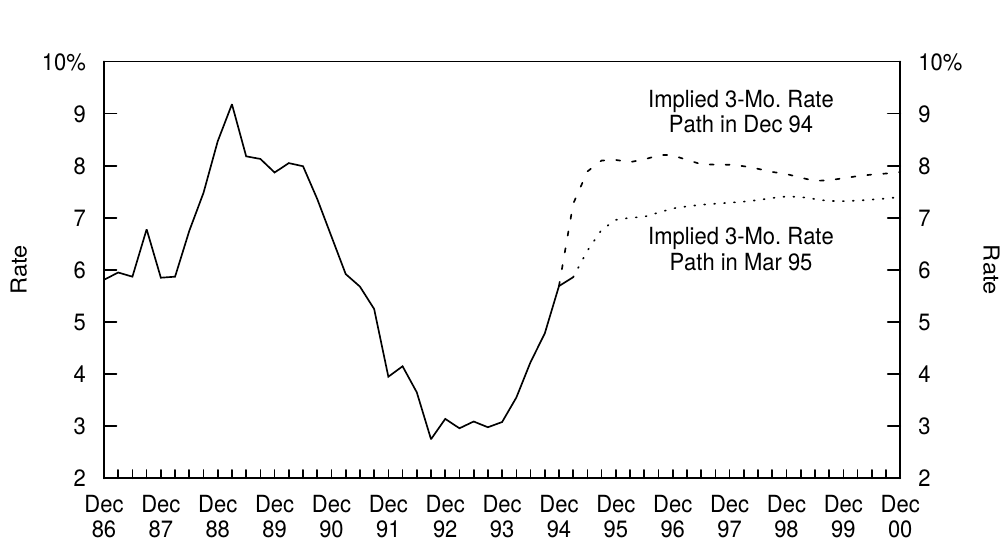
Figure 6. Historical Three-Month Rates and Implied Forward Three-Month Rate Path, as at 30 Dec 94 and 31 Mar 95
Forwards as Indicators of Cheap Maturity Sectors
远期收益率识别“便宜期限”
The other ways to use forwards require less subjective judgment than the first one. As a simple example, the forward rate curve can be used to identify cheap maturity sectors visually. Abnormally high forward rates are more visible than high spot or par rates because the latter are averages of forward rates.
另一个使用远期收益率的方法比前面的方法涉及更少的主观判断。一个简单的例子,远期收益率曲线可用于识别便宜期限。远期收益率中反常高的部分比即期收益率或到期收益率更明显,因为后者是远期收益率的平均化。
Figure 7 shows one real world example from the beginning of this decade when the par yield curve was extremely flat (although forwards may be equally useful when the par curve is not flat). Even though the par yield curve was almost horizontal (all par yields were within 25 basis points), the range of three-month annualized forward rates was almost 200 basis points because the forward rate curve magnifies the cheapness/richness of different maturity sectors. High forward rates identify the six-year sector and the 12-year sector as cheap, and low forward rates identify the four-year sector and the nine-year sector as expensive.[17]
图7显示一个现实的例子,这个十年开始时票面收益率曲线非常平坦(虽然在到期收益率曲线不平的情况下,远期收益率可能同样有用)。即使票面收益率曲线几乎是水平的(票面收益率的变动幅度在25个基点内),三月期年化远期收益率的变动范围几乎是200个基点,因为远期收益率曲线放大了不同期限的价格比。高远期收益率表示六年期和12年期债券相对便宜,低远期收益率表示四年期和九年期债券的价格被高估。
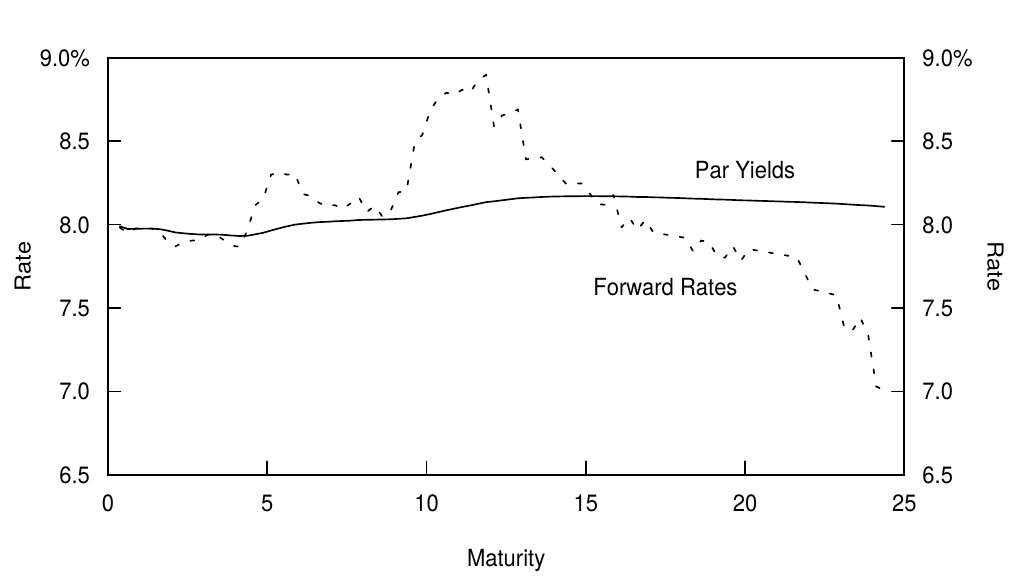
Figure 7. Par Yields and Three-Month Forward Rates, as of 2 Jan 90
Once an investor has identified a sector with abnormally high forward rates (for example, between nine and 12 years), he can exploit the cheapness of this sector by buying a bond that matures at the end of the period (12 years) and by selling a bond that matures at the beginning of the period (nine years). If equal market values of these bonds are bought and sold, the position is exposed to a general increase in rates and a steepening yield curve. More elaborate trades can be constructed (for example, by selling both the nine-year and 15-year bonds against the 12-year bonds with appropriate weights) to retain level and slope neutrality. To the extent that bumps and kinks in the forward curve reflect temporary local cheapness, the trade will earn capital gains when the forward curve becomes flatter and the cheap sector richens (in addition to the higher yield and rolldown the position earns). In the example of Figure 7, such "richening" actually did happen over the next three months.
一旦投资者识别了一个具有异常高远期收益率的期限(例如,9到12年),他可以通过做多在期末(12年)到期的债券和做空在期初(9年)到期的债券发掘被低估的价值。如果市值相等的上述债券被交易,那么这种组合的风险敞口是收益率走高和曲线变陡。可以构建更复杂的交易策略(例如,通过以适当的权重做空9年期和15年期债券同时做多12年期债券),以保持水平和斜率中性。远期收益率曲线中的波动和扭曲反映了部分期限的债券被暂时低估,当远期收益率曲线变得更平坦并且被低估的债券修复时交易将获得资本回报(除非收益率走高并降低头寸回报)。在图7的示例中,这样的“修复”实际上在接下来的三个月中发生了。
Forwards as Relative Value Tools for Yield Curve Trades
远期收益率作为收益率曲线交易中的相对价值工具
Above, forwards are used quite loosely to identify cheap maturity sectors. A more formal way to use forwards is to construct quantitative cheapness indicators for duration-neutral flattening trades, such as barbell-bullet trades. We first introduce some concepts with an example of a market-directional trade.
上面的例子中,远期收益率相当粗略地用于识别价值被低估的期限。使用远期收益率更正式的方法是为久期中性投资交易(如杠铃-子弹交易)构建识别价值低估的定量指标。我们首先介绍一些概念与市场方向性交易的例子。
When the yield curve is upward sloping, long-term bonds' yield advantage over the riskless short-term bond provides a cushion against rising yields. In a sense, duration extensions are "cheap" when the yield curve is very steep and the cushion (positive carry) is large. These trades only lose money if capital losses caused by rising rates offset the initial yield advantage. Moreover, the longer-term bonds' rolling yield advantages [18] over the short-term bond are even larger than their yield advantages. The one-year forward rate is, by construction, equal to the n-year zero's rolling yield (see Appendix C). Thus, it is a direct measure of the n-year zero's rolling yield advantage. (Another forward-related measure, the change in the n-1 year spot rate implied by the forwards, tells how much the yield curve has to shift to offset this advantage and to equate the holding-period returns of the n-year zero and the one-year zero.)
当收益率曲线向上倾斜时,长期债券的收益率优势相对于无风险短期债券提供了对收益率上升的缓冲。在某种意义上,当收益率曲线非常陡峭并且缓冲(正的Carry)大时,久期延长是“便宜的”。只有由于收益率上升造成的资本损失抵消了初始收益率优势,这些交易才会损失资金。此外,长期债券相对于短期债券的滚动收益率优势甚至大于其收益率优势。通过构造,一年期远期收益率等于n年期零息债券的滚动收益率(见附录C)。因此,它(远期收益率)是n年期零息债券滚动收益率优势的直接测量。(另一个与远期收益率相关的指标,即远期收益率所隐含的n-1年期即期收益率的变化,说明了收益率曲线必须变化多少以抵消这一优势,并在持有期使n年期零息债券的回报等于一年期零息债券)。
Because one-period forward rates measure zeros' near-term expected returns, they can be viewed as indicators of cheap maturity sectors. The use of such cheapness indicators does not require any subjective interest rate view. Instead, it requires a belief, motivated by history, that an unchanged yield curve is a good base case scenario.[19] If this is true, long-term bonds have higher (lower) near-term expected returns than short-term bonds when the forward rate curve is upward sloping (downward sloping). In the long run, a strategy that adjusts the portfolio duration dynamically based on the curve shape should earn a higher average return than constant-duration strategies.[20]
因为一年期远期收益率度量零息债券的近期预期回报,所以它们可被视为便宜期限的指标。使用这种指标不需要任何关于收益率的主观观点。相反,它需要一个由历史支持的信念,不变的收益率曲线是一个良好的基准。如果这是真的,当远期收益率曲线向上倾斜(向下倾斜)时,长期债券具有比短期债券更高的(更低的)近期预期回报。从长期来看,基于曲线形状动态调整投资组合久期的策略应该比恒定久期策略获得更高的平均回报。
Similar analysis holds for curve-flattening trades. Recall that when the yield curve is concave as a function of duration, any duration-neutral flattening trade earns a negative carry. Higher concavity (curvature) in the yield curve indicates less attractive terms for a flattening trade (larger negative carry) and more "implied flattening" by the forwards (which is needed to offset the negative carry). Therefore, the amount of spread change implied by the forwards is a useful cheapness indicator for yield curve trades at different parts of the curve. If the implied change is historically wide, the trade is expensive, and vice versa.
类似的分析适用于做平曲线交易。回想一下,当收益率曲线是久期的上凸函数时,任何久期中性的做平交易获得负Carry。收益率曲线的上凸程度(曲率)越高,表示做平交易越没有吸引力(较大的负Carry),远期收益率更加强烈的“隐含曲线的平坦化”(以抵消负Carry)。因此,对于收益率曲线交易,远期收益率隐含的利差变化是衡量曲线不同部分被低估程度的指标。如果隐含的变化处于历史高位,交易是昂贵的,反之亦然。
Figure 8 shows an example of a recent situation in which the flattening trades were extremely expensive. At the end of December 1994, the three-month to two-year sector of the Treasury curve was very steep (a spread of 200 basis points) and the two- to 30-year sector was quite flat (a spread of 20 basis points). The high curvature indicated strong flattening expectations —— forwards implied an inversion of the two- to 30-year spread by March —— or high expected volatility (high value of convexity).
图8给出了最近情况的示例,其中发现做平交易非常昂贵。1994年12月底,国债收益率曲线在三个月期至两年期的区间内非常陡(扩大了200个基点),两年期至30年期区间内却相当稳定(扩大了20个基点)。高曲率表明强烈的做平预期——远期收益率意味着三月份两到三十年期利差的倒挂——或高波动率的预期(高凸度价值)。
The barbell (of the 30-year bond and three-month bill) over the duration-matched two-year bullet would become profitable only if the curve flattened even more than the forwards implied or if a sudden increase in volatility occurred. Purely on yield grounds, the two-year bullet (a steepening position) appeared cheap in an absolute comparison (across bonds) and in a historical comparison (over time). With the benefit of hindsight, we know that the cheapness indicator gave a correct signal. The two-year bullet outperformed various duration-matched barbell positions substantially over the next quarter as it earned large capital gains in addition to its high initial yield and rolldown advantage. By the end of March, the front end of the curve had flattened by 108 basis points and the long end had steepened by 45 basis points. Figure 8 illustrates the decline in curvature by plotting the Treasury on-the-run yield curves (as a function of duration) on December 30 and on March 31. In later reports, we will show how to use forward rate analysis to evaluate opportunities like this.
当曲线变平的程度超过远期收益率隐含的量,或波动率突然增加时做多杠铃组合(30年期债券和三月期国库券),同时做空久期匹配的两年期子弹组合是有利可图的。纯粹基于收益率观察,两年期子弹组合(做陡)在横向比较(债券)和纵向历史比较(随着时间的推移)看来都被低估。有了后见之明,我们知道低估指标给出了正确的信号。两年期子弹组合在下个季度表现优于各种久期匹配的杠铃组合,因为它除了具有高的初始收益率和下滑回报优势之外,还获得了巨大的资本回报。截至3月底,曲线的前端已平坦化了108个基点,长端已经陡峭化了45个基点。图8通过绘制12月30日和3月31日的国债收益率曲线(作为久期的函数)显示了曲率的下降。在后面的报告中,我们将展示如何使用远期收益率分析来评估交易机会。
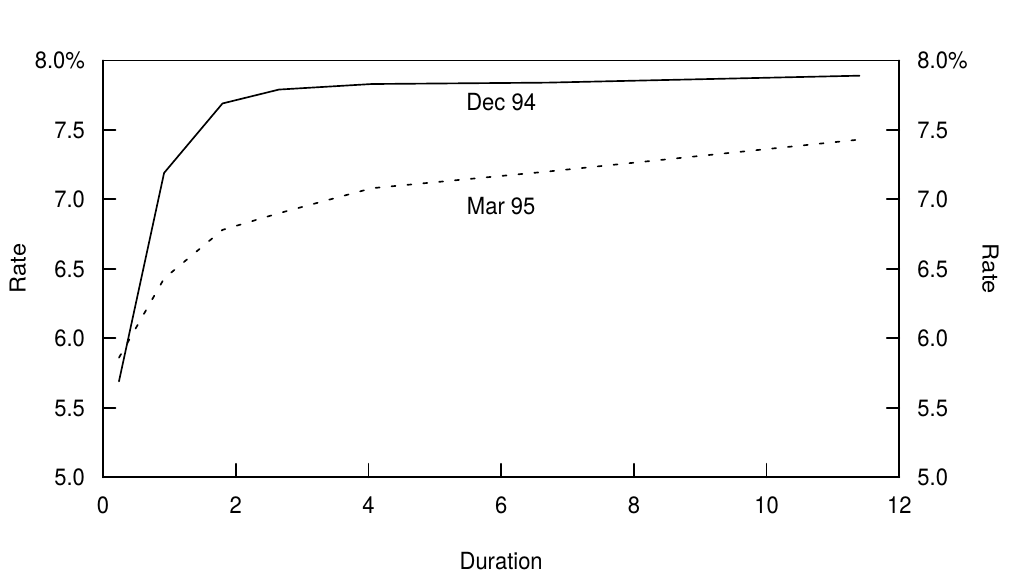
Figure 8. On-the-Run Yield Curves, as at 30 Dec 94 and 31 Mar 95
APPENDIX A. NOTATION AND DEFINITIONS USED IN THE SERIES UNDERSTANDING THE YIELD CURVE
附录A:《理解收益率曲线》系列报告中用到的符号与定义
| NOTATION | DEFINITIONS |
|---|---|
| (P) | Market price of a bond. |
| (P_n) | Market price of an n-year zero. |
| (C) | Coupon rate (in percentage; other rates are expressed as a decimal). |
| (y) | Annualized yield to maturity (YTM) of a bond. |
| n | Time to maturity of a bond (in years). |
| (s_n) | Annualized n-year spot rate; the discount rate of an n-year zero. |
| (s^*_{n-1}) | Annualized n-1 year spot rate next period; superscript * denotes next period's (year's) value. |
| (Delta s_{n-1}) | Realized change in the n-1 year spot rate between today and next period ((= s^*_{n-1} - s_{n-1})) |
| (f_{m,n}) | Annualized forward rate between maturities m and n, |
| (f_{n-1,n}) | One-year forward rate between maturities (n-1) and n; also the n-year zero's rolling yield. |
| (f_{1,n}) | Annualized forward rate between maturities 1 and n; also called the implied n-1 year spot rate one year forward. |
| (Delta f_{n-1}) | Implied change in the n-1 year spot rate between today and next period ((= f_{1,n} - s_{n-1})); also called the break-even yield change (over the next period) implied by the forwards. |
| (Delta fz_n) | Implied change in the yield of an n-year zero, a specific bond, over the next period ((= f_{1,n} - s_n)). |
| (FSP) | Forward-spot premium ((FSP_n = f_{n-1,n} - s_1)). |
| (h_n) | Realized holding—period return of an n-year zero over one period (year). |
| Rolling Yield | A bond's horizon return given a scenario of unchanged yield curve; sum of yield and rolldown return. |
| Bond Risk Premium (BRP) | Expected return of a long-term bond over the next period (year) in excess of the riskless one-period bond; for the n-year zero, (BRP_n = E(h_n - s_1)). |
| Realized BRP | Realized one-year holding-period return of a long-term bond in excess of the one-year bond; also called excess bond return; realized (BRP_n = h_n - s_1). |
| Persistence Factor (PF) | Slope coefficient in a regression of the annual realized (BRP_n) on (FSP_n). |
| Term Spread | Yield difference between a long-term bond and a short-term bond; for the n-year zero, (= s_n - s_1). |
| Real Yield | Difference between a long-term bond yield and a proxy for expected inflation; our proxy is the recently published year-on-year consumer price inflation rate. |
| Inverse Wealth | Ratio of exponentially weighted past wealth to the current wealth; we proxy wealth W by the stock market level; (= (W_{t-1} + 0.9^1 W_{t-2} + 0.9^2 W_{t-3} + ...) * 0.1 / W_t) |
| Duration (Dur) | Measure of a bond prices interest rate sensitivity; (Dur = -(dP/dy) * (1/P)) |
| Convexity (Cx) | Measure of the nonlinearity in a hands P/y -relation; (Cx = (d^2 P / dy^2) * (1/P)) |
| Convexity Bias (CB) | Impact of convexity on the curve of one-year forward rates; (CB_n = -0.5 * Cx_n * ( ext{Volatility of }Delta s_n )^2) |
| 符号 | 定义 |
|---|---|
| (P) | 债券市场价格 |
| (P_n) | n年期零息债券的市场价格 |
| (C) | 票息率(百分比,其他比率是数值) |
| (y) | 债券年化到期收益率 |
| n | 剩余期限 |
| (s_n) | 年化n年期即期收益率,n年期零息债券贴现率 |
| (s^*_{n-1}) | 下一期(年)年化n-1年期即期收益率,上标(*)表示下一期的年份 |
| (Delta s_{n-1}) | 当前与下一期n-1年期即期收益率的差,(= s^*_{n-1} - s_{n-1}) |
| (f_{m,n}) | m年到n年的年化远期收益率 |
| (f_{n-1,n}) | n-1年到n年的年化远期收益率,也是n年期零息债券的滚动收益率 |
| (f_{1,n}) | 1年到n年的年化远期收益率,也是隐含的一年后n-1年期即期收益率 |
| (Delta f_{n-1}) | 当前与下一期n-1年期即期收益率的隐含变化((f_{1,n}-s_{n-1})),也是远期收益率隐含的盈亏平衡收益率变化(较下一期) |
| (Delta fz_n) | 当前与下一期n年期零息债券收益率的隐含变化((= f_{1,n} - s_n)) |
| (FSP) | 远期-即期溢价((FSP_n = f_{n-1,n} - s_1)) |
| (h_n) | n年期零息债券持有一年的持有期回报 |
| 滚动收益率 | 收益率曲线不变情况下债券持有期回报,收益率与下滑回报的和 |
| 债券风险溢价 | 长期债券在下一期(年)的预期回报超过无风险一年期债券的部分,对于n年期零息债券,(BRP_n = E(h_n - s_1)) |
| 实现债券风险溢价 | 实现的长期债券在下一期(年)的预期回报超过无风险一年期债的部分,对于n年期零息债券,(BRP_n = h_n - s_1) |
| 持续因子(PF) | 年化实现(BRP_n)关于(FSP_n)回归的斜率参数 |
| 期限利差 | 长-短期债的收益率之差,对于n年期零息债券,(= s_n - s_1). |
| 真实收益率 | 长期债券的收益率与通胀指标的差,我们的通胀指标是年度CPI |
| 逆财富 | 过往财富的指数加权与当前财富的比值,我们用股票市场水平表示财富 (= (W_{t-1} + 0.9^1 W_{t-2} + 0.9^2 W_{t-3} + ...) * 0.1 / W_t) |
| 久期(Dur) | 债券价格关于收益率的敏感性,(Dur = -(dP/dy) * (1/P)) |
| 凸度(Cx) | 度量债券价格-收益率关系中的非线性部分,(Cx = (d^2 P / dy^2) * (1/P)) |
| 凸度偏差(CB) | 凸度对1年期远期收益率的影响,(CB_n = -0.5 * Cx_n * ( ext{Volatility of }Delta s_n )^2) |
APPENDIX B. CALCULATING SPOT AND FORWARD RATES WHEN PAR RATES ARE KNOWN
附录B:已知到期收益率计算即期和远期收益率
A simple example illustrates how spot rates and forward rates are computed on a coupon date when the par curve is known (and coupon payments and compounding frequency are annual). The basis of the procedure is the fact that a bond's price will be the same, the sum of the present values of its cash flows, whether it is priced via yield to maturity (Equation (4)) or via the spot rate curve (Equation (5)):
一个简单的例子说明在到期收益率曲线是已知的情况下(票息支付和复利频率是年度),在付息日如何计算即期收益率和远期收益率。计算的基础是无论通过到期收益率(等式(4))还是通过即期收益率(等式(5))计算,债券的价格(现金流的现值之和)将是相同的:
where P is the bond price, C is the coupon rate (in percentage), y is the annual yield to maturity (expressed as a decimal), s is the annual spot rate (expressed as a decimal), and n is the time to maturity (in years).
其中P是债券价格,C是票息率(以百分比计),y是年化到期收益率(以十进制小数表示),s是年化即期收益率(以十进制小数表示),n是到期期限(年)。
We only show the computation for the first two years, which have par rates of 6% and 8%. For the first year, par, spot, and forward rates are equal (6%). Longer spot rates are solved recursively using known values of the par bond's price and cash flows and the previously solved spot rates.
我们只显示前两年的计算,其到期收益率为6%和8%。对于第一年,到期、即期和远期收益率相等(6%)。使用已知的债券价格和现金流以及先前算出的即期收益率的值,递归求解更长期限对应的即期收益率。
Every par bond's price is 100 (par) by construction; thus, its yield (the par rate) equals its coupon rate. Because the two-year par bond's market price (100) and cash flows (8 and 108) are known, as is the one-year spot rate (6%), it is easy to solve for the two-year spot rate as the only unknown in the following equation:
根据合约,每个债券的价格是100(票面);因此,其收益率(票面收益率)等于其票息率。由于两年期债券的市场价格(100)和现金流(8和108)是已知的,一年期即期收益率(6%)也是如此,因此很容易解决两年期即期收益率仅在以下等式中未知:
A little manipulation shows that the solution for (s_2) is 8.08%. Equation (6) also can be used to compute par rates when only spot rates are known. If the spot rates are known, the coupon rate C, which equals the par rate, is the only unknown in Equation (6).
计算显示(s_2)的解是8.08%。当仅知道即期收益率时,等式(6)也可用于计算到期收益率。如果即期收益率是已知的,则等于票息率的票面收益率C是等式(6)中唯一未知的。
The forward rate between one and two years is computed using Equation (3) and the known one-year and two-year spot rates.
一年和两年之间的远期收益率使用公式(3)和已知的一年期和两年期的即期收益率计算。
The solution for (f_{1,2}) is 10.20%. The other spot rates and one-year forward rates ((f_{2,3}), (f_{3,4}), etc.) in Figure 9 are computed in the same way. These numbers are shown graphically in Figure 1.
(f_{1,2})的解为10.20%。图9中的其他即期收益率和一年期远期收益率((f_{2,3}),(f_{3,4}),...)以相同的方式计算。这些数字在图1中显示。
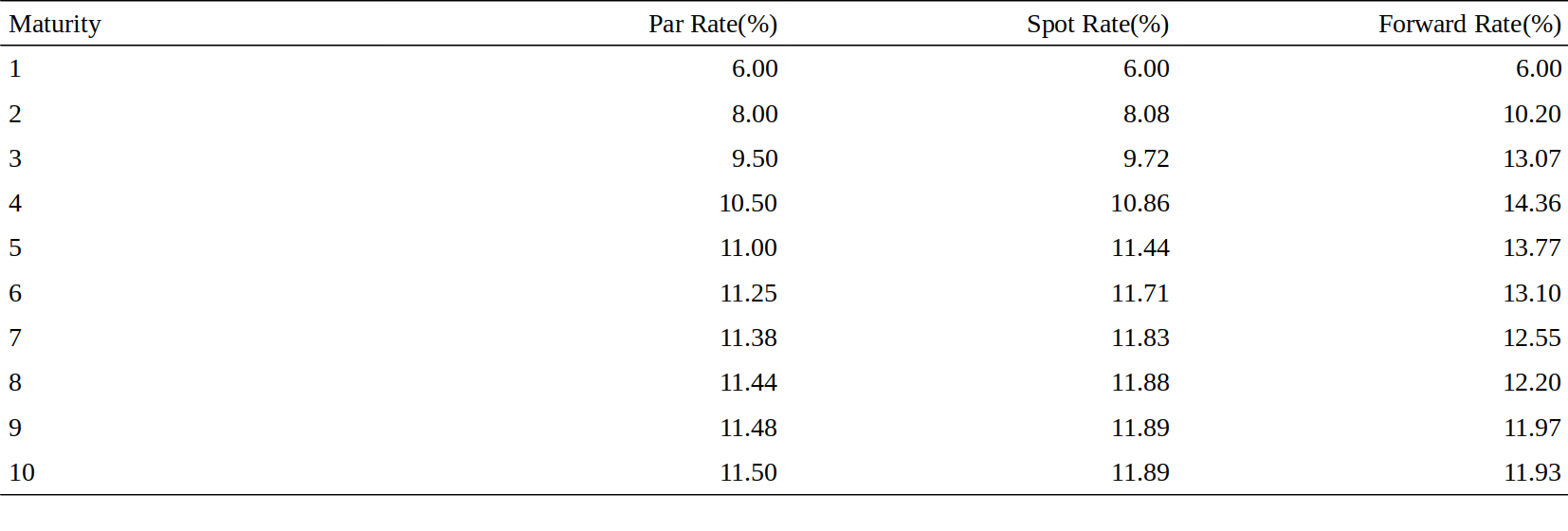
Figure 9. Par, Spot and One-Year Forward Rates
APPENDIX C. RELATIONS BETWEEN SPOT RATES, FORWARD RATES, ROLLING YIELDS, AND BOND RETURNS
附录C:即期收益率、远期收益率、滚动收益率与债券回报的关系
Investors often want to make quick "back-of-the-envelope" calculations with spot rates, forward rates and bond returns. In this appendix, we discuss some simple relations between these variables, beginning with a useful approximate relation between spot rates and one-year forward rates. These relations are discussed in more detail in the appendix of Market's Rate Expectations and Forward Rates. Equation (2) showed exactly how the forward rate between years m and n is related to m- and n-year spot rates. Equation (8) shows the same relation in an approximate but simpler form; this equation ignores nonlinear effects such as the convexity bias. The relation is exact if spot rates and forward rates are continuously compounded.
投资者往往想用即期收益率、远期收益率和债券回报进行快速的“推测”计算。在本附录中,我们从即期收益率和一年期远期收益率之间的近似关系开始,讨论这些变量之间的一些简单关系。这些关系在《市场收益率预期和远期收益率》的附录中更详细地讨论。等式(2)显示了m年和n年之间的远期收益率如何与m年和n年即期收益率相关。等式(8)以更简单的近似形式反映了相同的关系,这个等式忽略了诸如凸度偏差等非线性效应。如果即期收益率和远期收益率是连续复利的,那么这种关系是确切的。
For one-year forward rates (m = n-1), Equation (8) can be simplified to
对于一年期的远期收益率(m = n-1),等式(8)可以简化为
Equation (9) shows that the forward rate is equal to an n-year zero's one-year horizon return given an unchanged yield curve scenario: a sum of the initial yield and the rolldown return (the zero's duration at horizon (n-1) multiplied by the amount the zero rolls down the yield curve as it ages). This horizon return is often called the rolling yield. Thus, the one-year forward rates proxy for near-term expected returns at different parts of the yield curve if the yield curve is expected to remain unchanged. We can gain intuition about the equality of the one-year forward rate and the rolling yield by examining the n-year zero's realized holding-period return (h_n) over the next year, in Equation (10). The zero earns its initial yield (s_n) plus a capital gain/loss, which is approximated by the product of the zero's year-end duration and its realized yield change.
方程(9)表明,在收益率曲线不变的情况下,远期收益率等于n年零息债券的一年期平均回报:初始收益率和下滑回报(零息债券在持有期末的久期(n-1)乘以零息债券随期限减少而在收益率曲线上下滑的量)。这个期间平均回报通常被称为滚动收益率。因此,如果收益率曲线预期保持不变,一年期远期收益率代表收益率曲线不同部分的近期预期回报。在等式(10)中,我们可以通过检查下一年持有期零息债券的实现回报(h_n)获得关于一年期远期收益率和滚动收益率相等关系的直观观察。零息债券赚取其初始收益率(s_n)加上资本回报/损失,这由零息债券的年终久期和其实现收益率的变化的乘积近似。
where (s^*_{n-1}) is the n-1 year spot rate next year. If the yield curve follows a random walk, the best forecast for (s^*_{n-1}) is (today's) (s_{n-1}). Therefore, the n-year zero's expected holding period return equals the one-year forward rate in Equation (9). The key question is whether it is more reasonable to assume that the current spot rates are the optimal forecasts of future spot rates than to assume that forwards are the optimal forecasts. We present later empirical evidence which shows that the "random walk" forecast of an unchanged yield curve is more accurate than the forecast implied by the forwards.
其中(s^*_n)是次年的n-1年期即期收益率。如果收益率曲线遵循随机游走,则对于(s^*_{n-1})的最佳预测是(当前的)(s_{n-1})。因此,n年零息债券的预期持有期回报等于等式(9)中的一年期远期收益率。关键问题是,假设当前即期收益率是未来即期收益率的最佳预测,还是假设远期收益率是最佳预测。我们后来提出的实证证据表明,一个不变的收益率曲线的“随机游走”预测比基于远期收益率的预测更准确。
Equation (9) shows that the (one-year) forward rate curve lies above the spot curve as long as the latter is upward sloping (and the rolldown return is positive). Conversely, if the spot curve is inverted, the rolldown return is negative, and the forward rate curve lies below the spot curve. If the spot curve is first rising and then declining, the forward rate curve crosses it from above at its peak. Finally, the forward rate curve can become downward sloping even when the spot curve is upward sloping, if the spot curve's slope is first steep and then flattens (reducing the rolldown return). The calculations below illustrate this point and show that the approximation is good —— within a few basis points from the correct values (10.20-13.07-14.36-13.77) in Figure 9:
方程(9)表明,(一年期)远期收益率曲线位于即期收益率曲线上方,只要后者是向上倾斜(下滑回报是正的)。相反,如果即期收益率曲线倒挂,则下滑回报是负的,并且远期收益率曲线位于即期收益率曲线的下方。如果即期收益率曲线首先上升然后下降,则远期收益率曲线在其峰值处从上方与其交叉。最后,如果即期收益率曲线的斜率首先陡然后平坦(减小下降返回),则即使当即期收益率曲线向上倾斜时,远期收益率曲线也可以变得向下倾斜。下面的计算说明了这一点,并且在图9中显示出了良好的近似——只有几个基点内的偏差(10.20-13.07-14.36-13.77):
This overview will contain few references to earlier studies, but later reports in this series will provide a guide to academic and practitioner literature for interested readers. 本概述将很少提及早期的研究,但本系列的后续报告将为有兴趣的读者提供学术和实践文献的指南。 ↩︎
Arbitrage activities ensure that a bond's present value is similar when its cash flows are discounted using the marketwide spot rates as when its cash flows are discounted using the bonds own yield to maturity. However, some deviations are possible because of transaction costs and other market imperfections. In other words, the term structure of spot rates gives a consistent set of discount rates for all government bonds, but all bonds' market prices are not exactly consistent with these discount rates. Individual bonds may be rich or cheap relative to the curve because of bond-specific liquidity, coupon, tax, or supply effects. For example, the Salomon Brothers Government Bond Strategy Group reports daily each bond's spread off the estimated Treasury Model curve. (Because cheapness appears to persist over time, many investors prefer to use the Model spread relative to its own history as a relative value indicator.)
套利活动确保债券的现金流使用即期收益率贴现时的现值相似于使用债券自身的到期收益率贴现的现值。然而,由于交易成本和其他市场缺陷,有些偏差是可能的存在的。换句话说,即期收益率的期限结构为所有政府债券提供了一套一致的贴现率,但是所有债券的市场价格与这些贴现率并不完全一致。由于债券特定的流动性、票息率、税收或供给因素,特定债券可能相对于曲线来说是高估或低估的。例如,Salomon Brothers政府债券组每日报告每笔债券关于国债模型曲线的利差。(因为随着时间的推移,低估持续存在,许多投资者喜欢使用模型利差相对于其自身的历史数据变化作为相对价值指标。) ↩︎These curves can be computed directly by interpolating between on-the-run bond yields (approximate par curve) or between zero yields (spot curve). Because these assets have special liquidity characteristics, these curves may not be representative of the broad Treasury market. Therefore, the par, spot or forward rate curve is typically estimated using a broad universe of coupon Treasury bond prices. Many different "curve fitting" techniques exist, but a common goal is to fit the prices well with a reasonably shaped curve. This report does not focus on yield curve estimation but on the interpretation and practical uses of the curve once it has been estimated.
这些曲线可以通过在当前的收益率(近似票面收益率曲线)之间或零息债券收益率(即期收益率曲线)之间进行内插来直接计算。由于这些资产具有特殊的流动性特征,这些曲线可能并不代表国债市场。因此,通常使用一揽子的国债价格来估计票面、即期和远期收益率曲线。存在许多不同的“曲线拟合”技术,但是一个共同的目标是使价格适当地与合理的曲线拟合。本报告并不侧重于收益率曲线估计,而是对估计出的曲线的解释和实际应用。 ↩︎Further, one can use today's spot rates and Equation (2) to back out implied spot curves for any future date and implied future paths for the spot rate of any maturity. It is important to distinguish the implied spot curve one year forward ((f_{1,2}), (f_{1,3}), (f_{1,4}), ...), a special case of Equation (2) in which in m = 1 from the constant maturity one-year forward rate curve ((f_{1,2}), (f_{2,3}), (f_{3,4}), ...). Today's spot curve can be subtracted from the former curve to derive the yield changes implied by the forwards. (This terminology is somewhat misleading because these "implied" forward curves/paths do not reflect only the market‘s expectations of future rates)
此外,可以使用当前的即期收益率和公式(2)来算出任何未来日期的隐含即期收益率曲线以及任何期限的即期收益率的未来路径。注意区分一年后的隐含即期曲线和一年期远期收益率曲线,前者这是方程(2)的一个特殊情况,其中m = 1。可以从前面的曲线中减去当前的即期曲线,以得出由远期收益率隐含的收益率变化。(由于这些“隐含的”远期收益率曲线或路径并不仅仅反映市场对未来收益率的预期,所以这种说法有点误导性) ↩︎Note that all one-year forward rates actually have a one-year maturity even though, in the x-axis of Figure 1, each forward rate's maturity refers to the final maturity. For example, the one-year forward rate between n-1 and n matures n years from today.
注意,在图1的x轴上,所有一年期远期收益率实际上都有一年的期限,每个远期收益率的期限都是指最终期限的。例如,n-1年和n年之间的一年期远期收益率(f_{n-1,n})从当前开始算还有n年。 ↩︎An alternative interpretation is also possible. Instead of viewing (f_{1,2}) as the break-even selling rate of the two-year zero in one year's time we can view it as the break-even reinvestment rate of the one-year zero over the second year. In the first case, we equate the uncertain one-year return of the two-year zero with the known return of a (horizon-matching) one-year zero. In the second case, we equate the uncertain two-year return of a roll-over-one-year-zeros strategy with the known return of a two-year zero. 另一种解释也是可能的。在一年的时间内,我们可以把(f_{1,2})看作是一年期零息债券的盈亏平衡再投资收益率,而不是将其视为两年期零息债券的盈亏平衡卖出收益率。在第一种情况下,我们将两年零息债券不确定的一年期回报与已知的(期限匹配)一年零息债券的回报相等。在第二种情况下,我们将一年期零息债券滚动策略的不确定回报与已知的两年期零息债券回报相等。 ↩︎
A concave shape means that the (upward is loping) yield curve is sleeper at the front end than at the long end. The yield loss of moving from the two-year bond to cash produces a greater yield loss than the yield gain achieved by moving from the two-year bond 10 the ten-year bond. Thus, the yield earned from the combination of cash and tens is lower than the foregone yield from twos. 上凸形状意味着(向上倾斜)收益率曲线在前端比在后端陡峭。从两年期债券转为现金所损失的收益率比从两年期债券转为10年期债券所获得的收益率更大。因此,现金和10年期债券组合的收益率低于两年期债券的收益率。 ↩︎
Part 2 of this series. Market's Rate Expectations and Forward Rates discusses these issues in detail.
报告的第二部分,《市场收益率预期与远期收益率》详细讨论了这些内容 ↩︎The empirical bond risk premia are computed based on monthly returns of various maturity-subsector portfolios of Treasury bills or bonds between 1970 and 1994. This period does not have an obvious bearish or bullish bias because long-term yields were at roughly similar level in the end of 1994 as they were in the beginning of 1970. Figure 3 lots arithmetic average annual returns on average durations. The geometric average returns would be a bit lower and the curve would be essentially flat after two years.
实证债券风险溢价是根据1970年至1994年期间不同期限的国库券或国债投资组合的月回报计算的。这一时期没有明显的牛市或熊市偏差,因为长期收益率在1994年底与1970年初相近。图3绘制了算术平均年度回报关于平均久期的关系。几何平均回报将略低一点,曲线将在两年后基本上保持水平。 ↩︎Parts 3 and 4 of this series describe the empirical behavior of the bond risk premia. Does Duration Extension Enhance Long-Term Expected Returns? focuses on the long-run average return differentials across bonds with different maturities. Forecasting US Bond Returns focuses on the near-term expected return differentials across bonds and on the time-variation in the bond risk premia.
本系列的第3和4部分描述了债券风险溢价的经验行为。《久期增加会提高长期预期回报吗?》着重于不同期限债券的长期平均回报差异。《预测美国债券回报》的重点是债券近期预期回报差异和债券风险溢价的时变性。 ↩︎The degree of convexity varies across bonds, mainly depending on their option characteristics and durations. Embedded short options decrease convexity. For bonds without embedded options, convexity increases roughly as a square of duration (see Figure 4 top panel). There also are convexity differences between bonds that have the same duration. A barbell position (with very dispersed cash flows) exhibits more convexity than a duration-matched bullet bond. The reason is that a yield rise reduces the relative weight of the barbell's longer cash flows (because their present values decline more than those of the shorter cash flows). shortening the barbell's duration. The inverse relation between duration and yield level increases a barbell's convexity, limiting its losses when yields rise and enhancing its gains when yields decline. Of all bonds with the same duration, a zero has the smallest convexity because its cash flows are not disperse; thus, its Macaulay duration does not vary with the yield level.
凸度因债券而异,主要取决于期权特征和久期。嵌入的看跌期权会减少凸度。对于没有嵌入期权的债券,凸度大致按久期的平方增加(见图4上半部分)。在具有相同久期的债券之间也存在凸度差异。杠铃组合(具有非常分散的现金流)比久期匹配的子弹组合具有更大的凸度。原因是收益率上升降低了杠铃组合长端现金流的相对比重(因为它们的现值比较短端的现金流贴现得更多),这缩短了杠铃组合的久期。久期和收益率水平之间的反比关系增加了杠铃组合的凸度,限制了当收益率上升时的损失,并在收益率下降时增加回报。在所有久期相同的债券中,由于现金流不分散,所以零息债券的凸度最小,因此,Macaulay久期并不随收益率水平而变化。 ↩︎Convexity bias is closely related to the distinction between different versions of the pure expectations hypothesis. Above, we referred to the pure expectations hypothesis. In fact, alternative versions of this hypothesis exist that are not exactly consistent with each other. The local expectations hypothesis (LEH) assumes that "all bonds earn the same expected return over the next period" while the unbiased expectations hypothesis (UEH) assumes that "forward rates equal expected spot rates.” In Figure 4 (lower panel), the LEH is assumed to hold; thus, UEH is not exactly true. The expected future short rates are flat at 8% even though the curve of one-year forward rates is inverted. In yield terms, the difference between the LEH and the UEH is the convexity bias.
凸度偏差与完全预期假说不同版本之间的区别密切相关。现在开始,我们指的是之前提到的完全预期假说。事实上,存在与这种假说不完全一致的替代版本。局部预期假说(LEH)假设“所有债券在下一期间获得相同的预期回报”,而无偏预期假说(UEH)假设“远期收益率等于预期的即期收益率”。在图4(下半部分)中,假设LEH是成立的,UEH并不完全正确。即使一年期远期收益率曲线倒挂,预期的未来短期收益率也是8%。到期收益率方面,LEH和UEH之间的差异就是凸度偏差。 ↩︎Part 5 of this series, Convexity Bias and the Yield Curve, discusses these topics in more detail.
报告第五部分,《凸度偏差与收益率曲线》详细讨论了这些问题 ↩︎In later papers, we will show how interest rate expectations can he measured using survey data, how bond risk premia can be estimated using historical return data and how the convexity bias can be inferred using option prices. Alternatively, all three components can be estimated from the yield curve if one is willing to impose the structure of some term structure model (with its possibly unrealistic assumptions).
在后面的报告中,我们将展示如何利用调查数据衡量收益率预期,如何使用历史回报数据估算债券风险溢价,以及如何使用期权价格推导凸度偏差。或者,如果愿意强加一些期限结构模型的结构(其可能不切实际的假设),则可以从收益率曲线估计所有三个组成部分。 ↩︎A related assertion claims that if near-term expected returns were not equal across bonds, it would imply the existence of riskless arbitrage opportunities. This assertion is erroneous. It is true that it forward contracts were traded assets, arbitrage forces would require their pricing to be consistent with zero prices according to Equation (2). However, the arbitrage argument says nothing about the economic determinants of the zero prices themselves, such as rate expectations or risk premia. The bond market's performance in 1994 shows that buying long-term bonds is not riskless even if they have higher expected returns than short-term bonds.
相关论断声称,如果近期预期回报在债券之间不相等,则意味着存在无风险的套利机会。这个断言是错误的。诚然,远期合约是交易资产,套利力量将根据公式(2)要求其定价与零息债券价格一致。然而,套利并没有说明零息债券价格本身的经济决定因素,如收益率预期或风险溢价。1994年债券市场的表现显示,即使购买长期债券的预期回报比短期债券更高,购买长期债券也不是无风险的。 ↩︎Note that the first point in each implied forward par curve in Figure 5 is the implied forward three-month rate at a given future date. Therefore, the forward path in Figure 6 can be constructed by tracing through the three-month points in the three curves of Figure 5 and through similar curves at other horizons. Because Figure 6 depicts a rate path over time, the x-axis is calendar years and not maturity.
请注意,图5中每个隐含的远期曲线中的第一点是在给定未来日期的隐含的三月期远期收益率。因此,图6中的远期收益率路径可以通过跟踪图5的三条曲线中的三月期点和其他期限中的类似曲线来构建。因为图6描绘了随时间的收益率路径,所以x轴是历年,而不是期限。 ↩︎Forward rates are also very low at the long maturities, but this characteristic probably reflects the convexity bias. Forward rates are downward-biased estimates of expected returns because they ignore the convexity advantage which is especially large at long maturities.
远期收益率通常在很长的期限上很低,但这种特征可能反映了凸度偏差。远期收益率是预期回报的下偏估计,因为它们忽视长期限的凸度优势(通常特别大)。 ↩︎As bonds age, they roll down the upward-sloping yield curve and earn some rolldown return (capital gain due to this yield change) if the yield curve remains unchanged. A bond's rolling yield, or horizon return includes the yield and the rolldown return given a scenario of no change in the yield curve. 随着债券临近到期,如果收益率曲线保持不变,收益率会沿着上倾斜的收益率曲线下滑,并获得下滑回报(由于该收益率变化而产生的资本回报)。收益率曲线中没有变化的情况下,债券的滚动收益率或持有期回报包括收益率和下滑回报。 ↩︎
The one-period forward rate can proxy for the near-term expected return —— albeit with a downward-bias because it ignores the value of convexity —— if the current yield curve is not expected to change. Empirical studies show that the assumption of an unchanged curve is more realistic than the assumption that forward rates reflect expected future yields. Historically current spot rates predict future spot rates better than current forward rates do because the yield changes implied by the forwards have not been realized, on average.
如果目前的收益率曲线预期不会改变,一(年)期远期收益率可以代指近期预期回报,尽管存在下偏(因为它忽略了凸度的值)。实证研究表明,假设一个不变的曲线比远期收益率反映预期未来收益率的假设更为接近现实。历史上,当前即期收益率比远期收益率能更好的预测未来即期收益率,因为远期收益率隐含的收益率变化平均来看并没有实现。 ↩︎Part 4 of this series, Forecasting US Bond Returns, evaluates the historical performance of dynamic strategies that exploit the predictability of long-term bonds' near-term returns. The dynamic strategies have consistently outperformed static strategies that do not actively adjust the portfolio duration.
本系列第4部分,《预测美国债券回报》评估了利用长期债券近期回报可预测性的动态策略的历史表现。动态策略始终优于没有积极调整投资组合久期的静态策略。 ↩︎