1066. Root of AVL Tree (25)

An AVL tree is a self-balancing binary search tree. In an AVL tree, the heights of the two child subtrees of any node differ by at most one; if at any time they differ by more than one, rebalancing is done to restore this property. Figures 1-4 illustrate the rotation rules.

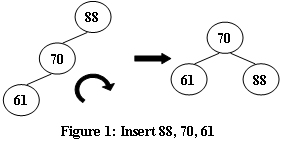
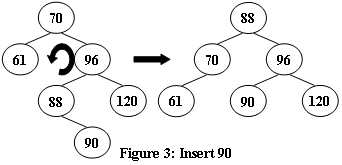
Now given a sequence of insertions, you are supposed to tell the root of the resulting AVL tree.

Input Specification:

Each input file contains one test case. For each case, the first line contains a positive integer N (<=20) which is the total number of keys to be inserted. Then N distinct integer keys are given in the next line. All the numbers in a line are separated by a space.

Output Specification:

For each test case, print ythe root of the resulting AVL tree in one line.

Sample Input 1:

5
88 70 61 96 120

Sample Output 1:

Sample Input 2:

7
88 70 61 96 120 90 65

Sample Output 2:

  1 #include<stdio.h>
  2 #include<vector>
  3 #include<algorithm>
  4 using namespace std;
  5 
  6 struct node
  7 {
  8     node(int v):left(NULL),right(NULL),high(1),val(v){}
  9     node* left,* right;
 10     int val,high;
 11 };
 12 
 13 int gethigh(node* root)
 14 {
 15     int a = 0, b = 0;
 16     if(root->left!= NULL)
 17         a = root->left->high;
 18     if(root->right!= NULL)
 19         b = root->right->high;
 20     return a > b ? a+1:b+1;
 21 }
 22 
 23 void R(node* & root)
 24 {
 25     node* tem = root->left;
 26     root->left = tem->right;
 27     tem->right = root;
 28     root->high = gethigh(root);
 29     tem->high = gethigh(tem);
 30     root = tem;
 31 }
 32 
 33 void L(node* & root)
 34 {
 35     node* tem = root->right;
 36     root->right = tem->left;
 37     tem->left = root;
 38     root->high = gethigh(root);
 39     tem->high = gethigh(tem);
 40     root = tem;
 41 }
 42 
 43 void insert(node*& root,int val)
 44 {
 45     if(root == NULL)
 46     {
 47         root = new node(val);
 48         return;
 49     }
 50 
 51     if(val < root->val)
 52     {
 53         insert(root->left,val);
 54         root->high = gethigh(root);
 55         int a = root->left == NULL ? 0 : root->left->high;
 56         int b = root->right == NULL ? 0 : root->right->high;
 57         if(a - b == 2)
 58         {
 59             int c = root->left->left == NULL ? 0:root->left->left->high;
 60             int d = root->left->right == NULL ? 0:root->left->right->high;
 61             if(c - d  == 1 )
 62             {
 63                 R(root);
 64             }
 65             else if(c - d  == -1)
 66             {
 67                 L(root->left);
 68                 R(root);
 69             }
 70         }
 71     }
 72     else
 73     {
 74         insert(root->right,val);
 75         root->high = gethigh(root);
 76         int a = root->left == NULL ? 0 : root->left->high;
 77         int b = root->right == NULL ? 0 : root->right->high;
 78         if(a - b == -2)
 79         {
 80             int c = root->right->right == NULL ? 0:root->right->right->high;
 81             int d = root->right->left == NULL ? 0:root->right->left->high;
 82             if(c - d == 1)
 83             {
 84                 L(root);
 85             }
 86             else if(c - d == -1)
 87             {
 88                 R(root->right);
 89                 L(root);
 90             }
 91         }
 92     }
 93 }
 94 
 95 int main()
 96 {
 97     int n,tem;
 98     scanf("%d",&n);
 99     node* Tree = NULL;
100     for(int i = 0;i < n;++i)
101     {
102         scanf("%d",&tem);
103         insert(Tree,tem);
104     }
105     printf("%d
",Tree->val);
106     return 0;
107 }

相关阅读:
软解析和硬解析
 oracle存储过程常用技巧
 jquery-1.11.1.js
JavaScript遍历table
JavaScript向select下拉框中添加和删除元素
 glog
DDL引发的对象invalidation
模拟cursor pin S wait on X
rsync 排除目录
 JavaScript解决select下拉框中的内容太长显示不全的问题
原文地址：https://www.cnblogs.com/xiaoyesoso/p/5248938.html