You are given the root node of a binary search tree (BST) and a value to insert into the tree. Return the root node of the BST after the insertion. It is guaranteed that the new value does not exist in the original BST.
Notice that there may exist multiple valid ways for the insertion, as long as the tree remains a BST after insertion. You can return any of them.
Example 1:
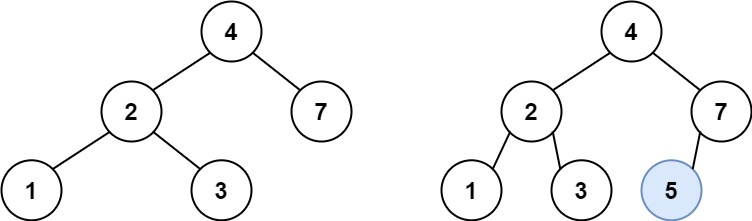
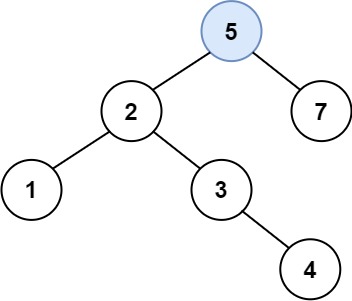
Input: root = [4,2,7,1,3], val = 5 Output: [4,2,7,1,3,5] Explanation: Another accepted tree is:
Example 2:
Input: root = [40,20,60,10,30,50,70], val = 25 Output: [40,20,60,10,30,50,70,null,null,25]
Example 3:
Input: root = [4,2,7,1,3,null,null,null,null,null,null], val = 5 Output: [4,2,7,1,3,5]
Constraints:
- The number of nodes in the tree will be in the range
[0, 104]. -108 <= Node.val <= 108- All the values
Node.valare unique. -108 <= val <= 108- It's guaranteed that
valdoes not exist in the original BST.
class Solution { public TreeNode insertIntoBST(TreeNode root, int val) { if(root == null) return new TreeNode(val); TreeNode cur = root; while(cur != null) { if(cur.val < val) { if(cur.right == null) { cur.right = new TreeNode(val); break; } else cur = cur.right; } else { if(cur.left == null) { cur.left = new TreeNode(val); break; } else cur = cur.left; } } return root; } }
屮,把bst和大小堆搞混了,以为会很难。iterative way就遍历,如果insert的数大于根,就往右走,如果右是空就直接新建node,如果不是,就进入它的right再次对比val。
如果小于等于根,就往左走,和上面的判断一样。最后返回root
class Solution { public TreeNode insertIntoBST(TreeNode root, int val) { if(root == null) return new TreeNode(val); if(root.val < val) { root.right = insertIntoBST(root.right, val); } else root.left = insertIntoBST(root.left, val); return root; } }
recursive way。