Problem Description
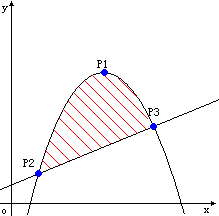
Ignatius bought a land last week, but he didn't know the area of the land because the land is enclosed by a parabola and a straight line. The picture below shows the area. Now given all the intersectant points shows in the picture, can you tell Ignatius the area of the land?
Note: The point P1 in the picture is the vertex of the parabola.
Input
The input contains several test cases. The first line of the input is a single integer T which is the number of test cases. T test cases follow.
Each test case contains three intersectant points which shows in the picture, they are given in the order of P1, P2, P3. Each point is described by two floating-point numbers X and Y(0.0<=X,Y<=1000.0).
Output
For each test case, you should output the area of the land, the result should be rounded to 2 decimal places.
Sample Input
2 5.000000 5.000000 0.000000 0.000000 10.000000 0.000000 10.000000 10.000000 1.000000 1.000000 14.000000 8.222222
Sample Output
33.33 40.69
Hint
For float may be not accurate enough, please use double instead of float.
设y=a*(x-b)^2+c
y=ax^2-2abx+ab^2+c
积分后1/3ax^3-abx^2+(ab^2+c)x
jfp(double xu,double xd)计算抛物线与x轴之间的面积
jfx(double xu,double xd)计算直线与x轴之间的面积
然后将上下限带入相减就得到最后阴影面积。
今天发现自己状态很不对,做了两小时都WA,经Chris检验,这个思路当然对。。但为什么答案不对?
捣鼓了半天,想着把下面两点和x轴组成的面积用梯形公式求,但输出错误的结果跟用积分求相同,那就断定,求下面面积的公式正确。最后功夫不负有心人,chris发现了我竟然有把jfp写成如下形式
double jfp(double xu,double xd){
double ux = a*xu*xu*xu/3 - a*b*xu*xu + (a*b*b+c)*xu;
double dx = a*xu*xu*xd/3 - a*b*xu*xd + (a*b*b+c)*xd;
return ux-dx;
}
看出来了吧,复制粘贴罪大恶极,以后想不想再粘了!!!!????
#include<iostream>
#include<iomanip>
#include<cmath>
using namespace std;
double a,b,c,k,b2;
double jfp(double xu,double xd){
double ux = a*xu*xu*xu/3 - a*b*xu*xu + (a*b*b+c)*xu;
double dx = a*xd*xd*xd/3 - a*b*xd*xd + (a*b*b+c)*xd;
return ux-dx;
}
double jfx(double xu,double xd){
double ux = 0.5*k*xu*xu+b2*xu;
double dx = 0.5*k*xd*xd+b2*xd;
return ux-dx;
}
double y(double x){
return k*x + b2;
}
void main(){
int T;
cin>>T;
while(T>0){
double x1,x2,x3,y1,y2,y3;
cin>>x1>>y1>>x2>>y2>>x3>>y3;
a = (y2-y1)/((x2-x1)*(x2-x1));
b = x1;
c = y1;
k = (y3-y2)/(x3-x2);
b2 = y2-k*x2;//-(y(x2)+y(x3))*(x3-x2)/2.0;//
double area = jfp(x3,x2)-jfx(x3,x2);
cout.setf(ios::fixed);
cout.precision(2);
cout<<area<<endl;
T--;
}
}