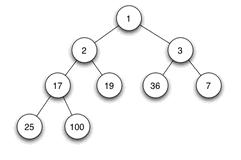
二叉堆是完全二叉树或者是近似完全二叉树。二叉堆满足二个特性:1.父结点的键值总是大于或等于(小于或等于)任何一个子节点的键值。2.每个结点的左子树和右子树都是一个二叉堆(都是最大堆或最小堆)。当父结点的键值总是大于或等于任何一个子节点的键值时为最大堆。当父结点的键值总是小于或等于任何一个子节点的键值时为最小堆。下图展示一个最小堆:
由于其它几种堆(二项式堆,斐波纳契堆等)用的较少,一般将二叉堆就简称为堆。
堆的存储一般都用数组来表示堆,i结点的父结点下标就为 i / 2。它的左右子结点下标分别为2 * i 和 2 * i +1

堆排序 代码如下 : 要领 : 建堆->堆排->堆化[维持]。
#include <iostream>
#include <string>
#include <cstring>
#include <cstdlib>
#include <cstdio>
#include <cmath>
#include <vector>
#include <stack>
#include <deque>
#include <queue>
#include <bitset>
#include <list>
#include <map>
#include <set>
#include <iterator>
#include <algorithm>
#include <functional>
#include <utility>
#include <sstream>
#include <climits>
#include <cassert>
#define BUG puts("here!!!");
using namespace std;
void heapify(int a[], int i, int size) { // 堆化的维持需要用递归
int ls = 2*i, rs = 2*i + 1;
int large = i;
if(ls <= size && a[ls] > a[i]) {
large = ls;
}
if(rs <= size && a[rs] > a[large]) {
large = rs;
}
if(large != i) {
swap(a[i], a[large]);
heapify(a, large, size);
}
}
void buildHeap(int a[], int size) {
for(int i = size/2; i >= 1; i--) {
heapify(a, i, size);
}
}
void heapSort(int a[], int size) {
buildHeap(a, size);
int len = size;
for(int i = len; i >= 2; i--) {
swap(a[i], a[1]);
len--;
heapify(a, 1, len);
}
}
int main() {
int a[] = {0, 8, 5, 4, 9, 2, 3, 6}; // 测试用例是 : 8, 5, 4, 9, 2, 3, 6
heapSort(a, 7);
for(int i = 1; i <= 7; i++) {
cout << a[i] << ' ';
}
return 0;
}