算法秦九韶算法

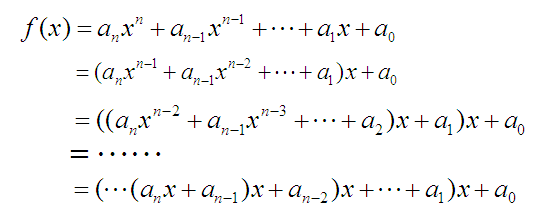
介绍

秦九韶算法又叫霍纳算法。一般地，一元n次多项式的求值需要经过[n（n+1）]/2次乘法和n次加法，而秦九韶算法只需要n次乘法和n次加法。在人工计算时，一次大大简化了运算过程。

Pn(x)= anx ^n+a(n－1)x^(n-1)+…+a1x+a0

可简化成

Pn(x)= anx ^n+a(n－1)x^(n-1)+…+a1x+a0=((…(((anx +an－1)x+an－2)x+ an－3)…)x+a1)x+a0

java实现

  1 package com.qyf404.learn.algorithm;
  2 
  3 import java.math.BigDecimal;
  4 
  5 /**
  6  * 
  7  秦九韶算法又称霍纳算法。 一般地，一元n次多项式的求值需要经过[n（n+1）]/2次乘法和n次加法，
  8  * 而秦九韶算法只需要n次乘法和n次加法。在人工计算时，一次大大简化了运算过程。
  9  * 
 10  * Pn(x)= anx ^n+a(n－1)x^(n-1)+…+a1x+a0
 11  * 
 12  * 可简化成
 13  * 
 14  * Pn(x)= anx ^n+a(n－1)x^(n-1)+…+a1x+a0=((…(((anx +an－1)x+an－2)x+
 15  * an－3)…)x+a1)x+a0
 16  * 
 17  * @author qyfmac
 18  */
 19 public class HornerAlgorithm {
 20     private double a[];
 21     private Double x;
 22 
 23     public HornerAlgorithm(double[] a, double x) {
 24         this.a = a;
 25         this.x = x;
 26     }
 27     public void check(){
 28         if(a == null || x == null || a.length < 1 ){
 29             throw new RuntimeException();
 30         }
 31     }
 32     
 33     /**
 34      * 简单的for循环实现
 35      * 
 36      * 测试比较使用
 37      * @return
 38      */
 39     private double oldCompute() {
 40         check();
 41         double s = 0;
 42         for (int i = 0; i < a.length; i++) {
 43             s = s + Math.pow(x, i) * a[i];
 44         }
 45         return s;
 46     }
 47     /**
 48      * 简单的for循环实现
 49      * 
 50      * 测试比较使用
 51      * @return
 52      */
 53     private BigDecimal oldCompute2BigDecimal() {
 54         check();
 55         BigDecimal x = new BigDecimal(this.x);
 56         BigDecimal s = new BigDecimal(0);
 57         for (int i = 0; i < a.length; i++) {
 58             s = s.add(x.pow(i).multiply(new BigDecimal(a[i])));
 59         }
 60         return s;
 61     }
 62     
 63 
 64     /**
 65      * 秦九韶算法实现
 66      * 
 67      * @return
 68      */
 69     public double compute() {
 70         check();
 71         
 72         int n = a.length -1;
 73         double s = a[n];
 74         
 75         if(n == 0){
 76             //f(x)=a0 的情况
 77             return s;
 78         }
 79         
 80         int i = 0;
 81         do{
 82             i++;
 83             s = a[n-i] + x * s;
 84             
 85         }while(i < n);
 86         
 87         
 88         return s;
 89     }
 90     /**
 91      * 秦九韶算法实现
 92      * 
 93      * @return
 94      */
 95     public BigDecimal compute2BigDecimal() {
 96         check();
 97         
 98         int n = a.length -1;
 99         BigDecimal s = new BigDecimal(a[n]);
100         
101         if(n == 0){
102             //f(x)=a0 的情况
103             return s;
104         }
105         BigDecimal x = new BigDecimal(this.x);
106         int i = 0;
107         do{
108             i++;
109             s = new BigDecimal(a[n-i]).add(s.multiply(x));
110             
111         }while(i < n);
112         
113         
114         return s;
115     }
116 
117     public static void main(String[] args) {
118 //        double a[] ={1};
119 //        double a[] ={1,1};
120 //        double a[] ={1,1,1};
121 //        double a[] ={1,1,1,2};
122         double a[] = { 1 ,111.3 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,111 ,11};
123         double x = 2;
124         HornerAlgorithm ha = new HornerAlgorithm(a, x);
125 
126         {
127             long start = System.currentTimeMillis();
128             BigDecimal s = ha.oldCompute2BigDecimal();
129             long end = System.currentTimeMillis();
130             System.out.println("耗时" + (end - start) + "结果为" + s);
131         }
132         {
133             long start = System.currentTimeMillis();
134             BigDecimal s = ha.compute2BigDecimal();
135             long end = System.currentTimeMillis();
136             System.out.println("耗时" + (end - start) + "结果为" + s);
137         }
138 
139     }
140 }

最终测试结果

1 耗时22结果为1139953162956611208548808572713851989272522501907096190014794725752241296178091297082821351384513148463092452900820004233019437006863981436491416946700513548219890381356958359367906614177967433056035.5999999999999943156581139191985130310058593750
2 耗时3结果为1139953162956611208548808572713851989272522501907096190014794725752241296178091297082821351384513148463092452900820004233019437006863981436491416946700513548219890381356958359367906614177967433056035.5999999999999943156581139191985130310058593750

结论

在n很大的时候，常规for循环时间复杂度O(n^2),而秦九韶算法时间复杂度O(2n)。

作者：辵鵵

出处：http://qyf404.cnblogs.com

欢迎转载，但未经作者同意请保留此段声明，并在文章页面明显位置给出原文链接。

相关阅读:
Chrome浏览器M53更新后超链接的dispatchEvent(evt)方法无法触发文件下载
 用es5实现模板字符串
 JS求数组最大值常用方法
 js生成随机数
 常用MouseEvent鼠标事件对象&KeyboardEvent键盘事件对象&常用键盘码
 原生js重写each方法
 indexdb开cai发keng实zhi践lu
substring和substr的区别和使用
 前端常见面试题总结part2
前端常见面试题总结1
原文地址：https://www.cnblogs.com/qyf404/p/3971800.html

算法 秦九韶算法

介绍

java实现

结论

算法秦九韶算法