上一帖已经说过了大概的设计,第一步我们是要把输入的正则式构建成抽象模式树,我们先定义表示模式树的类。
public enum Releation
{
Default,
Or,
And
}
public class PatternNode {
public PatternNode()
:this(null)
{
}
public PatternNode(string text) {
Text = text;
Nodes = new List<PatternNode>();
OneOrMore = false;
Releation = Releation.Default;
}
public List<PatternNode> Nodes { get; set; }
public string Text { get; set; }
public bool OneOrMore { get; set; }
public Releation Releation{get;set;}
}
基本不用解释,属于一个仅用于封装数据的类,大家可以针对命名和代码布局风格挑一些刺儿。难点在于根据正则式解析成模式树,我是这样做的:按理说这种复杂逻辑的实现,应该先写伪代码,在脑子里演练一番算法,然后再写真正的目标代码,可我之前写的伪代码没保留下来,就说说思路吧,代码如下:
public static PatternNode Parse(string input) {
PatternNode resultNode = new PatternNode(input);
int index = 0;
int leftParenthesisCount = 0;
List<string> childNodeStr = new List<string>();
StringBuilder currentScanStr = new StringBuilder();
while (index < input.Length) {
char currentChar = input[index];
switch (currentChar) {
case '|':
if (leftParenthesisCount == 0) {
if (currentScanStr.Length > 0) {
childNodeStr.Add(currentScanStr.ToString());
currentScanStr.Remove(0, currentScanStr.Length);
}
}
else {
currentScanStr.Append(currentChar);
}
break;
case '(':
leftParenthesisCount++;
currentScanStr.Append(currentChar);
break;
case ')':
leftParenthesisCount--;
currentScanStr.Append(currentChar);
if (index + 1 < input.Length && input[index + 1] == '*') {
currentScanStr.Append('*');
index++;
}
break;
default:
currentScanStr.Append(currentChar);
break;
}
index++;
}
if (leftParenthesisCount != 0) {
throw new ApplicationException("括号不匹配");
}
if (currentScanStr.Length > 0) {
childNodeStr.Add(currentScanStr.ToString());
currentScanStr.Remove(0, currentScanStr.Length);
}
if (childNodeStr.Count > 1) { //本级有or关系,如“a|b”
childNodeStr.ForEach((str) => resultNode.Nodes.Add(new PatternNode(str)));
resultNode.Releation = Releation.Or;
resultNode.Nodes.ForEach((pattNode) =>
ProcessAndReleation(pattNode));
}
else {
ProcessAndReleation(resultNode);
}
return resultNode;
}
- 要解析输入的正则式肯定要从头向后便利输入的字符串,由于遍历的逻辑比较复杂,所以打算用while循环,比较灵活,声明变量index用来控制循环,while的条件是index < input.Length
- 考虑到小括号的影响,循环外要用一个变量leftParenthesisCount来对左括号进行计数,以检测出括号少括或者多括的错误正则式。
- 用一个StringBuild变量currentScanStr来存储遍历过程中的子模式,我们叫它字模式缓冲区
- 循环过程中主要是处理"|","(",")"这3个字符,在遇到|的时候,如果左括号的个数为0,并且子模式缓冲区有数据,就把当前子模式添加到子节点中。如果左括号计数大于0,那就把当前字符放到子模式缓冲区里,下一次递归解析会处理它,本次处理只处理本层的模式。
- 遇到(只要增加左括号计数并更新子模式缓冲区就行,但遇到)除了递减左括号计数器外,还需要考虑括号外有闭包的情况,把*也加到子模式缓冲区里。
- 每一个层次的解析循环完了后要检查左括号是否为0,如果不为0,说明少些了有括号,直接跑错。如果循环完了子模式缓冲区里还有数据,也要添加到子节点里。
- 遍历结束后,如果如果子节点数量大于1,那就说明本层的解析有或关系,就把本级节点的关系设置为or。可以看到循环的逻辑里是在遇到|的时候添加子节点的。
上面这个方法基本上是只处理或的关系,可以看到上面的函数里在没有扫描出或子节点时调用了ProcessAndReleation,该方法去尝试解析连接子节点,如下
private static void ProcessAndReleation(PatternNode pattNode) {
int index = 0;
string input = pattNode.Text;
StringBuilder currentScanStr = new StringBuilder();
int leftParenthesisCount = 0;
List<string> childNodeStr = new List<string>();
while (index < input.Length) {
char currentChar = input[index];
switch (currentChar) {
case '(':
//abc(de(fg))取出abc
if (leftParenthesisCount == 0 && currentScanStr.Length > 0) {
childNodeStr.Add(currentScanStr.ToString());
currentScanStr.Remove(0, currentScanStr.Length);
}
leftParenthesisCount++;
currentScanStr.Append(currentChar);
break;
case ')':
leftParenthesisCount--;
currentScanStr.Append(currentChar);
if (index + 1 < input.Length && input[index + 1] == '*') {
currentScanStr.Append('*');
index++;
}
//只有最顶层的括号闭合才取出来abc(de(fg))只取(de(fg)),不取(fg)
if (leftParenthesisCount == 0 && currentScanStr.Length > 0) {
childNodeStr.Add(currentScanStr.ToString());
currentScanStr.Remove(0, currentScanStr.Length);
}
break;
default:
currentScanStr.Append(currentChar);
break;
}
index++;
}
if (leftParenthesisCount != 0) {
throw new ApplicationException("括号不匹配");
}
if (currentScanStr.Length > 0) {
childNodeStr.Add(currentScanStr.ToString());
currentScanStr.Remove(0, currentScanStr.Length);
}
if (childNodeStr.Count > 1) {//本层有and关系,如"a(b|c)d"会分成a,(b|c),d
pattNode.Releation = Releation.And;
childNodeStr.ForEach((str) => pattNode.Nodes.Add(new PatternNode(str)));
pattNode.Nodes.ForEach(
(node) => {
if (node.Text.Contains('(')) {//子节点下可能还有or或者and关系,如"(b|c)"
var orNode = Parse(node.Text);
node.Nodes = orNode.Nodes;
node.Releation = orNode.Releation;
node.OneOrMore = node.Text.EndsWith("*");
}
else {//子节点是纯字符串,如"a"
//结束递归
}
});
}
else {
if (childNodeStr[0] == pattNode.Text) {//本层没有and关系,如"(b|c)","cd"
if (pattNode.Text.IndexOf('(') == 0) {//(ab)或者(a|b),最外层有括号
int toRemove = pattNode.Text.Length - 2;
if (pattNode.Text.EndsWith("*"))
{
toRemove--;
}
string qukuohao = pattNode.Text.Substring(1, toRemove);
var qukuohaoNode = Parse(qukuohao);
pattNode.Nodes = qukuohaoNode.Nodes ;
pattNode.Releation = qukuohaoNode.Releation;
pattNode.OneOrMore = pattNode.Text.EndsWith("*");
}
else //如"abc"
{
//结束递归
}
}
else {
//按理说不可能
System.Diagnostics.Debugger.Break();
}
}
}
说实在的,这个函数折腾时间最长,基本思路和处理或子节点类似,也是一个大循环,只不过处理的情况更复杂
- 遇到(时如果左括号为0,并且子模式缓冲区有数据,应该先把缓冲区添加到子节点,然后再把当前的字符放入缓冲区,比如abc(de(fg))取出abc
- 遇到)时同样要处理括号外有*的情况,但本层之处理本层的模式,所以要确保只有最顶层的括号闭合才取出来abc(de(fg))只取(de(fg)),不取(fg)。
- 循环结束后如果有子节点,说明本层有and关系,因为本函数是通过()来进行本层的分割,也就是只解析连接子节点,如果子节点里面如果有括号的话,肯定还有and和or的关系,所以要遍历子节点,递归调用Parse来解析本层的子模式。
- 如何本函数没有解析出and子节点,那说明本层要解析的模式字符串的最外层是括号括住的,要把最外层的括号去掉,然后递归调用Parse方法。
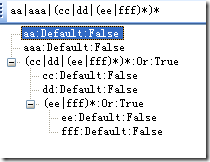
我们来测试一下,给定一个输入,把解析出来的模式树用treeview来表示。
private void showParseNode(PatternNode pattNode, TreeNodeCollection coll) {
if (pattNode.Nodes.Count == 0) {
coll.Add(pattNode.Text);
return;
}
for (int i = 0; i < pattNode.Nodes.Count; i++) {
PatternNode node = pattNode.Nodes[i];
TreeNode treeNode = new TreeNode(node.Text + ":"+node.Releation.ToString()
+":"+node.OneOrMore);
coll.Add(treeNode);
if (node.Nodes.Count != 0)
showParseNode(node,treeNode.Nodes);
}
}
截图如下,可以验证下这两个方法的执行结果是否正确,treeview的每个节点的表示是”模式字符串:子节点间关系:是否匹配多次”
这两个函数共同组成一个递归的调用,而且每个函数里的if else,while都不算少,而且while里还有break,我可以很肯定的预测,尽管这段代码我连写带调试费了好长时间,但这段代码里肯定有低级错误,或者有更清晰简单的写法,请大家来指教一下,或者自己完全重新写一个更优雅的。