Problem Description
You are given N positive integers, denoted as x0, x1 ... xN-1. Then give you some intervals [l, r]. For each interval, you need to find a number x to make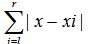 as small
as possible!
as small
as possible!
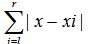 as small
as possible!
as small
as possible!Input
The first line is an integer T (T <= 10), indicating the number of test cases. For each test case, an integer N (1 <= N <= 100,000) comes first. Then comes N positive integers x (1 <= x <= 1,000, 000,000) in the next line. Finally,
comes an integer Q (1 <= Q <= 100,000), indicting there are Q queries. Each query consists of two integers l, r (0 <= l <= r < N), meaning the interval you should deal with.
Output
For the k-th test case, first output “Case #k:” in a separate line. Then output Q lines, each line is the minimum value of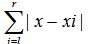 . Output a blank line after every test
case.
. Output a blank line after every test
case.
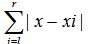 . Output a blank line after every test
case.
. Output a blank line after every test
case.Sample Input
2 5 3 6 2 2 4 2 1 4 0 2 2 7 7 2 0 1 1 1
Sample Output
Case #1: 6 4 Case #2: 0 0
Author
standy
Source
Recommend
zhengfeng | We have carefully selected several similar problems for you: 3474 1828 3397 3333 3472
被杭电的输出坑了 好久。。。。
被杭电的输出坑了 好久。。。。
printf lld 就WA 要I64d才行。。。。。真吭。!
!
!
易得使绝对值和最小就是中位数,能够參考坐标上的点到两点间距离之和最小的原理。
。
。
这道题让我对划分树的原理理解更加深刻了。
#include <iostream>
#include <cstdio>
#include <cstring>
#include <vector>
#include <string>
#include <algorithm>
#include <queue>
using namespace std;
#define md(x, y) (((x)+(y))>>1)
const int maxn = 100000+10;
typedef long long LL;
int n,m;
int ls;
int num[maxn];
int seg[20][maxn];
int lftnum[20][maxn];
LL lfts[20][maxn];
LL presum[maxn];
LL lsum;
void build(int L,int R,int dep){
if(L==R)return;
int mid = md(L,R);
int key = num[mid];
int lcnt = mid-L+1;
for(int i = L; i <= R; i++){
if(seg[dep][i] < key)
lcnt--;
}
int lp = L,rp = mid+1;
for(int i = L; i <= R; i++){
if(i==L){
lftnum[dep][i] = 0;
lfts[dep][i] = 0;
}else{
lfts[dep][i] = lfts[dep][i-1];
lftnum[dep][i] = lftnum[dep][i-1];
}
if(seg[dep][i] < key){
lftnum[dep][i]++;
lfts[dep][i] += seg[dep][i];
seg[dep+1][lp++] = seg[dep][i];
}
else if(seg[dep][i] > key){
seg[dep+1][rp++] = seg[dep][i];
}
else{
if(lcnt>0){
lcnt--;
lftnum[dep][i]++;
lfts[dep][i] += seg[dep][i];
seg[dep+1][lp++] = seg[dep][i];
}else{
seg[dep+1][rp++] = seg[dep][i];
}
}
}
build(L,mid,dep+1);
build(mid+1,R,dep+1);
}
LL query(int L,int R,int ll,int rr,int dep,int k){
if(ll == rr)
return seg[dep][ll];
int mid = md(ll,rr);
int ncnt,ucnt;
LL tsum = 0;
if(L==ll){
ncnt = 0;
tsum = lfts[dep][R];
ucnt = lftnum[dep][R]-ncnt;
}else{
ncnt = lftnum[dep][L-1];
ucnt = lftnum[dep][R]-ncnt;
tsum = lfts[dep][R]-lfts[dep][L-1];
}
if(ucnt >= k){
L = ll + ncnt;
R = ll + ncnt + ucnt-1;
return query(L,R,ll,mid,dep+1,k);
}else{
int aa = L-ll-ncnt;
int bb = R-L-ucnt+1;
L = mid+aa+1;
R = mid+aa+bb;
ls += ucnt;
lsum += tsum;
return query(L,R,mid+1,rr,dep+1,k-ucnt);
}
}
int main(){
int ncase,T=1;
cin >>ncase;
while(ncase--){
scanf("%d",&n);
presum[0] = 0;
for(int i = 1; i <= n; i++){
scanf("%d",&num[i]);
seg[0][i] = num[i];
presum[i] = presum[i-1]+num[i];
}
sort(num+1,num+n+1);
build(1,n,0);
scanf("%d",&m);
printf("Case #%d:
",T++);
while(m--){
int a,b,k;
scanf("%d%d",&a,&b);
++a;++b;
k = (b-a)/2+1;
lsum = 0;
ls = 0;
int rs = 0;
int t = query(a,b,1,n,0,k);
LL rsum = presum[b]-presum[a-1]-t-lsum;
rs = b-a-ls;
LL ans = rsum-lsum+t*(ls-rs);
printf("%I64d
",ans);
}
printf("
");
}
return 0;
}