题目描述
Linux用户和OSX用户一定对软件包管理器不会陌生。通过软件包管理器,你可以通过一行命令安装某一个软件包,然后软件包管理器会帮助你从软件源下载软件包,同时自动解决所有的依赖(即下载安装这个软件包的安装所依赖的其它软件包),完成所有的配置。Debian/Ubuntu使用的apt-get,Fedora/CentOS使用的yum,以及OSX下可用的homebrew都是优秀的软件包管理器。
你决定设计你自己的软件包管理器。不可避免地,你要解决软件包之间的依赖问题。如果软件包A依赖软件包B,那么安装软件包A以前,必须先安装软件包B。同时,如果想要卸载软件包B,则必须卸载软件包A。现在你已经获得了所有的软件包之间的依赖关系。而且,由于你之前的工作,除0号软件包以外,在你的管理器当中的软件包都会依赖一个且仅一个软件包,而0号软件包不依赖任何一个软件包。依赖关系不存在环(若有m(m≥2)个软件包A1,A2,A3,⋯,Am,其中A1依赖A2,A2依赖A3,A3依赖A4,……,A[m-1]依赖Am,而Am依赖A1,则称这m个软件包的依赖关系构成环),当然也不会有一个软件包依赖自己。
现在你要为你的软件包管理器写一个依赖解决程序。根据反馈,用户希望在安装和卸载某个软件包时,快速地知道这个操作实际上会改变多少个软件包的安装状态(即安装操作会安装多少个未安装的软件包,或卸载操作会卸载多少个已安装的软件包),你的任务就是实现这个部分。注意,安装一个已安装的软件包,或卸载一个未安装的软件包,都不会改变任何软件包的安装状态,即在此情况下,改变安装状态的软件包数为0。
输入输出格式
输入格式:
从文件manager.in中读入数据。
输入文件的第1行包含1个整数n,表示软件包的总数。软件包从0开始编号。
随后一行包含n−1个整数,相邻整数之间用单个空格隔开,分别表示1,2,3,⋯,n−2,n−1号软件包依赖的软件包的编号。
接下来一行包含1个整数q,表示询问的总数。之后q行,每行1个询问。询问分为两种:
install x:表示安装软件包x
uninstall x:表示卸载软件包x
你需要维护每个软件包的安装状态,一开始所有的软件包都处于未安装状态。
对于每个操作,你需要输出这步操作会改变多少个软件包的安装状态,随后应用这个操作(即改变你维护的安装状态)。
输出格式:
输出到文件manager.out中。
输出文件包括q行。
输出文件的第i行输出1个整数,为第i步操作中改变安装状态的软件包数。
输入输出样例
7 0 0 0 1 1 5 5 install 5 install 6 uninstall 1 install 4 uninstall 0
3 1 3 2 3
10 0 1 2 1 3 0 0 3 2 10 install 0 install 3 uninstall 2 install 7 install 5 install 9 uninstall 9 install 4 install 1 install 9
1 3 2 1 3 1 1 1 0 1
说明
【样例说明 1】
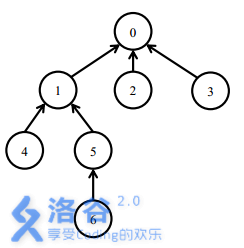
一开始所有的软件包都处于未安装状态。
安装5号软件包,需要安装0,1,5三个软件包。
之后安装6号软件包,只需要安装6号软件包。此时安装了0,1,5,6四个软件包。
卸载1号软件包需要卸载1,5,6三个软件包。此时只有0号软件包还处于安装状态。
之后安装4号软件包,需要安装1,4两个软件包。此时0,1,4处在安装状态。最后,卸载0号软件包会卸载所有的软件包。`
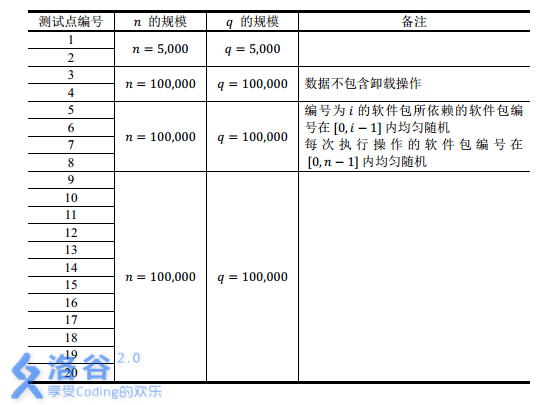
【数据范围】
【时限1s,内存512M】
//卸载操作:把以当前点为跟的子树删除,先query一下得出ans再midify //安装操作:把当前点到根所处的链的top上的点置为1,要先query一遍求出改变的个数。 #include<iostream> #include<cstdio> #include<cstring> #include<algorithm> using namespace std; const int N=1e5+5; int n,m; int head[N],num_edge; struct Edge //这道题就应该是单向边不是双向边了 { int v,nxt; }edge[N]; struct Node { int fa,son; int size; int dep; int s,t; int top; }node[N]; struct TREE { TREE *lson,*rson; int l,r,mid; int sum; int flag; }tree[N<<2]; typedef TREE* Tree; Tree now_node=tree,Root; inline int read() { char c=getchar();int num=0,f=1; for(;!isdigit(c);c=getchar()) f=c=='-'?-1:f; for(;isdigit(c);c=getchar()) num=num*10+c-'0'; return num*f; } inline void add_edge(int u,int v) { edge[++num_edge].v=v; edge[num_edge].nxt=head[u]; head[u]=num_edge; } void dfs1(int u) { node[u].size=1; for(int i=head[u],v;i;i=edge[i].nxt) { v=edge[i].v; node[v].fa=u; node[v].dep=node[u].dep+1; dfs1(v); node[u].size+=node[v].size; if(!node[u].son||node[v].size>node[node[u].son].size) node[u].son=v; } } int bound; void dfs2(int u,int top) { node[u].top=top; node[u].s=++bound; if(node[u].son) { dfs2(node[u].son,top); for(int i=head[u],v;i;i=edge[i].nxt) { v=edge[i].v; if(v==node[u].son) continue; dfs2(v,v); } } node[u].t=bound; } void build(Tree &root,int l,int r) { root=++now_node; root->l=l,root->r=r,root->mid=l+r>>1; if(l==r) return; build(root->lson,l,root->mid); build(root->rson,root->mid+1,r); } inline void pushdown(Tree root) { if(root->flag!=-1) { root->lson->flag=root->flag; root->rson->flag=root->flag; root->lson->sum=(root->lson->r-root->lson->l+1)*root->flag; root->rson->sum=(root->rson->r-root->rson->l+1)*root->flag; root->flag=-1; } } void update(const Tree &root,int l,int r,int flag) { if(l<=root->l&&root->r<=r) { root->flag=flag; root->sum=(r-l+1)*flag; return; } pushdown(root); if(r<=root->mid) update(root->lson,l,r,flag); else if(l>root->mid) update(root->rson,l,r,flag); else { update(root->lson,l,root->mid,flag); update(root->rson,root->mid+1,r,flag); } root->sum=root->lson->sum+root->rson->sum; } int query1(const Tree &root,int pos) { if(root->l==root->r) return root->sum; pushdown(root); if(pos<=root->mid) return query1(root->lson,pos); else return query1(root->rson,pos); } int query2(const Tree &root,int l,int r) { if(l<=root->l&&root->r<=r) return r-l+1-root->sum; pushdown(root); if(r<=root->mid) return query2(root->lson,l,r); else if(l>root->mid) return query2(root->rson,l,r); else return query2(root->lson,l,root->mid)+query2(root->rson,root->mid+1,r); } int query3(const Tree &root,int l,int r) { if(l<=root->l&&root->r<=r) return root->sum; pushdown(root); if(r<=root->mid) return query3(root->lson,l,r); else if(l>root->mid) return query3(root->rson,l,r); else return query3(root->lson,l,root->mid)+query3(root->rson,root->mid+1,r); } inline void Modify(int x) { int fx=node[x].top; while(fx) { update(Root,node[fx].s,node[x].s,1); x=node[fx].fa; fx=node[x].top; } update(Root,1,node[x].s,1); } inline int Query(int x) { int fx=node[x].top; int ans=0; while(fx) { ans+=query2(Root,node[fx].s,node[x].s); x=node[fx].fa; fx=node[x].top; } return ans+query2(Root,1,node[x].s); } char s[15]; int main() { n=read(); for(int i=1,u;i<n;++i) { u=read(); add_edge(u,i); } dfs1(0); dfs2(0,0); build(Root,1,n); m=read(); int x; while(m--) { scanf("%s",s); if(s[0]=='i') { x=read(); if(query1(Root,node[x].s)) puts("0"); else { printf("%d ",Query(x)); Modify(x); } } else { x=read(); if(query1(Root,node[x].s)==0) puts("0"); else { printf("%d ",query3(Root,node[x].s,node[x].t)); update(Root,node[x].s,node[x].t,0); } } } return 0; }