二叉树图解以及二叉树的递归原理

　概述　

　　递归(recursion) 又称递回，在数学与计算机科学中，是指在函数的定义中使用函数自身的方法。 
　　 一般来说，递归需要有边界条件、递归前进段和递归返回段。当边界条件不满足时，递归前进；当边界条件满足时，递归返回。 

构成递归需具备的条件：

1. 子问题须与原始问题为同样的事，且更为简单； 

2. 不能无限制地调用本身，须有个出口，化简为非递归状况处理。 
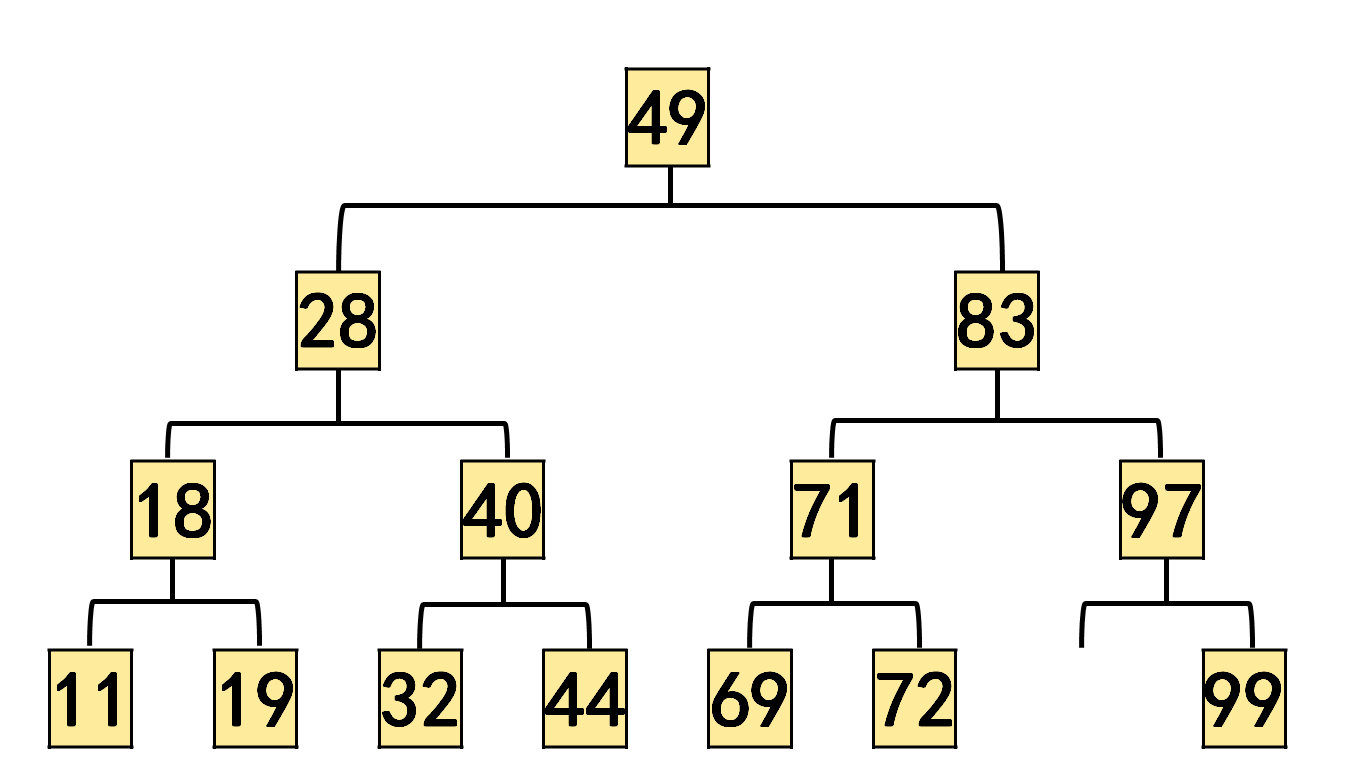
　　代码取自美国课本 "Java How to Program "(Deitel & Detel)的练习： 20.25。 以中序遍历递归方法为例，这里显示的图解，仅诠释开始一小部分递归前进段与递归返回段的交叉过程。通过这一小段的繁琐解释，希望读者可见到二叉树递归遍历的端倪。  

private void inorderHelper( TreeNode node ){      
     if ( node == null ) //若节点为空          
        return; //无任何操作 
    inorderHelper( node.leftNode ); //有序遍历下一级左子树
    System.out.print( node.data + " " ); //输出节点数据
    inorderHelper( node.rightNode );//有序遍历下一级右子树    
}     

 
 

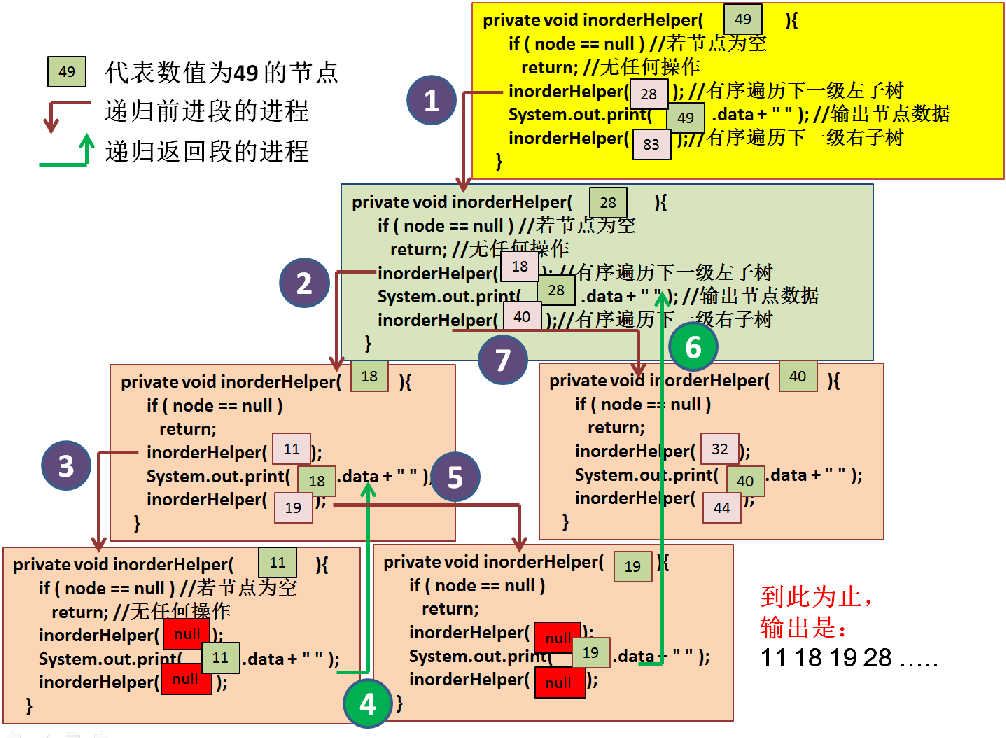
插图说明： 

 前进段的进程 1 ：鉴于以树根 节点 "49" 为参数，调用 inorderHelper(...)，开始调用以下一级树根 节点 "28" 为参数 的inorderHelper(...) 方法。 
 前进段的进程 2 ：鉴于以树根 节点 "28" 为参数，调用 inorderHelper(...)，开始调用以下一级树根 节点 "18" 为参数 的 inorderHelper(...) 方法。 
 前进段的进程 3 ：鉴于以树根 节点 "18" 为参数，调用 inorderHelper(...)，开始调用以下一级树根 节点 "11" 为参数 的 inorderHelper(...) 方法。 
 节点 "11" 为叶节点，递归前进到终点。开始启动返回操作， 输出其数值 11。 
 至此，参数为 节点 "11" 的 方法 inorderHelper(...) 执行完毕。返回进程 4 启动下一个 输出：18。 
 输出 18 的代码行执行完毕，进入前进段进程 5， 执行接下来一行的代码：调用参数为 节点 "19" 的节点的方法 inorderHelper(...) 
 节点 "19" 为叶节点，递归前进到终点。开始启动返回操作， 输出其数值 19。 
 至此，参数为 节点 "19" 的 方法 inorderHelper(...) 执行完毕。返回进程 6 启动下一个 输出：28。

实现方法：

package test;

import java.util.LinkedList;  
import java.util.List;   
//把一个数组的值存入二叉树中，然后进行3种方式的遍历 
public class BinaryTree {   
    private int[] array = { 1, 2, 3, 4, 5, 6, 7, 8, 9 };  
    private static List<Node> nodeList = null;  
    //内部类结点
    private static class Node {  
        Node leftChild;  
        Node rightChild;  
        int data;  
        //构造方法初始化
        Node(int newData) {  
            leftChild = null;  
            rightChild = null;  
            data = newData;  
        }  
    }  
    public void createBinTree() {  
        nodeList = new LinkedList<Node>();  
        // 将一个数组的值依次转换为Node节点  
        for (int nodeIndex = 0; nodeIndex < array.length; nodeIndex++) {  
            nodeList.add(new Node(array[nodeIndex]));  
        }  
        // 对前lastParentIndex-1个父节点按照父节点与孩子节点的数学关系建立二叉树  
        for (int parentIndex = 0; parentIndex < array.length / 2 - 1; parentIndex++) {  
            // 左孩子  
            nodeList.get(parentIndex).leftChild = nodeList.get(parentIndex * 2 + 1);  
            // 右孩子  
            nodeList.get(parentIndex).rightChild = nodeList.get(parentIndex * 2 + 2);  
        }  
        // 最后一个父节点:因为最后一个父节点可能没有右孩子，所以单独拿出来处理  
        int lastParentIndex = array.length / 2 - 1;  
        // 左孩子  
        nodeList.get(lastParentIndex).leftChild = nodeList.get(lastParentIndex * 2 + 1);  
        // 右孩子,如果数组的长度为奇数才建立右孩子  
        if (array.length % 2 == 1) {  
            nodeList.get(lastParentIndex).rightChild = nodeList.get(lastParentIndex * 2 + 2);  
        }  
    }  
    /** 
     * 先序遍历 
     *  
     * 这三种不同的遍历结构都是一样的，只是先后顺序不一样而已 
     *  
     * @param node 
     *            遍历的节点 
     */  
    public static void preOrderTraverse(Node node) {  
        if (node == null)  
            return;  
        System.out.print(node.data + " ");  
        preOrderTraverse(node.leftChild);  
        preOrderTraverse(node.rightChild);  
    }  
    /** 
     * 中序遍历 
     *  
     * 这三种不同的遍历结构都是一样的，只是先后顺序不一样而已 
     *  
     * @param node 
     *            遍历的节点 
     */  
    public static void inOrderTraverse(Node node) {  
        if (node == null)  
            return;  
        inOrderTraverse(node.leftChild);  
        System.out.print(node.data + " ");  
        inOrderTraverse(node.rightChild);  
    }  
    /** 
     * 后序遍历 
     *  
     * 这三种不同的遍历结构都是一样的，只是先后顺序不一样而已 
     *  
     * @param node 
     *            遍历的节点 
     */  
    public static void postOrderTraverse(Node node) {  
        if (node == null)  
            return;  
        postOrderTraverse(node.leftChild);  
        postOrderTraverse(node.rightChild);  
        System.out.print(node.data + " ");  
    }  
    public static void main(String[] args) {  
        BinaryTree binTree = new BinaryTree();  
        binTree.createBinTree();  
        // nodeList中第0个索引处的值即为根节点  
        Node root = nodeList.get(0);  
        System.out.println("先序遍历：");  
        preOrderTraverse(root);  
        System.out.println();  
        System.out.println("中序遍历：");  
        inOrderTraverse(root);  
        System.out.println();  
        System.out.println("后序遍历：");  
        postOrderTraverse(root);  
    }  
}  

输出结果：

先序遍历：

2 4 8 9 5 3 6 7
中序遍历：
4 9 2 5 1 6 3 7
后序遍历：
9 4 5 2 6 7 3 1