Calculating the derivative of a polynomial is an easy task. But what about calculating the derivative of , where
is vector? Here we denote
. To calculate the derivative of
, you should know 5 rules:
, where
is a vector here.
, where
and
means to take
as constants for
.
, where
is a constant.
, where
and
is a constant.
Now your task is to calculate the first-derivative of for some given
.
Input
The first line of the input contains an integer T (T <= 10), indicating the number of cases.
Each test case contains two blocks.The first line of the first block contains two integers, n and m (0 < n, m <= 100), indicating the number of terms of the polynomial and the dimension of
The first line of the second block contains one integer Q (0 < Q <= 100), indicating the number of queries for the given f(x). Each of the following Q lines contains m integers each, indicating the entry values of
Note: All the values in the input are nonnegative integers not exceeding 100.
OutputFor each query of each test case, output the resulting first-derivative vector in one line, with entries separated by one space and no extra space at the end of the line. For the result may be very big, you are only asked to output each element mod 1000000007.
Add a blank line between two consecutive test cases. There must be no extra blank line at the end of output.
Sample Input2 3 2 1 2 0 3 0 2 7 0 0 2 1 4 2 3 2 2 1 1 1 9 1 0 2 1 4 2 3Sample Output
2 24 4 18 13 1 12 2Hint
The first-derivative of the first case is .
The first-derivative of the second case is .
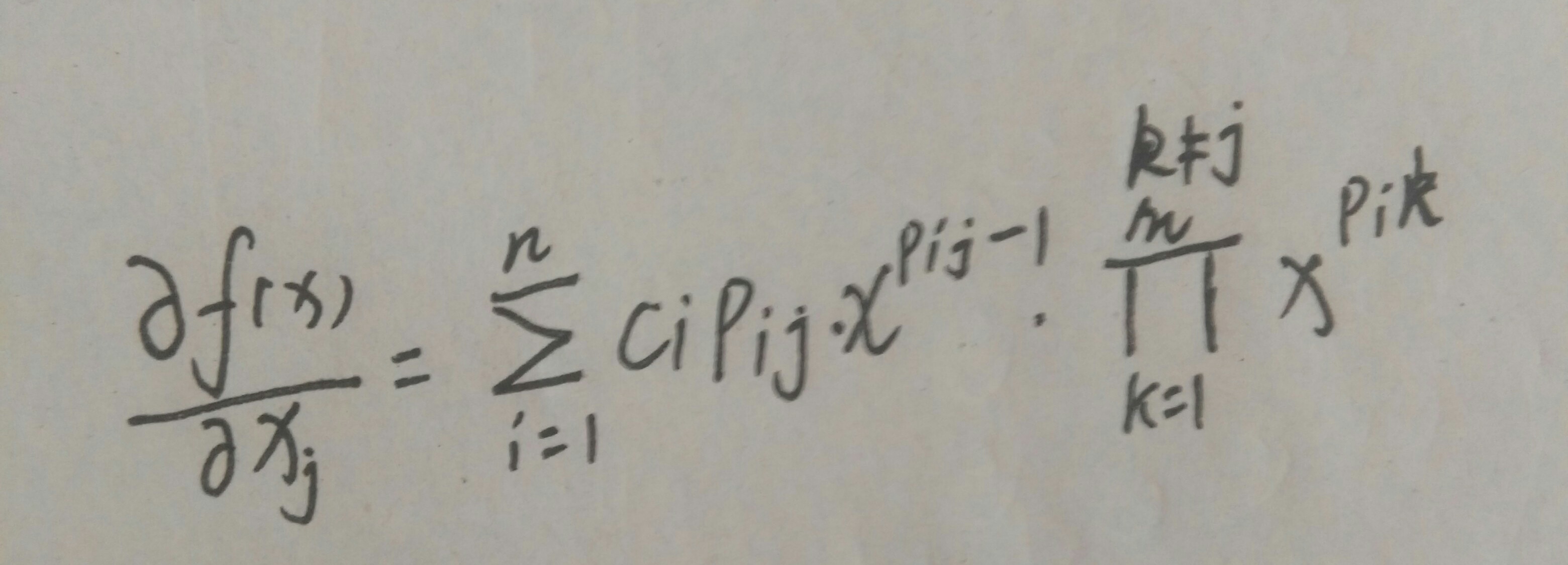
#include <cstdio> #include <iostream> #include <cstring> using namespace std; const int N=103; const int mod=1000000007; typedef long long ll; ll Pow[N][N]; void POW_init() //幂打表 { for(int i=0;i<N;i++){ Pow[0][i] = 0; Pow[i][0] = 1; } for(int i=1;i<N;i++){ long long t=1; for(int j=1;j<N;j++){ t*=i; if(t>=1000000007) t%=1000000007; Pow[i][j]=t; } } } int main() { POW_init(); int t,n,m,q; ll p[N][N],c[N],x[N]; scanf("%d",&t); while(t--) { scanf("%d%d",&n,&m); for(int i=1;i<=n;i++) { scanf("%lld",&c[i]); for(int j=1;j<=m;j++) scanf("%lld",&p[i][j]); } scanf("%d",&q); while(q--) { for(int i=1;i<=m;i++) scanf("%lld",&x[i]); for(int i=1;i<=m;i++) { ll ans=0; for(int j=1;j<=n;j++) { ll ant=1; if(!p[j][i]) continue; ant=(ant*c[j])%mod; ant=(ant*p[j][i])%mod; for(int k=1;k<=m;k++) { if(k==i) ant=(ant*Pow[x[i]][p[j][i]-1])%mod; else ant=(ant*Pow[x[k]][p[j][k]])%mod; } ans=(ans+ant)%mod; } printf("%lld%c",ans,i==m?' ':' '); } } if(t) printf(" "); } return 0; }