二叉树最大宽度
给定一个二叉树,编写一个函数来获取这个树的最大宽度。树的宽度是所有层中的最大宽度。这个二叉树与满二叉树(full binary tree)结构相同,但一些节点为空。
每一层的宽度被定义为两个端点(该层最左和最右的非空节点,两端点间的null节点也计入长度)之间的长度。
示例 1:
输入:

输出: 4
解释: 最大值出现在树的第 3 层,宽度为 4 (5,3,null,9)。
示例 2:
输入:
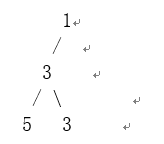
输出: 2
解释: 最大值出现在树的第 3 层,宽度为 2 (5,3)。
示例 3:
输入:
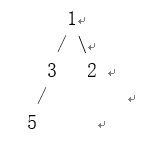
输出: 2
解释: 最大值出现在树的第 2 层,宽度为 2 (3,2)。
示例 4:
输入:
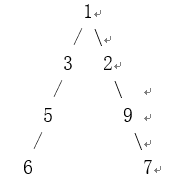
输出: 8
解释: 最大值出现在树的第 4 层,宽度为 8 (6,null,null,null,null,null,null,7)。
注意: 答案在32位有符号整数的表示范围内。
思路
pproach Framework
Explanation
As we need to reach every node in the given tree, we will have to traverse the tree, either with a depth-first search, or with a breadth-first search.
The main idea in this question is to give each node a position value. If we go down the left neighbor, then position -> position * 2; and if we go down the right neighbor, then position -> position * 2 + 1. This makes it so that when we look at the position values L and R of two nodes with the same depth, the width will be R - L + 1.
Intuition and Algorithm
Traverse each node in breadth-first order, keeping track of that node's position. For each depth, the first node reached is the left-most, while the last node reached is the right-most.
1 class Solution { 2 public int widthOfBinaryTree(TreeNode root) { 3 Queue<AnnotatedNode> queue = new LinkedList(); 4 queue.add(new AnnotatedNode(root, 0, 0)); 5 int curDepth = 0, left = 0, ans = 0; 6 while (!queue.isEmpty()) { 7 AnnotatedNode a = queue.poll(); 8 if (a.node != null) { 9 queue.add(new AnnotatedNode(a.node.left, a.depth + 1, a.pos * 2)); 10 queue.add(new AnnotatedNode(a.node.right, a.depth + 1, a.pos * 2 + 1)); 11 if (curDepth != a.depth) { 12 curDepth = a.depth; 13 left = a.pos; 14 } 15 ans = Math.max(ans, a.pos - left + 1); 16 } 17 } 18 return ans; 19 } 20 } 21 22 class AnnotatedNode { 23 TreeNode node; 24 int depth, pos; 25 AnnotatedNode(TreeNode n, int d, int p) { 26 node = n; 27 depth = d; 28 pos = p; 29 } 30 }
