HDU Computer (树形DP)

Computer

Time Limit : 1000/1000ms (Java/Other)   Memory Limit : 32768/32768K (Java/Other)

Total Submission(s) : 1   Accepted Submission(s) : 1

Font: Times New Roman | Verdana | Georgia

Font Size: ← →

Problem Description

A school bought the first computer some time ago(so this computer's id is 1). During the recent years the school bought N-1 new computers. Each new computer was connected to one of settled earlier. Managers of school are anxious about slow functioning of the net and want to know the maximum distance Si for which i-th computer needs to send signal (i.e. length of cable to the most distant computer). You need to provide this information. 


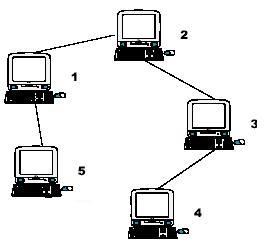
Hint: the example input is corresponding to this graph. And from the graph, you can see that the computer 4 is farthest one from 1, so S1 = 3. Computer 4 and 5 are the farthest ones from 2, so S2 = 2. Computer 5 is the farthest one from 3, so S3 = 3. we also get S4 = 4, S5 = 4.

Input

Input file contains multiple test cases.In each case there is natural number N (N<=10000) in the first line, followed by (N-1) lines with descriptions of computers. i-th line contains two natural numbers - number of computer, to which i-th computer is connected and length of cable used for connection. Total length of cable does not exceed 10^9. Numbers in lines of input are separated by a space.

Output

For each case output N lines. i-th line must contain number Si for i-th computer (1<=i<=N).

Sample Input

5
1 1
2 1
3 1
1 1

Sample Output

3
2
3
4
4

Author

scnu

首先按所给信息建图，建立的是有向图。然后将1视为根节点第一遍dfs从叶往根递推，求出每个点所能到达的最远距离和次远距离，只有当这一个点有多条分支时才存在次远距离（注意这里是有方向）；

该点所能到达的最远距离可能是第一遍dfs搜到最远距离，也有可能由父亲节点+父亲节点与该节点的距离所得。所以第二遍dfs从根节点1出发往树叶边遍历。

 

downf[i]表示以节点 i 向其 子树搜索 所能到达的最远距离

downs[i]表示次远距离

vis[i]记录 到达最远距离的那条边（最远 和次远 不会重边）

dp[i]表示 节点i 经其父亲节点延伸(延伸到父亲的最长或着次长)，所能到达的最远距离

求 i的最远时， i可能在父亲的最长路径上，也可能不再，所以要记录

 

最远 可能是向上最远 也可能向下 所以最后要 max(downf[k],dp[k]);

 

 

#include<iostream>
#include<cstdio>
#include<cstring>
#include<vector>

using namespace std;

const int N=10010;

struct node{
    int to,cap;
}a;

vector<node> vt[N];
int n,dp[N],downf[N],downs[N],vis[N];

void DFS1(int u){
    int flag1=-1,flag2=-1;
    if(downf[u])
        return ;
    int len=vt[u].size();
    if(len==0)
        return ;
    int MAX=-1;
    for(int i=0;i<len;i++){     //求最长距离,  由下往上递推 
        int v=vt[u][i].to;
        DFS1(v);                //先dfs
        if(MAX<downf[v]+vt[u][i].cap){  //后处理
            MAX=downf[v]+vt[u][i].cap;
            flag1=i;
        }
    }
    vis[u]=flag1;
    downf[u]=MAX;
    MAX=-1;
    for(int i=0;i<len;i++){     //求次长距离
        int v=vt[u][i].to;
        if(i!=flag1 && MAX<downf[v]+vt[u][i].cap){  //i!=flag1 保证最远距离和次远距离不会重边
            MAX=downf[v]+vt[u][i].cap;
            flag2=i;
        }
    }
    if(flag2!=-1)
        downs[u]=MAX;
}

void DFS2(int u){       //由上往下推 
    int len=vt[u].size();
    if(len==0)
        return ;
    for(int i=0;i<len;i++){
        int v=vt[u][i].to;
        if(i==vis[u])   //先处理
            dp[v]=max(downs[u],dp[u])+vt[u][i].cap;     //在父亲的最长路径上
        else
            dp[v]=max(downf[u],dp[u])+vt[u][i].cap;     //不在父亲的最长路径上
        DFS2(v);        //后dfs
    }
}

int main(){

    //freopen("input.txt","r",stdin);

    while(~scanf("%d",&n)){
        for(int i=0;i<=n;i++)
            vt[i].clear();
        int u;
        for(int v=2;v<=n;v++){
            scanf("%d%d",&u,&a.cap);
            a.to=v;
            vt[u].push_back(a);
        }
        memset(dp,0,sizeof(dp));
        memset(downf,0,sizeof(downf));
        memset(downs,0,sizeof(downs));
        DFS1(1);
        DFS2(1);
        for(int i=1;i<=n;i++)
            printf("%d\n",max(downf[i],dp[i]));
    }
    return 0;
}

 另一种建图：

#include<iostream>
#include<cstdio>
#include<cstring>

using namespace std;

const int VM=10010;

struct Edge{
    int to,nxt;
    int cap;
}edge[VM<<1];

int n,cnt,head[VM];
int dp[VM],downf[VM],downs[VM],vis[VM];

void addedge(int cu,int cv,int cw){
    edge[cnt].to=cv;    edge[cnt].cap=cw;   edge[cnt].nxt=head[cu];
    head[cu]=cnt++;
}

void DFS1(int u){
    int flag1=-1,flag2=-1;
    if(downf[u])
        return ;
    if(head[u]==-1)
        return ;
    int MAX=-1;
    for(int i=head[u];i!=-1;i=edge[i].nxt){
        int v=edge[i].to;
        DFS1(v);
        if(MAX<downf[v]+edge[i].cap){
            MAX=downf[v]+edge[i].cap;
            flag1=i;
        }
    }
    vis[u]=flag1;
    downf[u]=MAX;
    MAX=-1;
    for(int i=head[u];i!=-1;i=edge[i].nxt){
        int v=edge[i].to;
        if(i!=flag1 && MAX<downf[v]+edge[i].cap){
            MAX=downf[v]+edge[i].cap;
            flag2=i;
        }
    }
    if(flag2!=-1)
        downs[u]=MAX;
}

void DFS2(int u){
    if(head[u]==-1)
        return ;
    for(int i=head[u];i!=-1;i=edge[i].nxt){
        int v=edge[i].to;
        if(i==vis[u])
            dp[v]=max(downs[u],dp[u])+edge[i].cap;
        else
            dp[v]=max(downf[u],dp[u])+edge[i].cap;
        DFS2(v);
    }
}

int main(){

    //freopen("input.txt","r",stdin);

    while(~scanf("%d",&n)){
        cnt=0;
        memset(head,-1,sizeof(head));
        int u,w;
        for(int v=2;v<=n;v++){
            scanf("%d%d",&u,&w);
            addedge(u,v,w);
        }
        memset(dp,0,sizeof(dp));
        memset(downf,0,sizeof(downf));
        memset(downs,0,sizeof(downs));
        DFS1(1);
        DFS2(1);
        for(int i=1;i<=n;i++)
            printf("%d\n",max(downf[i],dp[i]));
    }
    return 0;
}