第四周作业
机卓1301储景瑞
一、设计任务:
结合本周学习的交流电机原理及启动、调速、制动特性,用Modelica设计和仿真一个用三相交流异步电机带动起重机起升机构运行。具体要求如下:
1)实现如下机械运动周期:
控制电机带重物上升,从静止加速到800r/min
保持800r/min匀速运动0.5s,
减速到静止,保持静止状态0.5s,
带重物下降,从静止达到600r/min
保持600r/min匀速运动0.6s,
减速到静止。
(为了便于仿真,匀速和静止持续时间较短)
2) 升降机构和重物折算到到电机转子轴上的等效负载惯量为1Kg.m^2,折算到到电机转子轴上的等效负载转矩是15N.m。
3)使用统一的电机模型,如果控制策略中用到转子串电阻,允许将该电机的转子改为绕线式转子(参数不变)。
4)参照教材中给出的交流电机启动、调速和制动方法,设计控制策略,用Modelica实现控制策略并与电机模型实现联合仿真。
5)可以采用定子串电阻、转子串电阻、定子调压、定子调频等手段,但必须具备工程上的可实施性。
6)评价指标:快速启动、制动,冲击转矩和冲击电流小,能耗小,兼顾实施的经济性。
7)方案最佳同学获本周"控制之星"称号。
二、理论推导
三、仿真结果


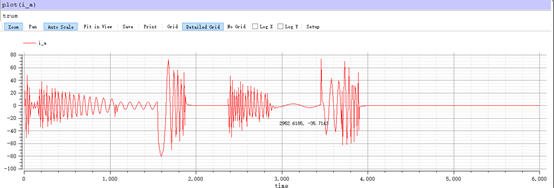
由仿真结果可以看出,该方案满足题目要求,时间也很短
四、学习心得
三相交流电机的计算还是比较麻烦的,不过套用书上的公式也不是很麻烦,但是要真正从其本质上理解它还是有点难度的,至少到现在我只有一个定性的理解,但是相信认真推导,与直流电机做类比,最后是可以理解的。同时还是很佩服特斯拉的,我想他也是掌握了最小作用原理的,我的理解是最小作用的都是对称的,还有很多极值点也都是在对称位置,但是一时也找不到具体的例子。
五、源代码
model SACIM "A Simple AC Induction Motor Model"
type Voltage=Real(unit="V");
type Current=Real(unit="A");
type Resistance=Real(unit="Ohm");
type Inductance=Real(unit="H");
type Speed=Real(unit="r/min");
type Torque=Real(unit="N.m");
type Inertia=Real(unit="kg.m^2");
type Frequency=Real(unit="Hz");
type Flux=Real(unit="Wb");
type Angle=Real(unit="rad");
type AngularVelocity=Real(unit="rad/s");
constant Real Pi = 3.1415926;
Current i_A"A Phase Current of Stator";
Current i_B"B Phase Current of Stator";
Current i_C"C Phase Current of Stator";
Voltage u_A"A Phase Voltage of Stator";
Voltage u_B"B Phase Voltage of Stator";
Voltage u_C"C Phase Voltage of Stator";
Current i_a"A Phase Current of Rotor";
Current i_b"B Phase Current of Rotor";
Current i_c"C Phase Current of Rotor";
Frequency f_s"Frequency of Stator";
Torque Tm"Torque of the Motor";
Speed n"Speed of the Motor";
Flux Psi_A"A Phase Flux-Linkage of Stator";
Flux Psi_B"B Phase Flux-Linkage of Stator";
Flux Psi_C"C Phase Flux-Linkage of Stator";
Flux Psi_a"a Phase Flux-Linkage of Rotor";
Flux Psi_b"b Phase Flux-Linkage of Rotor";
Flux Psi_c"c Phase Flux-Linkage of Rotor";
Angle phi"Electrical Angle of Rotor";
Angle phi_m"Mechnical Angle of Rotor";
AngularVelocity w"Angular Velocity of Rotor";
Torque Tl"Load Torque";
parameter Resistance Rs = 0.531"Stator Resistance";
parameter Resistance Rr=8 "Rotor Resistance";
parameter Inductance Ls = 0.00252"Stator Leakage Inductance";
parameter Inductance Lr = 0.00252"Rotor Leakage Inductance";
parameter Inductance Lm = 0.00847"Mutual Inductance";
parameter Frequency f_N = 50"Rated Frequency of Stator";
parameter Voltage u_N = 220"Rated Phase Voltage of Stator";
parameter Real p =2"number of pole pairs";
parameter Inertia Jm = 0.1"Motor Inertia";
parameter Inertia Jl = 1"Load Inertia";
initial equation
Psi_A = 0;
Psi_B = 0;
Psi_C = 0;
Psi_a = 0;
Psi_b = 0;
Psi_c = 0;
phi = 0;
w = 0;
equation
u_A = Rs * i_A + 1000 * der(Psi_A);
u_B = Rs * i_B + 1000 * der(Psi_B);
u_C = Rs * i_C + 1000 * der(Psi_C);
0 = Rr * i_a + 1000 * der(Psi_a);
0 = Rr* i_b + 1000 * der(Psi_b);
0 = Rr * i_c + 1000 * der(Psi_c);
Psi_A = (Lm+Ls)*i_A + (-0.5*Lm)*i_B + (-0.5*Lm)*i_C + (Lm*cos(phi))*i_a + (Lm*cos(phi+2*Pi/3))*i_b + (Lm*cos(phi-2*Pi/3))*i_c;
Psi_B = (-0.5*Lm)*i_A + (Lm+Ls)*i_B + (-0.5*Lm)*i_C + (Lm*cos(phi-2*Pi/3))*i_a + (Lm*cos(phi))*i_b + (Lm*cos(phi+2*Pi/3))*i_c;
Psi_C = (-0.5*Lm)*i_A + (-0.5*Lm)*i_B + (Lm+Ls)*i_C + (Lm*cos(phi+2*Pi/3))*i_a + (Lm*cos(phi-2*Pi/3))*i_b + (Lm*cos(phi))*i_c;
Psi_a = (Lm*cos(phi))*i_A + (Lm*cos(phi-2*Pi/3))*i_B + (Lm*cos(phi+2*Pi/3))*i_C + (Lm+Lr)*i_a + (-0.5*Lm)*i_b + (-0.5*Lm)*i_c;
Psi_b = (Lm*cos(phi+2*Pi/3))*i_A + (Lm*cos(phi))*i_B + (Lm*cos(phi-2*Pi/3))*i_C + (-0.5*Lm)*i_a + (Lm+Lr)*i_b + (-0.5*Lm)*i_c;
Psi_c = (Lm*cos(phi-2*Pi/3))*i_A + (Lm*cos(phi+2*Pi/3))*i_B + (Lm*cos(phi))*i_C + (-0.5*Lm)*i_a + (-0.5*Lm)*i_b + (Lm+Lr)*i_c;
Tm =-p*Lm*((i_A*i_a+i_B*i_b+i_C*i_c)*sin(phi)+(i_A*i_b+i_B*i_c+i_C*i_a)*sin(phi+2*Pi/3)+(i_A*i_c+i_B*i_a+i_C*i_b)*sin(phi-2*Pi/3));
w = 1000 * der(phi_m);
phi_m = phi/3;
n= w*60/(2*Pi);
Tm-Tl = (Jm+Jl) * 1000 * der(w);
if time <= 10 then
u_A = 0;
u_B = 0;
u_C = 0;
f_s = 0;
Tl = 0;
else if time<=1050 then
f_s = f_N;
u_A = u_N *1.732* 1.414 * sin(2*Pi*f_s*time/1000);
u_B = u_N *1.732* 1.414 * sin(2*Pi*f_s*time/1000-2*Pi/3);
u_C = u_N *1.732* 1.414 * sin(2*Pi*f_s*time/1000-4*Pi/3);
Tl =15;
else if time <=1550 then
f_s = f_N;
u_A = u_N * 1.414 * sin(2*Pi*f_s*time/1000);
u_B = u_N * 1.414 * sin(2*Pi*f_s*time/1000-2*Pi/3);
u_C = u_N * 1.414 * sin(2*Pi*f_s*time/1000-4*Pi/3);
Tl =15;
else if time <=1875 then
f_s = f_N;
u_A = u_N *1.732* 1.414 * sin(2*Pi*f_s*time/1000);
u_B = u_N *1.732* 1.414 * sin(2*Pi*f_s*time/1000-4*Pi/3);
u_C = u_N *1.732* 1.414 * sin(2*Pi*f_s*time/1000-2*Pi/3);
Tl =15;
else if time <=2375 then
u_A = 0;
u_B = 0;
u_C = 0;
f_s = 0;
Tl = 0;
else if time <=2825 then
f_s = f_N;
u_A = u_N *1.732* 1.414 * sin(2*Pi*f_s*time/1000);
u_B = u_N *1.732* 1.414 * sin(2*Pi*f_s*time/1000-4*Pi/3);
u_C = u_N *1.732* 1.414 * sin(2*Pi*f_s*time/1000-2*Pi/3);
Tl =15;
else if time <=3450 then
f_s = f_N*6/11;
u_A = u_N * 1.414 * sin(2*Pi*f_s*time/1000);
u_B = u_N * 1.414 * sin(2*Pi*f_s*time/1000-4*Pi/3);
u_C = u_N * 1.414 * sin(2*Pi*f_s*time/1000-2*Pi/3);
Tl =15;
else if time <=3700 then
f_s = f_N;
u_A = u_N * 1.414 * sin(2*Pi*f_s*time/1000);
u_B = u_N * 1.414 * sin(2*Pi*f_s*time/1000-2*Pi/3);
u_C = u_N * 1.414 * sin(2*Pi*f_s*time/1000-4*Pi/3);
Tl =15;
else if time <=3895 then
f_s = f_N;
u_A = u_N *1.732* 1.414 * sin(2*Pi*f_s*time/1000);
u_B = u_N * 1.732*1.414 * sin(2*Pi*f_s*time/1000-2*Pi/3);
u_C = u_N *1.732* 1.414 * sin(2*Pi*f_s*time/1000-4*Pi/3);
Tl =15;
else
u_A = 0;
u_B = 0;
u_C = 0;
f_s = 0;
Tl = 0;
end if;
end if ;
end if;
end if;
end if;
end if;
end if;
end if;
end if;
end SACIM;
simulate(SACIM,startTime=0,stopTime=6000)
plot(n)
plot(i_A)
plot(i_a)
