力扣208——实现 Trie (前缀树)

这道题主要是构造前缀树节点的数据结构，帮助解答问题。

原题

实现一个 Trie (前缀树)，包含 insert, search, 和 startsWith 这三个操作。

示例:

Trie trie = new Trie();

trie.insert("apple");
trie.search("apple");   // 返回 true
trie.search("app");     // 返回 false
trie.startsWith("app"); // 返回 true
trie.insert("app");   
trie.search("app");     // 返回 true

说明:

• 你可以假设所有的输入都是由小写字母 a-z 构成的。
• 保证所有输入均为非空字符串。

原题url：https://leetcode-cn.com/problems/implement-trie-prefix-tree/

解题

前缀树的意义

我们用前缀树这种数据结构，主要是用在在字符串数据集中搜索单词的场景，但针对这种场景，我们也可以使用平衡树和哈希表，而且哈希表可以在O(1)时间内寻找到键值。那为什么还要前缀树呢？

原因有3：

1. 前缀树可以找到具有同意前缀的全部键值。
2. 前缀树可以按词典枚举字符串的数据集。
3. 前缀树在存储多个具有相同前缀的键时可以使用较少的空间，只需要O(m)的时间复杂度，其中 m 为键长。在平衡树中查找键值却需要O(m log n)，其中 n 是插入的键的数量；而哈希表随着大小的增加，会出现大量的冲突，时间复杂度可能增加到O(n)。

构造前缀树的节点结构

既然是树，肯定也是有根节点的。至于其节点结构，需要有以下特点：

1. 最多 R 个指向子结点的链接，其中每个链接对应字母表数据集中的一个字母。本题中假定 R 为 26，小写拉丁字母的数量。
2. 布尔字段，以指定节点是对应键的结尾还是只是键前缀。

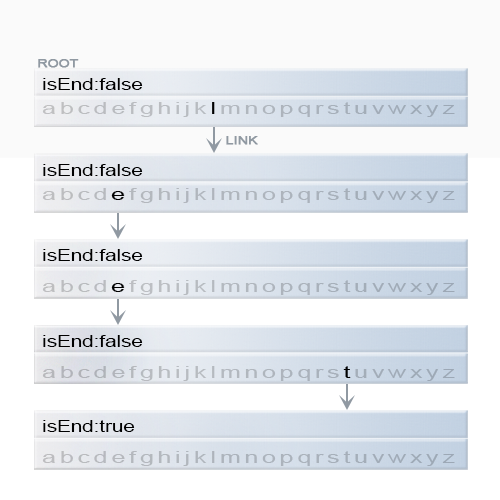
接下来让我们看看节点结构的代码：

class TrieNode {

    TrieNode[] nodes;

    boolean isEnd;

    public TrieNode() {
        // 26个小写英文字母
        nodes = new TrieNode[26];
        // 当前是否已经结束
        isEnd = false;
    }
		
		/**
		 * 当前节点是否包含字符 ch
		 */
    public boolean contains(char ch) {
        return nodes[ch - 'a'] != null;
    }
		
		/**
		 * 设置新的下一个节点
		 */
    public TrieNode setNode(char ch, TrieNode node) {
		    // 判断当前新的节点是否已经存在
        TrieNode tempNode = nodes[ch - 'a'];
				// 如果存在，就直接返回已经存在的节点
        if (tempNode != null) {
            return tempNode;
        }

        // 否则就设置为新的节点，并返回
        nodes[ch - 'a'] = node;
        return node;
    }
		
		/**
		 * 获取 ch 字符
		 */
    public TrieNode getNode(char ch) {
        return nodes[ch - 'a'];
    }
		
		/**
		 * 设置当前节点为结束
		 */
    public void setIsEnd() {
        isEnd = true;
    }

    /**
		 * 当前节点是否已经结束
		 */
    public boolean isEnd() {
        return isEnd;
    }
}

接下来就是真正的前缀树的结构：

class Trie {

    /**
		 * 根节点
		 */
    TrieNode root;

    /** Initialize your data structure here. */
    public Trie() {
        root = new TrieNode();
    }
    
    /** Inserts a word into the trie. */
    public void insert(String word) {
        TrieNode before = root;
        TrieNode node;
				// 遍历插入单词中的每一个字母
        for (int i = 0; i < word.length(); i++) {
            node = new TrieNode();
            node = before.setNode(word.charAt(i), node);
            before = node;
        }
				// 设置当前为终点
        before.setIsEnd();
    }
    
    /** Returns if the word is in the trie. */
    public boolean search(String word) {
        TrieNode before = root;
        TrieNode temp;
				// 遍历查找
        for (int i = 0; i < word.length(); i++) {
            temp = before.getNode(word.charAt(i));
            if (temp == null) {
                return false;
            }
            before = temp;
        }
				// 且最后一个节点也是终点
        return before.isEnd();
    }
    
    /** Returns if there is any word in the trie that starts with the given prefix. */
    public boolean startsWith(String prefix) {
        TrieNode before = root;
        TrieNode temp;
				// 遍历查找
        for (int i = 0; i < prefix.length(); i++) {
            temp = before.getNode(prefix.charAt(i));
            if (temp == null) {
                return false;
            }
            before = temp;
        }
        return true;
    }
}

提交OK，执行用时：43 ms，内存消耗：55.3 MB，虽然只战胜了87.40%的提交，但试了一下最快的那个代码，和我这个方法在时间上基本没什么差别，应该是当初提交的时候测试用例没有那么多吧。

总结

以上就是这道题目我的解答过程了，不知道大家是否理解了。这道题目可能需要专门去理解一下前缀树的用途，这样可以有助于构造前缀树的结构。

有兴趣的话可以访问我的博客或者关注我的公众号、头条号，说不定会有意外的惊喜。

https://death00.github.io/

公众号：健程之道