在对数据进行可视化的过程中,可能经常需要对数据进行三维绘图,在python中进行三维绘图其实是比较简单的,下面我们将给出一个二元高斯分布的三维图像案例,并且给出相关函数的参数。
通常,我们绘制三维图像经常需要如下步骤:
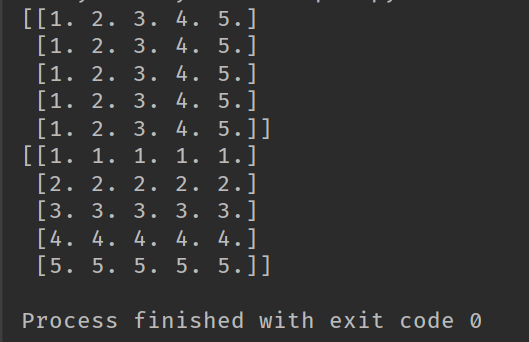
1.生成二维的网格坐标数据,我们可以使用np.meshgrid(x, y)函数进行二维网格坐标的生成,该函数通过传入的参数生成两个坐标的网格数据,并且返回的数据具有如下的格式:
import numpy as np t = np.linspace(1, 5, 5) x, y = np.meshgrid(t,t) print(x) print(y)
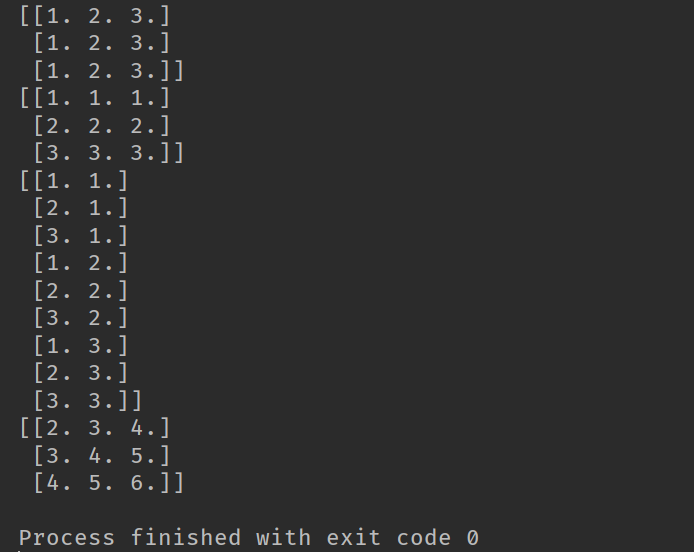
2.通过网格坐标,生成z轴上的网格坐标。得到了x,y的网格数据之后,我们需要根据x,y的数据的到z的网格数据,我们可以通过迭代将x,y进行拼接,形成列数为2的矩阵,并通过矩阵计算z的数据,计算之后,在将z的数据维度进行转换,得到z的网格数据。
xy = np.stack([x.flat, y.flat], axis=1) print(xy)
xy = np.stack([x.flat, y.flat], axis=1) print(xy) z = xy[:, 0] + xy[:, 1] z = np.array(z) z = z.reshape(x.shape) print(z)

3.获取绘制3维图像的句柄,调用相关函数进行绘制,绘制3维图面ax.plot_surface(x, y, z, cmap='rainbow', rstride=1, cstride=1),绘制3维曲线ax.scatter(),参数类似前者。
import numpy as np from mpl_toolkits.mplot3d import Axes3D import matplotlib.pyplot as plt fig = plt.figure() ax = Axes3D(fig)
ax.plot_surface(x, y, z, cmap='rainbow', rstride=1, cstride=1) ax.set_xlabel("x") ax.set_ylabel("y") ax.set_zlabel("z")
4.基本上很多的绘图思路都如上,比如绘制一些分类器的分类区域图。
import numpy as np from mpl_toolkits.mplot3d import Axes3D import matplotlib.pyplot as plt fig = plt.figure() ax = Axes3D(fig) t = np.linspace(1, 5, 30) x, y = np.meshgrid(t,t) print(x) print(y) xy = np.stack([x.flat, y.flat], axis=1) print(xy) z = xy[:, 0] * xy[:, 1] z = np.array(z) z = z.reshape(x.shape) print(z) ax.plot_surface(x, y, z, cmap='rainbow', rstride=1, cstride=1) ax.set_xlabel("x") ax.set_ylabel("y") ax.set_zlabel("z") plt.show()

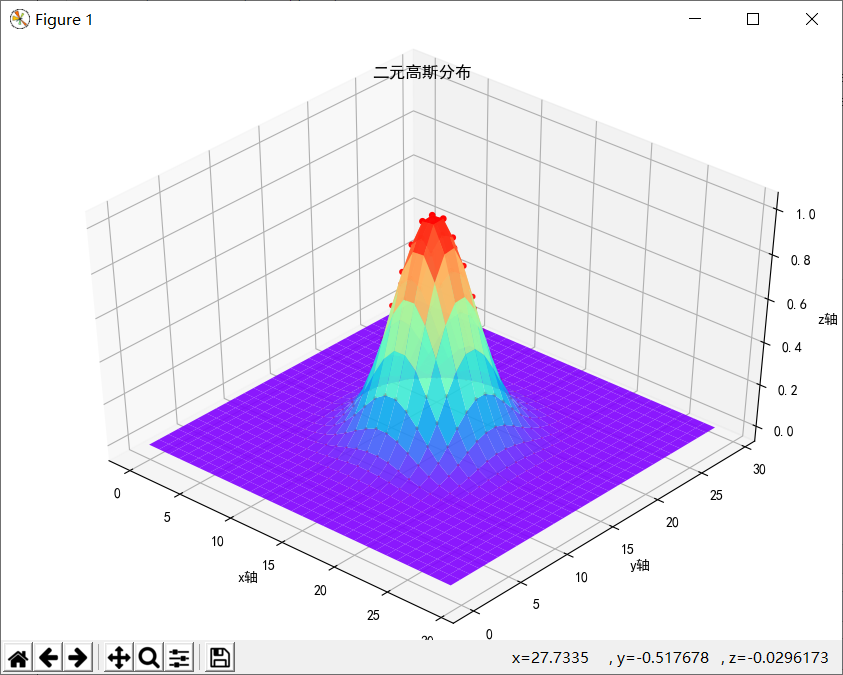
5.绘制二元高斯分布3维图
import numpy as np import matplotlib.pyplot as plt from mpl_toolkits.mplot3d import Axes3D import matplotlib as mpl if __name__ == '__main__': mpl.rcParams['font.sans-serif'] = ['SimHei'] mpl.rcParams['axes.unicode_minus'] = False d = np.random.randn(10000000, 2) N = 30 density, edges = np.histogramdd(d, bins=[30, 30]) print("样本总数: ", np.sum(density)) density = density/density.max() x = y = np.arange(N) t = np.meshgrid(x,y) fig = plt.figure() ax = Axes3D(fig) ax.scatter(t[0], t[1], density, c='r', s=15*density, marker='o', depthshade=True) ax.plot_surface(t[0], t[1], density, cmap='rainbow', rstride=1, cstride=1, alpha=0.9, lw=1) ax.set_xlabel("x轴") ax.set_ylabel("y轴") ax.set_zlabel("z轴") plt.title("二元高斯分布") plt.tight_layout(0.1) plt.show()