阿基米德螺旋线原理及代码

一个点在射线上匀速向外运动，同时射线以w的速度转动，点的轨迹就被称为阿基米德螺旋线或等速螺线。

1.公式

阿基米德螺旋线的极坐标公式可以表示为：
$r = a+b* heta$
其中a为起始点与极坐标中心的距离，主要负责旋转整个螺线（增加a顺时针旋转）；
b为控制螺线间的螺距，$b = dfrac{r}{ heta}$,b越大变化越快螺线越密；
$heta$的范围控制了螺线的大小，$heta$越大螺线的范围越大。


在直角坐标系下，利用极坐标系到直角坐标的公式，其公式可以被改写为：
$x = r*cos heta\ y = r*sin heta$
$x = (a+b* heta)*cos heta\ y = (a+b* heta)*sin heta$


此外还可以利用角速度和线速度的概念来控制螺线的形状，生成其他螺旋线：
$x = vt*cos(wt)\ y = vt*cos(wt)$
上式为关于t的参数方程，其中v为线速度、w为角速度，t为点运动的时间。可以通过上式子得到等角速度、等线速度等各类螺旋

2.程序

首先我们来画出极坐标系下的阿基米德螺线。

%matlab 程序
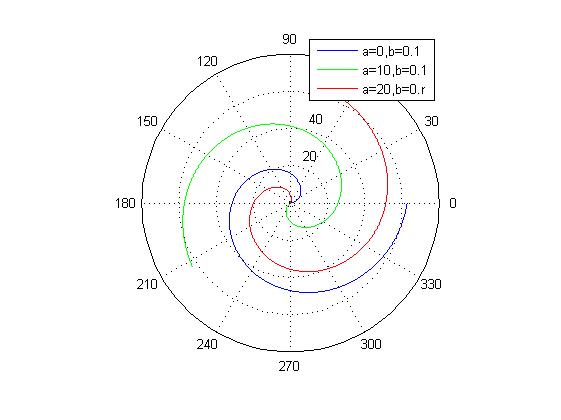
%不同a和b造成螺线的变化
theta = 0:0.01*pi:20*pi;
r1 = 0 + 0.1*theta;
r2 = 10 + 0.1*theta;
r3 = 20 + 0.1*theta;

polar(r1,theta,'b');hold on;
polar(r2,theta,'g')
polar(r3,theta,'r')

legend('a=0,b=0.1','a=10,b=0.1','a=20,b=0.r')

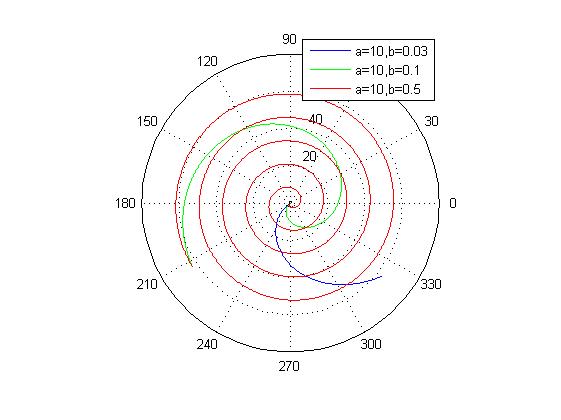
随后变化b来观察螺距的变化：

theta = 0:0.01*pi:20*pi;
r4 = 10 + 0.03*theta;
r5 = 10 + 0.1*theta;
r6 = 10 + 0.5*theta;

polar(r4,theta,'b');hold on;
polar(r5,theta,'g')
polar(r6,theta,'r')

legend('a=10,b=0.03','a=10,b=0.1','a=10,b=0.5')

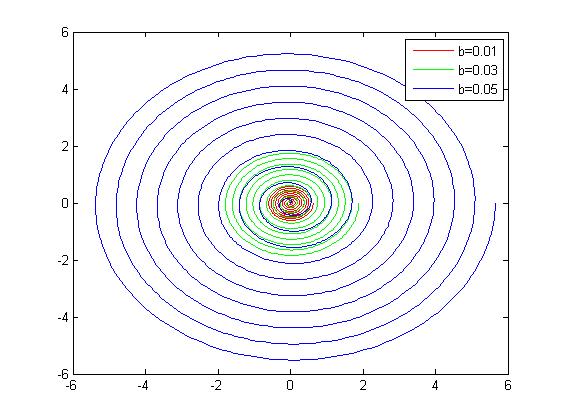
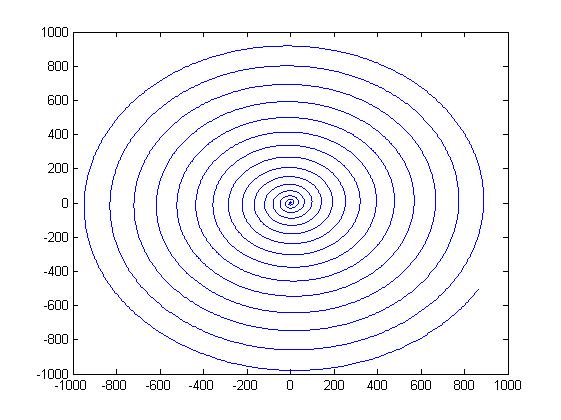
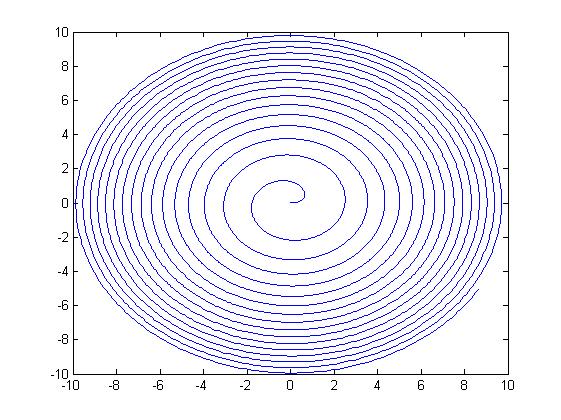
随后在直角坐标系中画出螺线：

theta = 0:0.01*pi:20*pi;
r7 = 0 + 0.01*theta;
x = r7.*cos(theta);
y = r7.*sin(theta);
%初始点是0，螺距为0.01
%在直角坐标系下b控制着螺线间距，b越大螺线间距越大
plot(x,y,'r')

hold on;
r8 = 0 + 0.03*theta;
x = r8.*cos(theta);
y = r8.*sin(theta);
plot(x,y,'g')

r9 = 0 + 0.09*theta;
x = r9.*cos(theta);
y = r9.*sin(theta);
plot(x,y,'b')

legend('b=0.01','b=0.03','b=0.05')

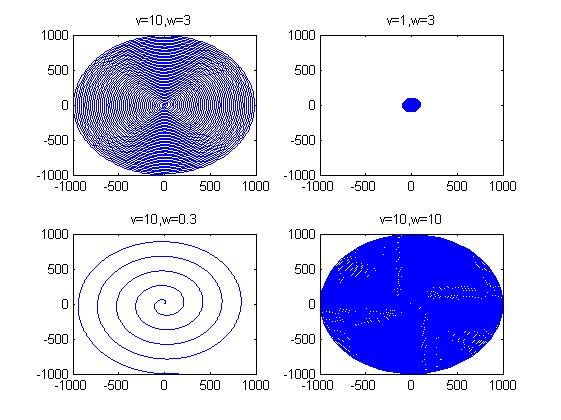
另外可以通过引入速度的概念控制螺线的形状：

t = 0:0.01:100;
v = 10;  %线速度控制了大小，越大走得越快螺线形状越大
w = 3 ; %角速度控制了疏密，越小越稀疏，单位时间内旋转少。
x = v*t.*cos(w*t);
y = v*t.*sin(w*t);
subplot(2,2,1);
plot(x,y)
title('v=10,w=3')
axis([-1000 1000 -1000 1000])


v = 1;  %线速度变小，图形变小
w = 3 ; 
x = v*t.*cos(w*t);
y = v*t.*sin(w*t);
subplot(2,2,2)
plot(x,y)
title('v=1,w=3')
axis([-1000 1000 -1000 1000])


v = 10;  
w = 0.3 ; %角速度变小，图形变疏
x = v*t.*cos(w*t);
y = v*t.*sin(w*t);
subplot(2,2,3)
plot(x,y)
title('v=10,w=0.3')
axis([-1000 1000 -1000 1000])

v = 10;  %变大
w = 10 ; %变密
x = v*t.*cos(w*t);
y = v*t.*sin(w*t);
subplot(2,2,4)
plot(x,y)
title('v=10,w=10')
axis([-1000 1000 -1000 1000])

或者将w变化或者v变化：

%向外渐开线
t = 0:0.01:100;
v = 0:0.001:10;  %线速度逐渐加快
w = 1 ; %
x = v.*t.*cos(w*t);
y = v.*t.*sin(w*t);
plot(x,y)


%渐密线
t = 0:0.01:100;
v = 0.1;  
w = 0:0.0001:1; ; %角速度逐渐加大变密.  tips：一定要注意区分坐标系，以及角速度与b的关系
x = v.*t.*cos(w.*t);
y = v.*t.*sin(w.*t);
plot(x,y)

---

ref
https://baike.baidu.com/item/阿基米德螺线/6174118?fr=aladdin
http://muchong.com/html/201106/3290808.html
https://blog.csdn.net/menghuanxiy/article/details/81348393
http://blog.sina.cn/dpool/blog/s/blog_62c180b20102wx3n.html

pic from pexels.com