HDOJ 1301 Jungle Roads （最小生成树）

Jungle Roads

Time Limit: 2000/1000 MS (Java/Others)    Memory Limit: 65536/32768 K (Java/Others)
Total Submission(s): 5681    Accepted Submission(s): 4098

Problem Description

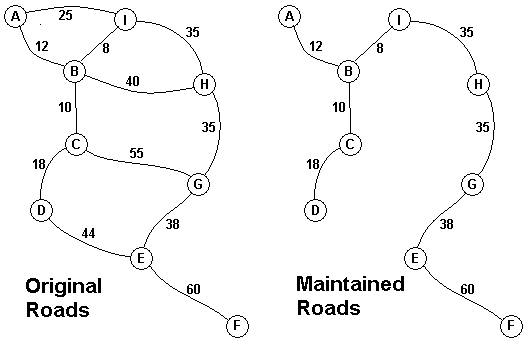
The Head Elder of the tropical island of Lagrishan has a problem. A burst of foreign aid money was spent on extra roads between villages some years ago. But the jungle overtakes roads relentlessly, so the large road network is too expensive to maintain. The Council of Elders must choose to stop maintaining some roads. The map above on the left shows all the roads in use now and the cost in aacms per month to maintain them. Of course there needs to be some way to get between all the villages on maintained roads, even if the route is not as short as before. The Chief Elder would like to tell the Council of Elders what would be the smallest amount they could spend in aacms per month to maintain roads that would connect all the villages. The villages are labeled A through I in the maps above. The map on the right shows the roads that could be maintained most cheaply, for 216 aacms per month. Your task is to write a program that will solve such problems. 

The input consists of one to 100 data sets, followed by a final line containing only 0. Each data set starts with a line containing only a number n, which is the number of villages, 1 < n < 27, and the villages are labeled with the first n letters of the alphabet, capitalized. Each data set is completed with n-1 lines that start with village labels in alphabetical order. There is no line for the last village. Each line for a village starts with the village label followed by a number, k, of roads from this village to villages with labels later in the alphabet. If k is greater than 0, the line continues with data for each of the k roads. The data for each road is the village label for the other end of the road followed by the monthly maintenance cost in aacms for the road. Maintenance costs will be positive integers less than 100. All data fields in the row are separated by single blanks. The road network will always allow travel between all the villages. The network will never have more than 75 roads. No village will have more than 15 roads going to other villages (before or after in the alphabet). In the sample input below, the first data set goes with the map above. 

The output is one integer per line for each data set: the minimum cost in aacms per month to maintain a road system that connect all the villages. Caution: A brute force solution that examines every possible set of roads will not finish within the one minute time limit. 

 

Sample Input

9
A 2 B 12 I 25
B 3 C 10 H 40 I 8
C 2 D 18 G 55
D 1 E 44
E 2 F 60 G 38
F 0
G 1 H 35
H 1 I 35
3
A 2 B 10 C 40
B 1 C 20
0

 

Sample Output

216
30

 

Source

Mid-Central USA 2002

 

给出n，表示有n个点，然后接下来的n行，第一个字符表示一个端点序号，第二个表示跟它相连的边数量k，然后紧跟着k组数，分别表示边的另一端点序号，和与当前端点的距离。求出将所有点连通最小花费。

#include<iostream>
#include<algorithm>
#include<cstring>
#include<cstdio>
#define MAX 5005
int pa[MAX];
int m,n,t,r;
using namespace std;
struct edge
{
    int beg,en,r;
};
edge edges[MAX];
bool cmp(edge x,edge y)
{
    return x.r<y.r;
}
int dfs(int x)
{
    if(pa[x]!=x)pa[x]=dfs(pa[x]);
    return pa[x];
}
int kruskal()
{
    int ans=0;
    sort(edges,edges+m,cmp);
    for(int i=0;i<26;i++)
        pa[i]=i;
    for(int i=0;i<m;i++)
    {
        int beg=edges[i].beg,en=edges[i].en;
        if(dfs(beg)!=dfs(en))
        {
            pa[dfs(beg)]=dfs(en);
            ans+=edges[i].r;
        }
    }
   return ans;
}
//n:节点数目 m:边数目
int main()
{
   while(scanf("%d",&n),n)
   {
     m=0;int num,r;
     char beg[5],en[5];
     for(int i=0;i<n-1;i++)
     {
        scanf("%s%d",beg,&num);
         while(num--)
         {
             scanf("%s%d",en,&r);
             edges[m].beg=beg[0]-'A';
             edges[m].en=en[0]-'A';
             edges[m++].r=r;
         }
     }
     printf("%d
",kruskal());
   }
}

版权声明：本文为博主原创文章，未经博主允许不得转载。