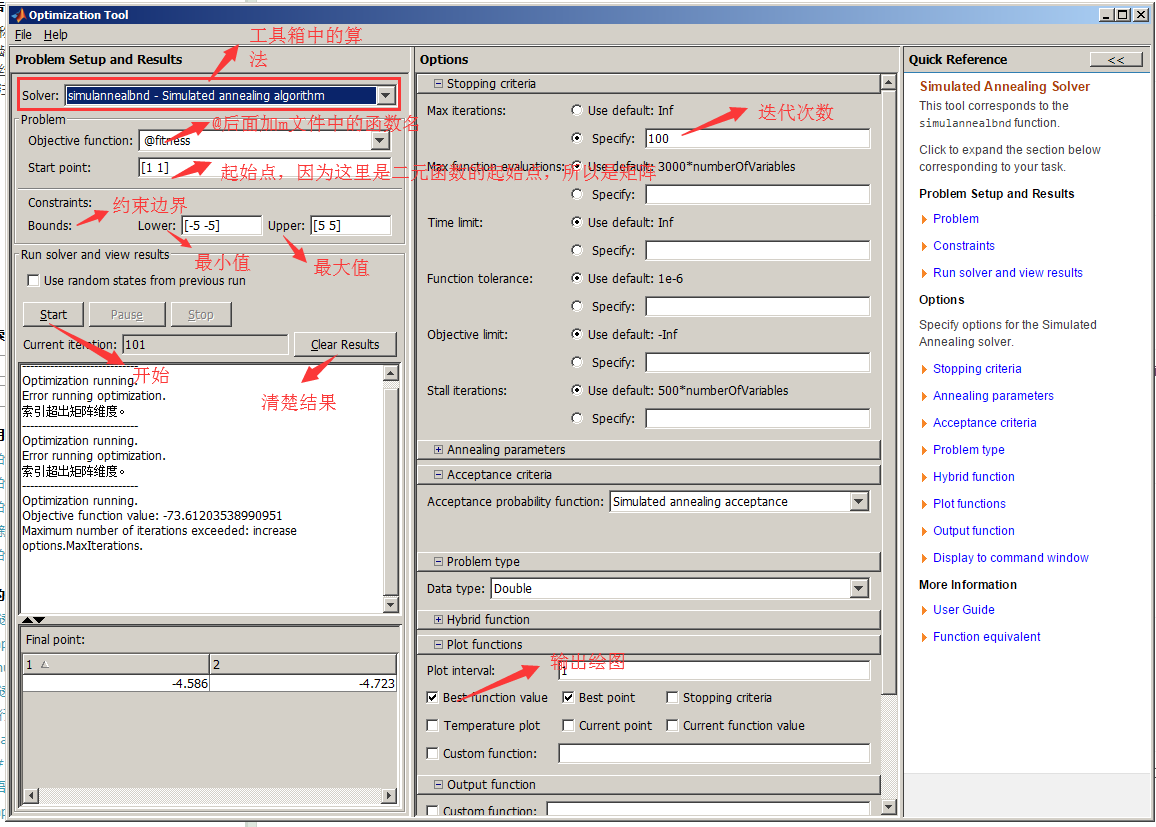
optimtool %打开工具箱,工具箱界面如下:
fitness函数如下:
function fitnessVal = fitness( x ) % fitnessVal = sin(10*pi*x) / x; % fitnessVal = -1 * sin(10*pi*x) / x; fitnessVal = -1 * (x(1)^2 + x(2).^2 - 10*cos(2*pi*x(1)) - 10*cos(2*pi*x(2)) + 20); end
%%主函数
% I. 清空环境变量
clear all
clc
%% II. 一元函数优化
x = 1:0.01:2;
y = sin(10*pi*x) ./ x;
figure
plot(x,y,'linewidth',1.5)
ylim([-1.5, 1.5]) %产生伪随机序列
xlabel('x')
ylabel('y')
title('y = sin(10*pi*x) / x')
hold on
%%
% 1. 标记出最大值点
[maxVal,maxIndex] = max(y);
plot(x(maxIndex), maxVal, 'r*','linewidth',2)
text(x(maxIndex), maxVal, {[' X: ' num2str(x(maxIndex))];[' Y: ' num2str(maxVal)]}) %num2str:将数字类型转换为字符串类型
hold on
%%
% 2. 标记出最小值点
[minVal,minIndex] = min(y);
plot(x(minIndex), minVal, 'ks','linewidth',2)
text(x(minIndex), minVal, {[' X: ' num2str(x(minIndex))];[' Y: ' num2str(minVal)]})
%% III. 二元函数优化
[x,y] = meshgrid(-5:0.1:5,-5:0.1:5);
z = x.^2 + y.^2 - 10*cos(2*pi*x) - 10*cos(2*pi*y) + 20;
figure
mesh(x,y,z) %mesh:画3-D图形
hold on
xlabel('x')
ylabel('y')
zlabel('z')
title('z = x^2 + y^2 - 10*cos(2*pi*x) - 10*cos(2*pi*y) + 20')
%%
% 1. 标记出最大值点
maxVal = max(z(:));
[maxIndexX,maxIndexY] = find(z == maxVal);
for i = 1:length(maxIndexX)
plot3(x(maxIndexX(i),maxIndexY(i)),y(maxIndexX(i),maxIndexY(i)), maxVal, 'r*','linewidth',2)
text(x(maxIndexX(i),maxIndexY(i)),y(maxIndexX(i),maxIndexY(i)), maxVal, {[' X: ' num2str(x(maxIndexX(i),maxIndexY(i)))];[' Y: ' num2str(y(maxIndexX(i),maxIndexY(i)))];[' Z: ' num2str(maxVal)]})
hold on
end