Gorgeous Sequence
Time Limit: 6000/3000 MS (Java/Others) Memory Limit: 131072/131072 K (Java/Others)
Total Submission(s): 6946 Accepted Submission(s): 1784
Problem Description
There is a sequence a of length n. We use ai to denote the i-th element in this sequence. You should do the following three types of operations to this sequence.
0 x y t: For every x≤i≤y, we use min(ai,t) to replace the original ai's value.
1 x y: Print the maximum value of ai that x≤i≤y.
2 x y: Print the sum of ai that x≤i≤y.
0 x y t: For every x≤i≤y, we use min(ai,t) to replace the original ai's value.
1 x y: Print the maximum value of ai that x≤i≤y.
2 x y: Print the sum of ai that x≤i≤y.
Input
The first line of the input is a single integer T, indicating the number of testcases.
The first line contains two integers n and m denoting the length of the sequence and the number of operations.
The second line contains n separated integers a1,…,an (∀1≤i≤n,0≤ai<231).
Each of the following m lines represents one operation (1≤x≤y≤n,0≤t<231).
It is guaranteed that T=100, ∑n≤1000000, ∑m≤1000000.
The first line contains two integers n and m denoting the length of the sequence and the number of operations.
The second line contains n separated integers a1,…,an (∀1≤i≤n,0≤ai<231).
Each of the following m lines represents one operation (1≤x≤y≤n,0≤t<231).
It is guaranteed that T=100, ∑n≤1000000, ∑m≤1000000.
Output
For every operation of type 1 or 2, print one line containing the answer to the corresponding query.
Sample Input
1
5 5
1 2 3 4 5
1 1 5
2 1 5
0 3 5 3
1 1 5
2 1 5
Sample Output
5
15
3
12
Hint
Please use efficient IO methodAuthor
XJZX
Source
Recommend
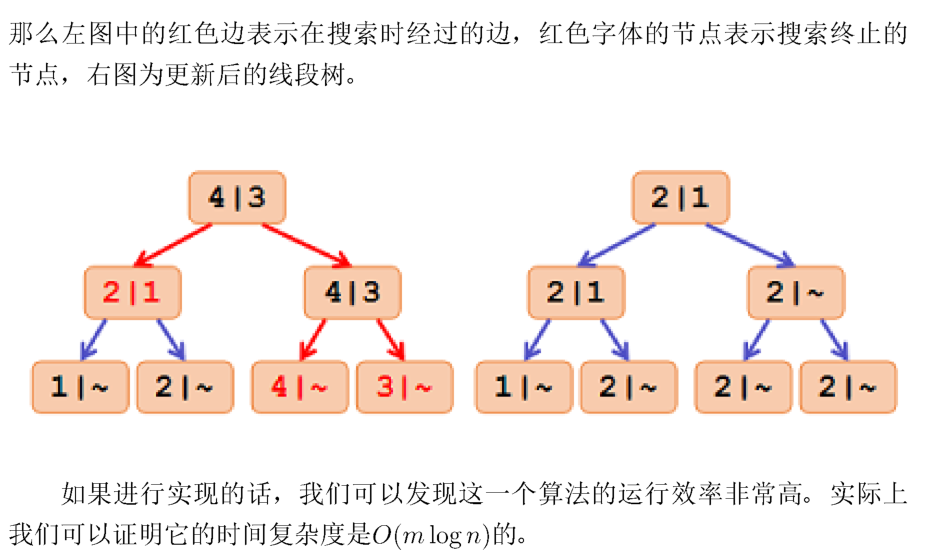
题意 :
有一个长度为n的序列a。我们用ai来表示这个序列中的第i个元素。您应该对这个序列执行以下三种类型的操作。
0 x y t:对于每一个x≤i≤y,我们用min(ai,t)替换原始ai的值。
1 x y:打印ai的最大值,即x≤i≤y。
2xy:输出x≤i≤y的ai之和。
输入
输入的第一行是一个整数T,表示测试用例的数量。
第一行包含两个整数n和m,表示序列的长度和操作的数量。
第二行包含n分离整数a1,…,一个(∀1≤≤n, 0≤ai < 231)。
以下m行每一行表示一个操作(1≤x≤y≤n,0≤t<231)。
保证T=100,∑n≤1000000,∑m≤1000000。
输出
对于类型1或2的每个操作,打印一行包含相应查询的答案。
题解:

c++代码

#include <bits/stdc++.h> using namespace std; typedef long long ll; const int maxn = 1e6 + 7; int a[maxn]; #define EF if(ch==EOF) return x; // #define lc k<<1 // #define rc k<<1|1 inline int read(){ int x=0,f=1;char ch=getchar(); while(ch<'0'||ch>'9'){if(ch=='-')f=-1;EF;ch=getchar();} while(ch>='0'&&ch<='9'){x=x*10+ch-'0';ch=getchar();} return x*f; } struct tree { int l , r; int miax , maxx; ll sum; int set_lazy; }t[maxn << 2]; // inline void push_up(int k){ // // sum[k]=sum[lc]+sum[rc]; // // mx[k]=max(mx[lc],mx[rc]); // // se[k]=max(se[lc],se[rc]); // // mc[k]=0; // // if(mx[lc]!=mx[rc]) se[k]=max(se[k],min(mx[lc],mx[rc])); // // if(mx[k]==mx[lc]) mc[k]+=mc[lc]; // // if(mx[k]==mx[rc]) mc[k]+=mc[rc]; // t[k].sum = t[lc].sum + t[rc].sum; // t[k].maxx = max(t[lc].maxx,t[rc].maxx); // t[k].miax = max(t[lc].miax,t[rc].miax); // t[k].set_lazy = 0; // if(t[lc].maxx != t[rc].maxx) t[k].miax = max(t[k].miax,min(t[lc].maxx,t[rc].maxx)); // if(t[k].maxx == t[lc].maxx) t[k].set_lazy += t[lc].set_lazy; // if(t[k].maxx == t[rc].maxx) t[k].set_lazy += t[rc].set_lazy; // } inline void push_up(int rt){ t[rt].sum = t[rt << 1].sum + t[rt << 1|1].sum; // t[rt].minn = min(t[rt << 1].minn,t[rt << 1|1].minn); t[rt].maxx = max(t[rt << 1].maxx,t[rt << 1|1].maxx); t[rt].miax = max(t[rt << 1].miax, t[rt << 1|1].miax); t[rt].set_lazy = 0; if(t[rt << 1].maxx != t[rt <<1|1].maxx) t[rt].miax = max(t[rt].miax,min(t[rt << 1].maxx , t[rt <<1|1].maxx)); //打上标记,记录下标记个数 if(t[rt].maxx == t[rt << 1].maxx) t[rt].set_lazy += t[rt << 1].set_lazy; if(t[rt].maxx == t[rt << 1|1].maxx) t[rt].set_lazy += t[rt << 1|1].set_lazy; // cout << t[rt].sum << " " << rt <<endl; } inline void dec_tag(int rt,int v){ if(v >= t[rt].maxx) return; t[rt].sum += 1ll * (v - t[rt].maxx)*t[rt].set_lazy; t[rt].maxx = v; } inline void push_down(int rt){ dec_tag(rt << 1,t[rt].maxx); dec_tag(rt << 1|1,t[rt].maxx); } // inline void push_down(int rt) { // if(t[rt].set_lazy) { ///if set_lazy add_lazy = 0 // t[rt<<1].set_lazy = t[rt].set_lazy; // t[rt<<1].sum = (t[rt<<1].r - t[rt<<1].l + 1) * t[rt].set_lazy; // t[rt<<1].maxx = t[rt].set_lazy; // t[rt<<1].minn = t[rt].set_lazy; // t[rt<<1|1].set_lazy = t[rt].set_lazy; // t[rt<<1|1].sum = (t[rt<<1|1].r - t[rt<<1|1].l + 1) * t[rt].set_lazy; // t[rt<<1|1].maxx = t[rt].set_lazy; // t[rt<<1|1].minn = t[rt].set_lazy; // //tre[rt].add_lazy = 0; // //tre[rt<<1].add_lazy = tre[rt<<1|1].add_lazy = 0; // t[rt].set_lazy = 0; // return ; // } // // if(tre[rt].add_lazy) { // // tre[rt<<1].add_lazy += tre[rt].add_lazy; // // tre[rt<<1].sum += (tre[rt<<1].r - tre[rt<<1].l + 1) * tre[rt].add_lazy; // // tre[rt<<1].max += tre[rt].add_lazy; // // tre[rt<<1].min += tre[rt].add_lazy; // // tre[rt<<1|1].add_lazy += tre[rt].add_lazy; // // tre[rt<<1|1].sum += (tre[rt<<1|1].r - tre[rt<<1|1].l + 1) * // // tre[rt].add_lazy; // // tre[rt<<1|1].max += tre[rt].add_lazy; // // tre[rt<<1|1].min += tre[rt].add_lazy; // // tre[rt].add_lazy = 0; // // } // } void build(int rt,int l ,int r){ t[rt].l = l, t[rt].r = r; //t[rt].set_lazy = 1; if(l == r){ t[rt].sum = t[rt].maxx = a[l]; t[rt].miax = -1; t[rt].set_lazy = 1; // cout <<"nbb " <<t[rt].sum << " "<<rt << endl; return; } int mid = (l + r) >> 1; build(rt <<1,l,mid); build(rt << 1|1,mid + 1,r); push_up(rt); } void up_date(int rt,int l,int r,int d){ if(d >= t[rt].maxx) return; //push_down(rt); if(l <= t[rt].l && r >= t[rt].r && d > t[rt].miax){ // t[rt].sum = (t[rt].r - t[rt].l + 1) * d; // t[rt].maxx = d; // t[rt].minn = d; // t[rt].set_lazy = d; dec_tag(rt,d); return; } push_down(rt); int mid = (t[rt].l + t[rt].r) >> 1; if(r <= mid) { up_date(rt<<1,l,r,d); } else if(l > mid) { up_date(rt<<1|1,l,r,d); } else { up_date(rt<<1,l,mid,d); up_date(rt<<1|1,mid+1,r,d); } push_up(rt); } ll query_sum(int rt,int l,int r) { ///sum if(l <= t[rt].l && t[rt].r <= r) { return t[rt].sum; } push_down(rt); int mid = (t[rt].l + t[rt].r) >> 1; if(r <= mid) { return query_sum(rt<<1,l,r); } else if(l > mid) { return query_sum(rt<<1|1,l,r); } else { return query_sum(rt<<1,l,mid) + query_sum(rt<<1|1,mid+1,r); } } int query_max(int rt,int l,int r) { ///max //cout << t[rt].maxx << endl; if(l <= t[rt].l && t[rt].r <= r) { return t[rt].maxx; } push_down(rt); int mid = (t[rt].l + t[rt].r) >> 1; if(r <= mid) { return query_max(rt<<1,l,r); } else if(l > mid) { return query_max(rt<<1|1,l,r); } else { return max(query_max(rt<<1,l,mid), query_max(rt<<1|1,mid+1,r)); } } // int query_min(int rt,int l,int r) { ///min // push_down(rt); // if(l <= t[rt].l && t[rt].r <= r) { // return t[rt].minn; // } // int mid = (t[rt].l + t[rt].r) >> 1; // if(r <= mid) { // return query_min(rt<<1,l,r); // } else if(l > mid) { // return query_min(rt<<1|1,l,r); // } else { // return min(query_min(rt<<1,l,mid), query_min(rt<<1|1,mid+1,r)); // } // } int main(int argc, char const *argv[]) { int t; //scanf("%d",&t); t = read(); while(t--){ int n , q; //scanf("%d%d",&n,&q); n = read(),q = read(); for(int i = 1;i <= n; i++) //scanf("%d",&a[i]); a[i] = read(); build(1,1,n); // //0 cout << 1; // for(int i = 1;i <= n; i++){ // cout << t[i].maxx ; // } while(q--){ int a,b,c; //scanf("%d%d%d",&a,&b,&c); a = read() ,b = read() , c = read(); if(a == 0){ int z; //scanf("%d",&z); z = read(); up_date(1,b,c,z); } else if(a == 1){ printf("%d ",query_max(1,b,c)); } else{ printf("%lld ",query_sum(1,b,c)); } } } return 0; }
