• 图及其遍历

    图——深度优先和广度优先算法

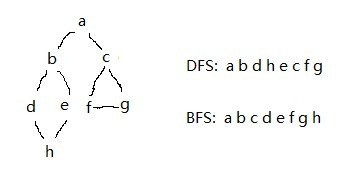
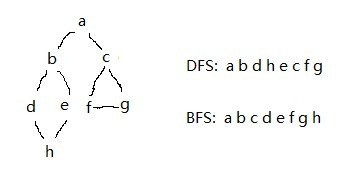
    无向图用二维邻接矩阵表示

    测试环境：VC 6.0 (C)

    [cpp:showcolumns] view plaincopyprint?
    ·········10········20········30········40········50········60········70········80········90········100·······110·······120·······130·······140·······150
    1. #include <stdio.h>  
    2. #include <malloc.h>  
    3. #include <stdlib.h>  
    4. #define INFINITY 32767  
    5. #define MAX_VEX 20  
    6. #define QUEUE_SIZE (MAX_VERTEX+1)  
    7. #define DataType char  /* vertext's info  */  
    8. int *visited; /* Node: visited flag with dynamic array, good idea ! */  
    9. /* init queue for bfs */  
    10. struct _node  
    11. {  
    12.     int v_num;  
    13.     struct _node *next;  
    14. };  
    15. typedef struct _node node, *pnode;  
    16. struct _queue  
    17. {  
    18.     pnode front;  
    19.     pnode rear;  
    20. };  
    21. typedef struct _queue queue, *pqueue;  
    22. struct _graph  
    23. {  
    24.     DataType *vexs;  
    25.     int arcs[MAX_VEX][MAX_VEX];  
    26.     int vexnum, arcnum;  
    27. };  
    28. typedef struct _graph graph, *pgraph;  
    29. /* operation of queue */  
    30. queue init_queue()  
    31. {  
    32.     queue qu;  
    33.     qu.front=qu.rear=(pnode)malloc(sizeof(node));  
    34.     if(qu.front == NULL)  
    35.         exit(1);  
    36.     qu.rear->next=NULL;  
    37.     return qu;  
    38. }  
    39. void en_queue(pqueue pqu, int v_num)  
    40. {  
    41.     pnode pn;  
    42.     pn=(pnode)malloc(sizeof(node));  
    43.     if(pqu->front == NULL)  
    44.         exit(1);  
    45.     pn->v_num=v_num;  
    46.     pn->next=NULL;  
    47.     pqu->rear->next=pn;  
    48.     pqu->rear=pqu->rear->next;  
    49. }  
    50. int isempty_queue(pqueue pqu)  
    51. {  
    52.     if(pqu->front == pqu->rear)  
    53.         return 1;  
    54.     else  
    55.         return 0;  
    56. }  
    57. int de_queue(pqueue pqu)  
    58. {  
    59.     pnode pn;  
    60.     int d;  
    61.     if(isempty_queue(pqu))  
    62.         return -1;  
    63.     pn=pqu->front;  
    64.     d=pn->v_num;  
    65.     pqu->front=pn->next;  
    66.     free(pn);  
    67.     return d;  
    68. }  
    69. int locate(graph g, DataType data)  
    70. {  
    71.     int i;  
    72.     for(i=0;i<g.vexnum;i++)  
    73.         if(g.vexs[i] == data)  
    74.             return i;  
    75.      return -1;  
    76. }  
    77. graph create_graph()  
    78. {  
    79.     int i,j,w, s1,s2;  
    80.     DataType ch1,ch2,tmp;  
    81.     graph g;  
    82.     printf("g sizeof: %d/n", sizeof(g));  
    83.     printf("Enter vexnum arcnum:");  
    84.     scanf("%d %d", &g.vexnum, &g.arcnum);  
    85.     tmp=getchar();  
    86.     g.vexs=(DataType *)malloc(sizeof(DataType));  
    87.     if(g.vexs == NULL)  
    88.         exit(1);  
    89.     printf("Enter %d vertext,please.../n", g.vexnum);  
    90.     for(i=0;i<g.vexnum;i++)  
    91.     {  
    92.         printf("vex %d: ", i);  
    93.         scanf("%c", &g.vexs[i]);  
    94.         tmp=getchar();  
    95.         //visited[i]=0;  
    96.     }  
    97.     for(i=0;i<g.vexnum;i++)  
    98.         for(j=0;j<g.vexnum;j++)  
    99.             g.arcs[i][j]=INFINITY;  
    100.      printf("Enter %d arcs:/n", g.arcnum);  
    101.      for(i=0;i<g.arcnum;i++)  
    102.      {  
    103.         printf("arc %d: ", i);  
    104.         scanf("%c %c %d", &ch1, &ch2, &w);  
    105.         tmp=getchar();  
    106.         s1=locate(g, ch1);  
    107.         s2=locate(g, ch2);  
    108.         g.arcs[s1][s2]=g.arcs[s2][s1]=w; /* NOTE: weight */  
    109.      }  
    110.      return g;  
    111. }  
    112. int firstvex_graph(graph g, int k)  
    113. {  
    114.     int i;  
    115.     if(k>=0 && k<g.vexnum)  
    116.         for(i=0;i<g.vexnum;i++)  
    117.             if(g.arcs[k][i] != INFINITY)  
    118.                 return i;  
    119.      return -1;  
    120. }  
    121. int nextvex_graph(graph g, int i, int j)  
    122. {  
    123.     int k;  
    124.     if(i>=0 && i<g.vexnum && j>=0 && j<g.vexnum)  
    125.         for(k=j+1; k<g.vexnum; k++)  
    126.             if(g.arcs[i][k] != INFINITY)  
    127.                 return k;  
    128.      return -1;  
    129. }  
    130. void dfs(graph g, int k)  
    131. {  
    132.     int i;  
    133.     if(k == -1)  
    134.     {  
    135.         for(i=0;i<g.vexnum;i++)  
    136.             if(!visited[i])  
    137.                 dfs(g,i);  
    138.      }  
    139.      else  
    140.      {  
    141.         visited[k]=1;  
    142.         printf("%c ", g.vexs[k]);  
    143.         for(i=firstvex_graph(g,k);i>=0;i=nextvex_graph(g,k,i))  
    144.             if(!visited[i])  
    145.                 dfs(g,i);  
    146.      }  
    147. }  
    148. void bfs(graph g)  
    149. {  
    150.     int i,j,k;  
    151.     queue qu;  
    152.     qu=init_queue();  
    153.     for(i=0;i<g.vexnum;i++)  
    154.         if(!visited[i])  
    155.         {  
    156.             visited[i] =1;  
    157.             printf("%c ", g.vexs[i]);  
    158.             en_queue(&qu, i);  
    159.             while(!isempty_queue(&qu))  
    160.             {  
    161.                 k=de_queue(&qu);  
    162.                 for(j=firstvex_graph(g,k); j>=0;j=nextvex_graph(g,k,j))  
    163.                     if(!visited[j])  
    164.                     {  
    165.                         visited[j]=1;  
    166.                         printf("%c ", g.vexs[j]);  
    167.                         en_queue(&qu, j);  
    168.                     }  
    169.             }  
    170.         }  
    171. }  
    172. void main()  
    173. {  
    174.     int i;  
    175.     graph g;  
    176.     g=create_graph();  
    177.     visited=(int *)malloc(g.vexnum*sizeof(int));  
    178.     for(i=0;i<g.vexnum;i++)  
    179.         visited[i]=0;  
    180.     printf("/n/n dfs:");  
    181.     dfs(g,-1);  
    182.     for(i=0;i<g.vexnum;i++)  
    183.         visited[i]=0;  
    184.     printf("/n bfs:");  
    185.     bfs(g);  
    186.       
    187.     if(visited)  
    188.         free(visited);  
    189.     printf("/n");  
    190. }  

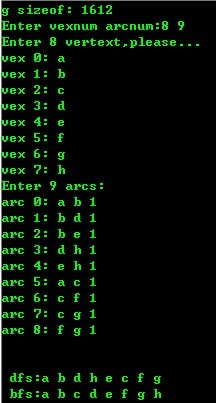
    运行结果：

          

    ======================================================

    图 ——深度优先

    测试环境：VS2008 (C)

    [cpp:showcolumns] view plaincopyprint?
    ·········10········20········30········40········50········60········70········80········90········100·······110·······120·······130·······140·······150
    1. #include "stdafx.h"  
    2. #include <stdlib.h>  
    3. #include <malloc.h>  
    4. #define MAX_VEX 20  
    5. #define INFINITY 65535  
    6. int *visited;  
    7. struct _node  
    8. {  
    9.     int vex_num;  
    10.     struct _node *next;  
    11. };  
    12. typedef struct _node node, *pnode;  
    13. struct _graph  
    14. {  
    15.     char *vexs;  
    16.     int arcs[MAX_VEX][MAX_VEX];  
    17.     int vexnum, arcnum;  
    18. };  
    19. typedef struct _graph graph, *pgraph;  
    20. int locate(graph g, char ch)  
    21. {  
    22.     int i;  
    23.     for(i=1; i<=g.vexnum; i++)  
    24.         if(g.vexs[i]==ch)  
    25.             return i;  
    26.     return -1;  
    27. }  
    28. graph create_graph()  
    29. {  
    30.     int i, j, w, p1, p2;  
    31.     char ch1, ch2;  
    32.     graph g;  
    33.     printf("Enter vexnum arcnum: ");  
    34.     scanf("%d %d", &g.vexnum, &g.arcnum);  
    35.     getchar();  
    36.     for(i=1; i<=g.vexnum; i++)  
    37.         for(j=1; j<g.vexnum; j++)  
    38.             g.arcs[i][j]=INFINITY;  
    39.     g.vexs=(char *)malloc(sizeof(char));  
    40.     printf("Enter %d vexnum.../n", g.vexnum);  
    41.     for(i=1; i<=g.vexnum; i++)  
    42.     {  
    43.         printf("vex %d: ", i);  
    44.         scanf("%c", &g.vexs[i]);  
    45.         getchar();  
    46.     }  
    47.     printf("Enter %d arcnum.../n", g.arcnum);  
    48.     for(i=1; i<=g.arcnum; i++)  
    49.     {  
    50.         printf("arc %d: ", i);  
    51.         scanf("%c %c %d", &ch1, &ch2, &w);  
    52.         getchar();  
    53.         p1=locate(g, ch1);  
    54.         p2=locate(g, ch2);  
    55.         g.arcs[p1][p2]=g.arcs[p2][p1]=w;  
    56.     }  
    57.     return g;  
    58. }  
    59. int firstvex_graph(graph g, int i)  
    60. {  
    61.     int k;  
    62.     if(i>=1 && i<=g.vexnum)  
    63.         for(k=1; k<=g.vexnum; k++)  
    64.             if(g.arcs[i][k]!=INFINITY)  
    65.                 return k;  
    66.     return -1;  
    67. }  
    68. int nextvex_graph(graph g, int i, int j)  
    69. {  
    70.     int k;  
    71.     if(i>=1 && i<=g.vexnum && j>=1 && j<=g.vexnum)  
    72.         for(k=j+1; k<=g.vexnum; k++)  
    73.             if(g.arcs[i][k]!=INFINITY)  
    74.                 return k;  
    75.     return -1;  
    76. }  
    77. void dfs(graph g, int i)  
    78. {  
    79.     int k, j;  
    80.     if(!visited[i])  
    81.     {  
    82.         visited[i]=1;  
    83.         printf("%c", g.vexs[i]);  
    84.         for(j=firstvex_graph(g, i); j>=1; j=nextvex_graph(g, i, j))  
    85.             if(!visited[j])  
    86.                 dfs(g, j);  
    87.     }  
    88. }  
    89. void dfs_graph(graph g)  
    90. {  
    91.     int i;  
    92.     visited=(int *)malloc((g.vexnum+1)*sizeof(int));  
    93.     for(i=1; i<=g.vexnum; i++)  
    94.         visited[i]=0;  
    95.     for(i=1; i<g.vexnum; i++)  
    96.         if(!visited[i])  
    97.             dfs(g, i);  
    98. }  
    99. int _tmain(int argc, _TCHAR* argv[])  
    100. {  
    101.     graph g;  
    102.     g=create_graph();  
    103.     dfs_graph(g);  
    104.     printf("/n");  
    105.     return 0;  
    106. }  

    ======================================================

    图 ——广度优先

    测试环境：VS2008 (C)

    [cpp:showcolumns] view plaincopyprint?
    ·········10········20········30········40········50········60········70········80········90········100·······110·······120·······130·······140·······150
    1. #include "stdafx.h"  
    2. #include <stdlib.h>  
    3. #include <malloc.h>  
    4. #define MAX_VEX 20  
    5. #define INFINITY 65535  
    6. int *visited;  
    7. struct _node  
    8. {  
    9.     int data;  
    10.     struct _node *next;  
    11. };  
    12. typedef struct _node node, *pnode;  
    13. struct _queue  
    14. {  
    15.     pnode front;  
    16.     pnode rear;  
    17. };  
    18. typedef struct _queue queue, *pqueue;  
    19. queue init_queue()  
    20. {  
    21.     pnode pn=NULL;  
    22.     queue qu;  
    23.     pn=(pnode)malloc(sizeof(node));  
    24.     if(pn==NULL)  
    25.         printf("init queue, malloc is fail.../n");  
    26.     pn->data=-1;  
    27.     pn->next=NULL;  
    28.     qu.front=qu.rear=pn;  
    29.     return qu;  
    30. }  
    31. int empty_queue(queue qu)  
    32. {  
    33.     if(qu.rear==qu.front)  
    34.         return 0;  
    35.     else  
    36.         return 1;  
    37. }  
    38. void en_queue(pqueue pqu, int data)  
    39. {  
    40.     pnode pn=NULL;  
    41.     if(pqu->rear==NULL)  
    42.         return;  
    43.     pn=(pnode)malloc(sizeof(node));  
    44.     pn->data=data;  
    45.     pn->next=pqu->rear->next;  
    46.     pqu->rear->next=pn;  
    47.     pqu->rear=pn;  
    48. }  
    49. int de_queue(pqueue pqu)  
    50. {  
    51.     int data;  
    52.     pnode pn=NULL;  
    53.     if(pqu->front->next==NULL)  
    54.         return -1;  
    55.       
    56.     pn=pqu->front->next;  
    57.     pqu->front=pqu->front->next;  
    58.     data=pn->data;  
    59.     free(pn);  
    60.     return data;  
    61. }  
    62. struct _graph  
    63. {  
    64.     char *vexs;  
    65.     int arcs[MAX_VEX][MAX_VEX];  
    66.     int vexnum, arcnum;  
    67. };  
    68. typedef _graph graph, *pgraph;  
    69. int locate(graph g, char ch)  
    70. {  
    71.     int i;  
    72.     for(i=1; i<=g.vexnum; i++)  
    73.         if(g.vexs[i]==ch)  
    74.             return i;  
    75.     return -1;  
    76. }  
    77. graph create_graph()  
    78. {  
    79.     int i, j, w, p1, p2;  
    80.     char ch1, ch2;  
    81.     graph g;  
    82.     printf("Enter vexnum arcnum: ");  
    83.     scanf("%d %d", &g.vexnum, &g.arcnum);  
    84.     getchar();  
    85.     for(i=1; i<=g.vexnum; i++)  
    86.         for(j=1; j<g.vexnum; j++)  
    87.             g.arcs[i][j]=INFINITY;  
    88.     g.vexs=(char *)malloc((g.vexnum+1)*sizeof(char));  
    89.     printf("Enter %d vexnum.../n", g.vexnum);  
    90.     for(i=1; i<=g.vexnum; i++)  
    91.     {  
    92.         printf("vex %d: ", i);  
    93.         scanf("%c", &g.vexs[i]);  
    94.         getchar();  
    95.     }  
    96.     printf("Enter %d arcnum.../n", g.arcnum);  
    97.     for(i=1; i<=g.arcnum; i++)  
    98.     {  
    99.         printf("arc %d: ", i);  
    100.         scanf("%c %c %d", &ch1, &ch2, &w);  
    101.         getchar();  
    102.         p1=locate(g, ch1);  
    103.         p2=locate(g, ch2);  
    104.         g.arcs[p1][p2]=g.arcs[p2][p1]=w;  
    105.     }  
    106.     return g;  
    107. }  
    108. int firstvex_graph(graph g, int i)  
    109. {  
    110.     int k;  
    111.     if(i>=1 && i<=g.vexnum)  
    112.         for(k=1; k<=g.vexnum; k++)  
    113.             if(g.arcs[i][k]!=INFINITY)  
    114.                 return k;  
    115.     return -1;  
    116. }  
    117. int nextvex_graph(graph g, int i, int j)  
    118. {  
    119.     int k;  
    120.     if(i>=1 && i<=g.vexnum && j>=1 && j<=g.vexnum)  
    121.         for(k=j+1; k<=g.vexnum; k++)  
    122.             if(g.arcs[i][k]!=INFINITY)  
    123.                 return k;  
    124.     return -1;  
    125. }  
    126. void bfs(graph g)  
    127. {  
    128.     int i, ivex, inextvex;  
    129.     visited=(int *)malloc((g.vexnum+1)*sizeof(int));  
    130.     for(i=1; i<=g.vexnum; i++)  
    131.         visited[i]=0;  
    132.     queue qu=init_queue();  
    133.     for(i=1; i<=g.vexnum; i++)  
    134.     {  
    135.         if(!visited[i])  
    136.         {  
    137.             visited[i]=1;  
    138.             printf("%c", g.vexs[i]);  
    139.             en_queue(&qu, i);  
    140.         }  
    141.           
    142.         while(!empty_queue(qu))  
    143.         {  
    144.             ivex=de_queue(&qu);  
    145.             for(inextvex=firstvex_graph(g, ivex); inextvex>=1; inextvex=nextvex_graph(g, ivex, inextvex))  
    146.                 if(!visited[inextvex])  
    147.                 {  
    148.                     visited[inextvex]=1;  
    149.                     printf("%c", g.vexs[inextvex]);  
    150.                     en_queue(&qu, inextvex);  
    151.                 }  
    152.         }  
    153.     }  
    154. }  
    155. int _tmain(int argc, _TCHAR* argv[])  
    156. {  
    157.     graph g;  
    158.     g=create_graph();  
    159.     bfs(g);  
    160.     printf("/n");  
    161.     return 0;  
    162. }  

     

    ======================================================

    图 ——深度优先和广度优先算法2（网摘）

    本文引用网址：http://bbs.bccn.net/thread-155311-1-1.html（编程论坛）

    看到本算法在网上转载较多，比较流行，且能直接运行

    但发现大多转载中，也把DFS与BFS正好写反了，对此本文已修正

    此外，本算法混用了C与C++，不够单纯，申请的指针空间也未及时释放

    测试环境：VC 6.0 (C)

    [cpp:showcolumns] view plaincopyprint?
    ·········10········20········30········40········50········60········70········80········90········100·······110·······120·······130·······140·······150
    1. #include <stdio.h>  
    2. #include <malloc.h>  
    3. #define INFINITY 32767  
    4. #define MAX_VEX 20   
    5. #define QUEUE_SIZE (MAX_VEX+1)   
    6. bool *visited;    
    7. typedef struct  
    8. {  
    9.     char *vexs;                    //顶点向量  
    10.     int arcs[MAX_VEX][MAX_VEX];    //邻接矩阵  
    11.     int vexnum,arcnum;             //图的当前顶点数和弧数  
    12. }Graph;  
    13. //队列类  
    14. class Queue  
    15. {  
    16.     public:  
    17.     void InitQueue(){  
    18.     base=(int *)malloc(QUEUE_SIZE*sizeof(int));  
    19.     front=rear=0;  
    20. }  
    21. void EnQueue(int e)  
    22. {  
    23.     base[rear]=e;  
    24.     rear=(rear+1)%QUEUE_SIZE;  
    25. }  
    26. void DeQueue(int &e)  
    27. {  
    28.     e=base[front];  
    29.     front=(front+1)%QUEUE_SIZE;  
    30. }  
    31. public:  
    32.     int *base;  
    33.     int front;  
    34.     int rear;  
    35. };  
    36. //图G中查找元素c的位置  
    37. int Locate(Graph G,char c)  
    38. {  
    39.     for(int i=0;i<G.vexnum;i++)  
    40.         if(G.vexs[i]==c)   
    41.             return i;  
    42.       
    43.     return -1;  
    44. }  
    45. //创建无向网  
    46. void CreateUDN(Graph &G){  
    47.     int i,j,w,s1,s2;  
    48.     char a,b,temp;  
    49.     printf("输入顶点数和弧数: ");  
    50.     scanf("%d%d",&G.vexnum,&G.arcnum);  
    51.     temp=getchar(); //接收回车  
    52.     G.vexs=(char *)malloc(G.vexnum*sizeof(char)); //分配顶点数目  
    53.     printf("输入%d个顶点./n",G.vexnum);  
    54.     for(i=0;i<G.vexnum;i++) //初始化顶点  
    55.     {   
    56.         printf("输入顶点%d: ",i);  
    57.         scanf("%c",&G.vexs[i]);  
    58.         temp=getchar(); //接收回车   
    59.     }  
    60.     for(i=0;i<G.vexnum;i++) //初始化邻接矩阵  
    61.         for(j=0;j<G.vexnum;j++)  
    62.             G.arcs[i][j]=INFINITY;  
    63.     printf("输入%d条弧./n",G.arcnum);  
    64.     for(i=0;i<G.arcnum;i++)  
    65.     { //初始化弧  
    66.         printf("输入弧%d: ",i);  
    67.         scanf("%c %c %d",&a,&b,&w); //输入一条边依附的顶点和权值  
    68.         temp=getchar(); //接收回车  
    69.         s1=Locate(G,a);  
    70.         s2=Locate(G,b);  
    71.         G.arcs[s1][s2]=G.arcs[s2][s1]=w;  
    72.     }  
    73. }  
    74. //图G中顶点k的第一个邻接顶点  
    75. int FirstVex(Graph G,int k)  
    76. {  
    77.     if(k>=0 && k<G.vexnum) //k合理  
    78.         for(int i=0;i<G.vexnum;i++)  
    79.             if(G.arcs[k][i]!=INFINITY) return i;  
    80.   return -1;  
    81. }  
    82. //图G中顶点i的第j个邻接顶点的下一个邻接顶点  
    83. int NextVex(Graph G,int i,int j)  
    84. {  
    85.     if(i>=0 && i<G.vexnum && j>=0 && j<G.vexnum) //i,j合理  
    86.         for(int k=j+1;k<G.vexnum;k++)  
    87.             if(G.arcs[i][k]!=INFINITY)   
    88.                 return k;  
    89.   return -1;  
    90. }  
    91. //深度优先遍历  
    92. void DFS(Graph G,int k)  
    93. {  
    94.     int i;  
    95.     if(k==-1) //第一次执行DFS时,k为-1  
    96.     {  
    97.         for(i=0;i<G.vexnum;i++)  
    98.             if(!visited[i])   
    99.                 DFS(G,i); //对尚未访问的顶点调用DFS  
    100.     }  
    101.     else  
    102.     {   
    103.         visited[k]=true;  
    104.         printf("%c ",G.vexs[k]); //访问第k个顶点  
    105.         for(i=FirstVex(G,k);i>=0;i=NextVex(G,k,i))  
    106.             if(!visited[i]) //对k的尚未访问的邻接顶点i递归调用DFS  
    107.                 DFS(G,i);   
    108.     }  
    109. }  
    110. //广度优先遍历  
    111. void BFS(Graph G)  
    112. {  
    113.     int k;  
    114.     Queue Q; //辅助队列Q  
    115.     Q.InitQueue();  
    116.     for(int i=0;i<G.vexnum;i++)  
    117.         if(!visited[i]) //i尚未访问  
    118.         {   
    119.             visited[i]=true;  
    120.             printf("%c ",G.vexs[i]);  
    121.              Q.EnQueue(i); //i入列  
    122.             while(Q.front!=Q.rear)  
    123.             {  
    124.                 Q.DeQueue(k); //队头元素出列并置为k  
    125.                 for(int w=FirstVex(G,k);w>=0;w=NextVex(G,k,w))  
    126.                     if(!visited[w]) //w为k的尚未访问的邻接顶点  
    127.                     {   
    128.                         visited[w]=true;  
    129.                         printf("%c ",G.vexs[w]);  
    130.                         Q.EnQueue(w);  
    131.                     }  
    132.             }  
    133.         }  
    134. }  
    135. //主函数  
    136. void main(){  
    137.     int i;  
    138.     Graph G;  
    139.     CreateUDN(G);  
    140.     visited=(bool *)malloc(G.vexnum*sizeof(bool));   
    141.     printf("/n深度优先遍历: ");   
    142.     for(i=0;i<G.vexnum;i++)  
    143.         visited[i]=false;  
    144.     DFS(G,-1); /* NODE: DFS */  
    145.     printf("/n广度优先遍历: ");   
    146.     for(i=0;i<G.vexnum;i++)  
    147.         visited[i]=false;  
    148.     BFS(G); /* NODE: BFS */  
    149.     printf("/n程序结束./n");  
    150. }  

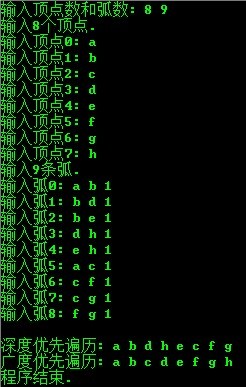
    运行结果：

         

    ======================================================

    [cpp:showcolumns] view plaincopyprint?
    ·········10········20········30········40········50········60········70········80········90········100·······110·······120·······130·······140·······150
    1. #include <iostream.h>   
    2. #include <stdlib.h>   
    3. #define INFINITY 0   
    4. #define MAX_VERTEX_NUM 10 //最大顶点数   
    5. #define MAX_EDGE_NUM 40 //最大边数   
    6. typedef enum {DG,DN,UDG,UDN}Graphkind;   
    7. typedef char VertexType; //顶点数据类型   
    8. typedef struct ArcCell   
    9. {   
    10. int adj; //无权图，1或0表示相邻否；带权图则是权值。   
    11. //int *info;   
    12. }ArcCell,AdjMatrix[MAX_VERTEX_NUM][MAX_VERTEX_NUM];   
    13. typedef struct   
    14. {   
    15. VertexType vexs[MAX_VERTEX_NUM]; //顶点向量   
    16. AdjMatrix arcs; //邻接矩阵   
    17. int vexnum,arcnum; //图的当前顶点数和弧数。   
    18. Graphkind kind;   
    19. }MGraph;   
    20. int LocateVex(MGraph G,VertexType v1)   
    21. {   
    22. int i;   
    23. for(i=0;i<G.vexnum;i++)   
    24. if(G.vexs[i]==v1)   
    25. return i;   
    26. return -1;   
    27. }   
    28. int CreatUDN(MGraph &G)   
    29. // 采用数组表示法，构造无向网 G   
    30. {   
    31. VertexType v1,v2;   
    32. int w,j;   
    33. cout<<"输入图的顶点数"<<endl;   
    34. cin>>G.vexnum;   
    35. cout<<"输入图的弧数"<<endl;   
    36. cin>>G.arcnum;   
    37. for(int i=0;i<G.vexnum;i++)   
    38. {   
    39. cout<<"输入顶点向量"<<endl;   
    40. cin>>G.vexs[i];   
    41. }   
    42. for(i=0;i<G.vexnum;i++)   
    43. for(j=0;j<G.vexnum;j++)   
    44. {   
    45. G.arcs[i][j].adj=INFINITY;   
    46. }   
    47. for(int k=0;k<G.arcnum;++k) //构造邻接矩阵   
    48. {   
    49. cout<<"输入边依附的两个顶点"<<endl;   
    50. cin>>v1>>v2;   
    51. cout<<"输入此边的权值"<<endl;   
    52. cin>>w;   
    53. i=LocateVex(G,v1);   
    54. j=LocateVex(G,v2);   
    55. G.arcs[i][j].adj=w;   
    56. G.arcs[j][i].adj=G.arcs[i][j].adj;   
    57. }   
    58. return 1;   
    59. }   
    60. void dispMGraph(MGraph G)   
    61. {   
    62. cout<<"图的邻接矩阵图是："<<endl;   
    63. for(int i=0;i<G.vexnum;i++)   
    64. {   
    65. for(int j=0;j<G.vexnum;j++)   
    66. cout<<" "<<G.arcs[i][j].adj;   
    67. cout<<endl;   
    68. }   
    69. }   
    70. void main()   
    71. {   
    72. MGraph G;   
    73. CreatUDN(G);   
    74. dispMGraph(G);   
    75. }   
    76. // 邻接表 表示:   
    77. #include <iostream.h>   
    78. #include <stdlib.h>   
    79. #define MAX_VERTEX_NUM 20 //最大顶点数   
    80. #define MAX_EDGE_NUM 40 //最大边数   
    81. int visited[ MAX_VERTEX_NUM];   
    82. typedef int VertexType ; //顶点数据类型   
    83. typedef struct ArcNode   
    84. {   
    85. int adjvex;   
    86. int weight;   
    87. struct ArcNode *nextarc;   
    88. }ArcNode;   
    89. typedef struct VNode   
    90. {   
    91. VertexType data;   
    92. ArcNode *firstarc;   
    93. }VNode,AdjList[MAX_VERTEX_NUM];   
    94. typedef struct   
    95. {   
    96. AdjList vertices;   
    97. int vexnum,arcnum;   
    98. int kind;   
    99. }ALGraph;   
    100. void CreateDG(ALGraph &G)   
    101. {   
    102. int i,j,k;   
    103. ArcNode *p;   
    104. cout<<"创建一个图:"<<endl;   
    105. cout<<"顶点数:"; cin>>G.vexnum;cout<<endl;   
    106. cout<<"边数:"; cin>>G.arcnum; cout<<endl;   
    107. for(i=0;i<G.vexnum;i++)   
    108. {   
    109. G.vertices[i].data=i;   
    110. G.vertices[i].firstarc=NULL;   
    111. }   
    112. for(k=0;k<G.arcnum;k++)   
    113. {   
    114. cout<<"请输入第"<<k+1<<"条边:";   
    115. cin>>i>>j;   
    116. p=(ArcNode*)malloc(sizeof(ArcNode));   
    117. p->adjvex=j;   
    118. p->nextarc=G.vertices[i].firstarc;   
    119. G.vertices[i].firstarc=p;   
    120. }   
    121. }   
    122. void Disp(ALGraph G)   
    123. {   
    124. int i,j;   
    125. ArcNode *p;   
    126. cout<<"输出图为:"<<endl;   
    127. for(i=0;i<G.vexnum;i++)   
    128. {   
    129. p=G.vertices[i].firstarc;   
    130. j=0;   
    131. while(p!=NULL)   
    132. {   
    133. cout<<"("<<i<<","<<p->adjvex<<")";   
    134. p=p->nextarc;   
    135. j=1;   
    136. }   
    137. if(j==1)   
    138. cout<<endl;   
    139. }   
    140. }   
    141. void dfs(ALGraph G,int v) //深度优先遍历   
    142. {   
    143. ArcNode *p;   
    144. cout<<v<<" ";   
    145. visited[v]=1;   
    146. p=G.vertices[v].firstarc;   
    147. while(p!=NULL)   
    148. { if(!visited[p->adjvex])   
    149. dfs(G,p->adjvex);   
    150. p=p->nextarc;   
    151. }   
    152. return ;   
    153. }   
    154. void dfs1(ALGraph G)   
    155. {   
    156. int i;   
    157. for(i=0;i<G.vexnum;i++)   
    158. if(visited[i]==0)   
    159. dfs(G,i);   
    160. }   
    161. void main()   
    162. {   
    163. ALGraph G;   
    164. CreateDG(G);   
    165. int v;   
    166. Disp(G);   
    167. cout<<"输入顶点:";   
    168. cin>>v;   
    169. cout<<"深度优先序列:";   
    170. dfs1(G);   
    171. cout<<endl;   

    原文链接：http://blog.csdn.net/Sunboy_2050/article/details/5645828
  • 相关阅读:
    音乐分类/生成杂记
    音视频编码笔记
    《The challenge of realistic music generation: modelling raw audio at scale》论文阅读笔记
    使用基于Caffe的MobileNet分类踩坑备忘录
    四大轻量化模型对比（转）
    WaveNet: 原始音频生成模型
    《SONG FROM PI: A MUSICALLY PLAUSIBLE NETWORK FOR POP MUSIC GENERATION》论文笔记
    上采样 及 Sub-pixel Convolution （子像素卷积）
    python之sys._getframe() 用于查看函数被什么函数调用以及被第几行调用及被调用函数所在文件
    11
  • 原文地址：https://www.cnblogs.com/10jschen/p/2639631.html
Copyright © 2020-2023  润新知