1146 Topological Order (25 分)

1146 Topological Order (25 分)

 

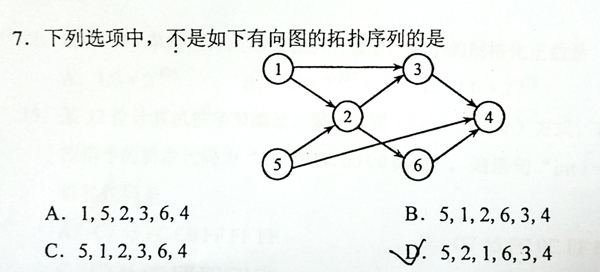
This is a problem given in the Graduate Entrance Exam in 2018: Which of the following is NOT a topological order obtained from the given directed graph? Now you are supposed to write a program to test each of the options.

Input Specification:

Each input file contains one test case. For each case, the first line gives two positive integers N (≤ 1,000), the number of vertices in the graph, and M (≤ 10,000), the number of directed edges. Then M lines follow, each gives the start and the end vertices of an edge. The vertices are numbered from 1 to N. After the graph, there is another positive integer K (≤100). Then K lines of query follow, each gives a permutation of all the vertices. All the numbers in a line are separated by a space.

Output Specification:

Print in a line all the indices of queries which correspond to "NOT a topological order". The indices start from zero. All the numbers are separated by a space, and there must no extra space at the beginning or the end of the line. It is graranteed that there is at least one answer.

Sample Input:

6 8
1 2
1 3
5 2
5 4
2 3
2 6
3 4
6 4
5
1 5 2 3 6 4
5 1 2 6 3 4
5 1 2 3 6 4
5 2 1 6 3 4
1 2 3 4 5 6

Sample Output:

3 4

拓扑排序的板子题，只要判断该排序是否符合就行。

#include <bits/stdc++.h>

using namespace std;
int n,m,k;
vector<int> v[1005], vt;
int val[1005],vals[1005], vis[1005], an[1005];

int main(){
    cin >> n >> m;
    int x, y;
    for(int i = 0 ; i < m; ++i){
        cin >> x >> y;
        v[x].push_back(y);
        val[y]++;
    }
    cin >> k;
    for(int l = 0; l < k; l++){
        for(int i = 1; i <= n; i++){
            cin >>an[i];
            vals[i] = val[i];
        }
        memset(vis,0,sizeof(vis));
        int pos = 0;
        while(pos++ < n){
            bool flag = false;
            if(vis[an[pos]] == 0&&vals[an[pos]] == 0){
                flag = true;
                vis[an[pos]] = 1;
                for(int j = 0; j < v[an[pos]].size(); j++){
                    vals[v[an[pos]][j]]--;
                }
            }
            if(!flag){
                break;
            }
        }
        if(pos != n+1)
            vt.push_back(l);
    }
    for(int i = 0 ; i < vt.size(); i++)
        printf("%d%c", vt[i], i == vt.size()-1?'
':' ');

    return 0;
}