A graph G = (V, E) is a data structure where V is a finite set of vertices and E is a binary relation on V represented by a set of edges. Fig. 1 illustrates an example of a graph (or graphs).
Fig. 1
A free tree is a connnected, acyclic, undirected graph. A rooted tree is a free tree in which one of the vertices is distinguished from the others. A vertex of a rooted tree is called “node.”
Your task is to write a program which reports the following information for each node u of a given rooted tree T:
node ID of u
parent of u
depth of u
node type (root, internal node or leaf)
a list of chidlren of u
If the last edge on the path from the root r of a tree T to a node x is (p, x), then p is the parent of x, and x is a child of p. The root is the only node in T with no parent.
A node with no children is an external node or leaf. A nonleaf node is an internal node
The number of children of a node x in a rooted tree T is called the degree of x.
The length of the path from the root r to a node x is the depth of x in T.
Here, the given tree consists of n nodes and evey node has a unique ID from 0 to n-1.
Fig. 2 shows an example of rooted trees where ID of each node is indicated by a number in a circle (node). The example corresponds to the first sample input.
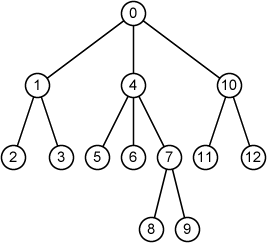
Fig. 2
Input
The first line of the input includes an integer n, the number of nodes of the tree.
In the next n lines, the information of each node u is given in the following format:
id k c1 c2 … ck
where id is the node ID of u, k is the degree of u, c1 … ck are node IDs of 1st, … kth child of u. If the node does not have a child, the k is 0.
Output
Print the information of each node in the following format ordered by IDs:
node id: parent = p , depth = d, type, [c1…ck]
p is ID of its parent. If the node does not have a parent, print -1.
d is depth of the node.
type is a type of nodes represented by a string (root, internal node or leaf). If the root can be considered as a leaf or an internal node, print root.
c1…ck is the list of children as a ordered tree.
Please follow the format presented in a sample output below.
Constraints
1 ≤ n ≤ 100000
Sample Input 1
13
0 3 1 4 10
1 2 2 3
2 0
3 0
4 3 5 6 7
5 0
6 0
7 2 8 9
8 0
9 0
10 2 11 12
11 0
12 0
Sample Output 1
node 0: parent = -1, depth = 0, root, [1, 4, 10]
node 1: parent = 0, depth = 1, internal node, [2, 3]
node 2: parent = 1, depth = 2, leaf, []
node 3: parent = 1, depth = 2, leaf, []
node 4: parent = 0, depth = 1, internal node, [5, 6, 7]
node 5: parent = 4, depth = 2, leaf, []
node 6: parent = 4, depth = 2, leaf, []
node 7: parent = 4, depth = 2, internal node, [8, 9]
node 8: parent = 7, depth = 3, leaf, []
node 9: parent = 7, depth = 3, leaf, []
node 10: parent = 0, depth = 1, internal node, [11, 12]
node 11: parent = 10, depth = 2, leaf, []
node 12: parent = 10, depth = 2, leaf, []
Sample Input 2
4
1 3 3 2 0
0 0
3 0
2 0
Sample Output 2
node 0: parent = 1, depth = 1, leaf, []
node 1: parent = -1, depth = 0, root, [3, 2, 0]
node 2: parent = 1, depth = 1, leaf, []
node 3: parent = 1, depth = 1, leaf, []
Note
You can use a left-child, right-sibling representation to implement a tree which has the following data:
the parent of u
the leftmost child of u
the immediate right sibling of u
Reference
Introduction to Algorithms, Thomas H. Cormen, Charles E. Leiserson, Ronald L. Rivest, and Clifford Stein. The MIT Press.
Code
/*
^....0
^ .1 ^1^
.. 01
1.^ 1.0
^ 1 ^ ^0.1
1 ^ ^..^
0. ^ 0^
.0 1 .^
.1 ^0 .........001^
.1 1. .111100....01^
00 11^ ^1. .1^
1.^ ^0 0^
.^ ^0..1
.1 1..^
1 .0 ^ ^
00. ^^0.^
^ 0 ^^110.^
0 0 ^ ^^^10.01
^^ 10 1 1 ^^^1110.1
01 10 1.1 ^^^1111110
010 01 ^^ ^^^1111^1.^ ^^^
10 10^ 0^ 1 ^^111^^^0.1^ 1....^
11 0 ^^11^^^ 0.. ....1^ ^ ^
1. 0^ ^11^^^ ^ 1 111^ ^ 0.
10 00 11 ^^^^^ 1 0 1.
0^ ^0 ^0 ^^^^ 0 0.
0^ 1.0 .^ ^^^^ 1 1 .0
^.^ ^^ 0^ ^1 ^^^^ 0. ^.1
1 ^ 11 1. ^^^ ^ ^ ..^
^..^ ^1 ^.^ ^^^ .0 ^.0
0..^ ^0 01 ^^^ .. 0..^
1 .. .1 ^.^ ^^^ 1 ^ ^0001
^ 1. 00 0. ^^^ ^.0 ^.1
. 0^. ^.^ ^.^ ^^^ ..0.0
1 .^^. .^ 1001 ^^ ^^^ . 1^
. ^ ^. 11 0. 1 ^ ^^ 0.
0 ^. 0 ^0 1 ^^^ 0.
0.^ 1. 0^ 0 .1 ^^^ ..
.1 1. 00 . .1 ^^^ ..
1 1. ^. 0 .^ ^^ ..
0. 1. .^ . 0 .
.1 1. 01 . . ^ 0
^.^ 00 ^0 1. ^ 1 1
.0 00 . ^^^^^^ .
.^ 00 01 ..
1. 00 10 1 ^
^.1 00 ^. ^^^ .1
.. 00 .1 1..01 ..
1.1 00 1. ..^ 10
^ 1^ 00 ^.1 0 1 1
.1 00 00 ^ 1 ^
. 00 ^.^ 10^ ^^
1.1 00 00 10^
..^ 1. ^. 1.
0 1 ^. 00 00 .^
^ ^. ^ 1 00 ^0000^ ^ 01
1 0 ^. 00.0^ ^00000 1.00.1 11
. 1 0 1^^0.01 ^^^ 01
.^ ^ 1 1^^ ^.^
1 1 0.
.. 1 ^
1 1
^ ^ .0
1 ^ 1
.. 1.1 ^0.0
^ 0 1..01^^100000..0^
1 1 ^ 1 ^^1111^ ^^
0 ^ ^ 1 1000^
.1 ^.^ . 00
.. 1.1 0. 0
1. . 1. .^
1. 1 1. ^0
^ . ^.1 00 01
^.0 001. .^
*/
// Virtual_Judge —— Rooted Trees Aizu - ALDS1_7_A .cpp created by VB_KoKing on 2019-05-08:08.
/* Procedural objectives:
Variables required by the program:
Procedural thinking:
Functions required by the program:
Determination algorithm:
Determining data structure:
*/
/* My dear Max said:
"I like you,
So the first bunch of sunshine I saw in the morning is you,
The first gentle breeze that passed through my ear is you,
The first star I see is also you.
The world I see is all your shadow."
FIGHTING FOR OUR FUTURE!!!
*/
#include <iostream>
#define MAX 100007
#define NIL -1
using namespace std;
struct Node{int parent,left,right;};
Node T[MAX];
int n,D[MAX];
void print(int u)
{
cout<<"node "<<u<<": ";
cout<<"parent = "<<T[u].parent<<", ";
cout<<"depth = "<<D[u]<<", ";
if (T[u].parent==NIL) cout<<"root, ";
else if (T[u].left==NIL) cout<<"leaf, ";
else cout<<"internal node, ";
cout<<'[';
for (int i = 0, c=T[u].left; c!=NIL ; i++, c=T[c].right) {
if (i) cout<<", ";
cout<<c;
}
cout<<']'<<endl;
}
//递归求深度
int rec(int u,int p)
{
D[u]=p;
if (T[u].right!=NIL) rec(T[u].right,p); //右侧兄弟设置为相同深度
if (T[u].left!=NIL) rec(T[u].left,p+1); //最左侧子结点的深度设置为自己的深度+1
}
int main()
{
cin>>n;
for (int i = 0; i < n; i++)
T[i].parent=T[i].left=T[i].right=NIL;
for (int i = 0; i < n; i++) {
int c,d,v,l;
cin>>v>>d;
for (int j = 0; j < d; j++) {
cin>>c;
if (j) T[l].right=c;
else T[v].left=c;
l=c;
T[c].parent=v;
}
}
int r; //根节点的编号
for (int i = 0; i < n; i++) {
if (T[i].parent==NIL) r=i;
}
rec(r,0);
for (int i = 0; i < n; i++) {
print(i);
}
return 0;
}